📌  相关文章
📜  对数微分——连续性和微分性

📅  最后修改于: 2022-05-13 01:54:13.910000             🧑  作者: Mango

对数微分——连续性和微分性

连续性一词的意思是本质上是连续的。水的流动是连续的，现实生活中的时间是连续的，还有更多的例子表明了现实生活中的连续性。在数学中，连续函数是在图形上绘制时不会显示任何中断并且本质上是连续的函数。函数的可微性只有且仅当它本质上是连续的时才可能。对数微分是一个单独的主题，因为它具有多个属性并且可以更好地理解 Log。

连续性和可区分性

函数的连续性表明了两件事，函数的属性和函数在任意点的函数值。如果函数的值在 x=a-、x=a+ 和 x=a 处保持不变，则称函数在 x = a 处是连续的。更正式地，它可以写成，

一个函数在闭区间 [a, b] 内连续，如果

  • f 在 (a, b) 中是连续的
  • 关注Lim_{x=a+}f(x)=f(a)
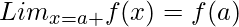
  • 关注Lim_{x=b-}f(x)=f(b)

可微性意味着函数是可微的，如果说函数在 x=a 处可微，则意味着 f'(a) [函数的导数] 存在于该域中的每个点。

可微性⇢ f'(a)=\frac{f(a+h)-f(a)}{h}

对数微分

对数微分在物理学和数学的许多地方都有其用途，无论是解决错误还是解决非常复杂的函数，对数微分都比简单微分更受欢迎。该方法具有应用时简化计算的特性和规则，应用商规则并且产品在非常复杂的函数中不实用，此时，进行对数微分是更好的选择。

求解对数微分的方法

  • 首先，取给定方程两边的自然对数。
  • 应用 log 的不同属性来破坏函数并使其更容易解决。
  • 区分应用规则的函数，如链式规则。
  • 将 RHS 与函数本身相乘，因为它在 LHS 的分母中。

logₐx 的导数（对于任何正基数 a≠1）

已知logx的微分是1/x，但这是自然对数的微分（即基数e），是否有可能有不同的基数，它们的微分也是可能的吗？是的。借助 log 的 2 个简单属性，可以推导出它。

让我们找出 Log a x 的导数（其中 a 是任何正整数 a≠1），

d/dx(lnx)= 1/x

当基数改变时，它们可以写成，

log_pq=\frac{logq}{logp}

因此，以上面给出的形式写入 log a x 然后对其进行微分将给出，

log_ax=\frac{logx}{loga}

区分双方，

d/dx[log_ax]=d/dx[\frac{logx}{loga}]\\=\frac{1}{loga}d/dx[logx]\\=\frac{1}{xloga}

示例 1：求 log 9 x 的微分

回答：

示例 2：微分 -5log 6 x

回答：

示例 3：微分 log 4 (x 2 +x)

解决方案：

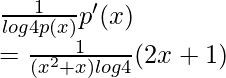
上面的例子都与 log a x 的微分有关，也与合数的微分有关。

Log的一些基本属性

Property/RuleFormula
Productln(xy)= ln(x)+ ln(y)
Log reciprocalln(1/x)= -ln(x)
Log of 1ln(1)= 0
Log of eln(e)= 1
Log of powerln(x)y= yln(x)
Quotientln(x/y)= ln(x)-ln(y)

现在让我们看看其他一些基于对数属性的例子。

示例问题

问题1：区分， f(x)= log_2{(1-3x^3)}

解决方案：

问题2：微分，h(x)= 5ln(x)

解决方案：

问题 3：微分，y= ln(4+ 7x 5 )

解决方案：

问题4：微分，y = cosx × cos3x × cos5x

解决方案：

问题5：一个数的Log是什么意思？

回答：

问题6：区分， f(x)= log(x)^{sinx}

回答：

问题 7：分步说明求解对数微分。

回答：

问题8：区分， y=\frac{x+5}{x^3+3}

回答：

问题9：区分， y= \frac{(x+3)(x^5-2)}{(x+10)(x^2+1)}

回答：