测量学是数学的一个分支,致力于研究不同的几何形状,面积和体积。它使用几何计算和代数方程来计算对象各个方面的度量,例如表面积,体积等。
体积是3D对象内部可以填充的空间量的度量。它可以测量任何固体物体,例如立方体,正方形,圆柱体,球体,金字塔等。物体的体积通常使用立方单位进行测量。通过找出物体的体积,还可以帮助我们找到填充物体所需的数量
体积的SI单位为m 3 。其他单位是毫升/升。
1m3 = 1 Litre
计算3D对象的体积
到现在为止,您必须已经了解了体积的含义,让我们开始寻找各种3D几何图形的体积,例如立方体,长方体和圆柱体。
求长方体的体积
长方体是具有六个矩形面的三维结构。长方体的体积等于长方体的长度,宽度和高度的乘积。在矩形长方体中,所有角度均成直角,并且长方体的相对面相等。
令l为长度, b为宽度, h为长方体的高度。

Example 1: Calculate the volume of the cuboid where length, breadth, and height are 12, 8, and 4 meters respectively.
Solution: Given, Length=12m
Breadth = 8m
Height = 4m
Applying the Formula,
Volume of cuboid = length x breadth x height
Volume of cuboid = 12 x 8 x 4 = 384m^3
Example 2: Calculate the breadth of the cuboid whose volume is given as 350-meter cube. And length and height are 7 and 5 meters respectively.
Solution: Given Volume of cuboid = length x breadth x height
350 = 7 x breadth x 5
350/35 = breadth
10m = breadth
立方体积
首先出现的问题是什么是立方体?因此,立方体是具有相等的宽度,高度和长度尺寸的三维形状。基本上,将长,宽,高相等的长方体称为立方体。
既然我们知道 长方体的体积=长度x宽度x高度,立方体是长方体的特例,其中所有长度,宽度和高度均相等。假设长度=宽度=高度= a
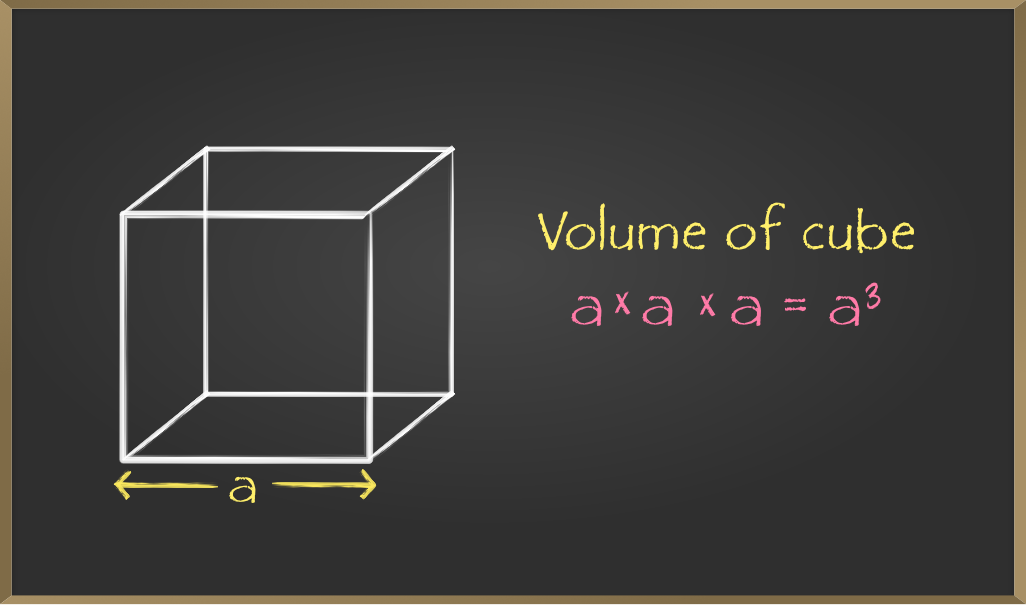
Example 1: Find the volume of a cube with sides of length 10 cm.
Solution: We know, V = (a ^ 3)
V = (10^3 cm)
So, Volume of cube = 300 centimeter cube
Example 2: Find the length of the sides of the cube whose volume is 343centimeter cube
Solution : Volume = side x side x side
343= (side)^3
7 cm = side
气缸容积
圆柱体是三维实体,包含两个通过曲面连接的平行基座。底座通常是圆形的。基部之间的垂直距离表示为圆柱体的高度“ h ”,而“ r ”为圆柱体的半径。
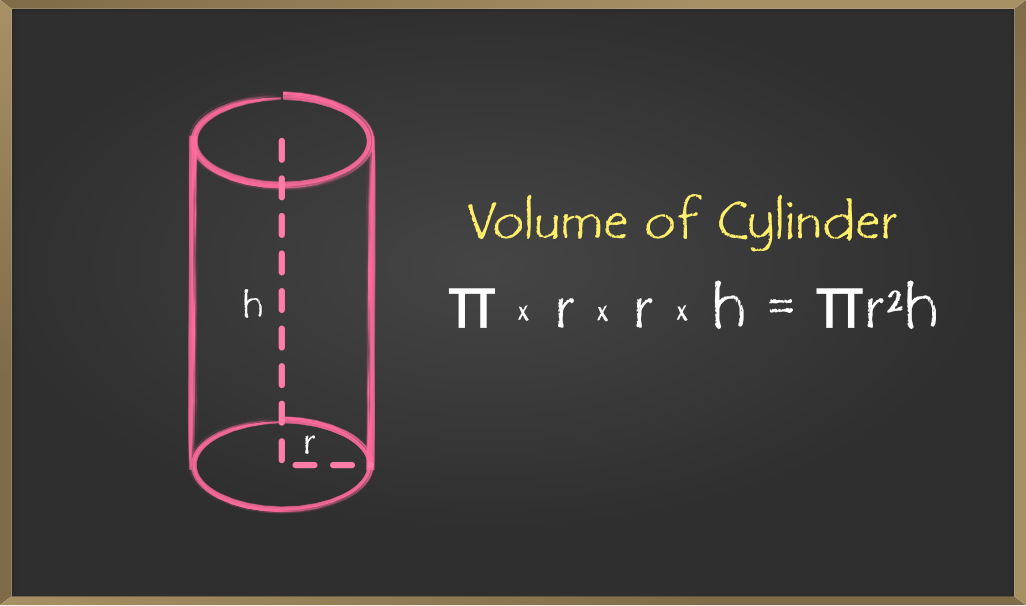
Example 1: Calculate the volume of the cylindrical container having a radius of 4 cm and height of 35 cm.
(Take pi = 22/7)
Solution: Radius = 4cm
Height = 35cm
Putting the values in the formulae,
Volume of cylinder = π x 4 x 4 x 35
= π x 560 (pi = 22/7)
= 1760 cm^3
Example 2: Calculate the radius of the cylinder whose height is 21 cm and volume of the cylinder is 1100 centimeter cube?(Take pi = 22/7)
Solution: Volume of cylinder = πr2h cubic units
1100 = 22/7 x r x r x 7
r = 7.07cm