📌  相关文章
📜  求圆柱体的体积和表面积

📅  最后修改于: 2022-05-13 01:54:12.622000             🧑  作者: Mango

求圆柱体的体积和表面积

在几何学中，圆柱体是一个 3D 立体图形，包含两个平行的圆形底面，这些底面由曲面连接，位于距圆柱体中心特定距离处。例如，卫生纸卷、塑料冷饮罐就是圆柱体的例子。圆柱体具有两个主要特性，即表面积和体积。圆柱体一词源自拉丁文（Cylindrus）词，意思是“滚”、“滚”和“tumblr”。

气缸的定义

圆柱体是一个三维实体，它包含两个平行的底面，这些底面通过一个曲面固定在一起，并保持固定的距离。圆柱体的底部通常是圆形的，类似于圆形。底座由一条线段连接在一起，该线段称为轴。这条线段到圆柱外表面的距离称为半径。这可以用“r”表示。圆柱体底面之间的垂直距离称为圆柱体的高度，用“h”表示。

气缸的零件

圆柱体被认为是由 2 个圆 + 1 个矩形组成。下图描绘了圆柱体的形成：

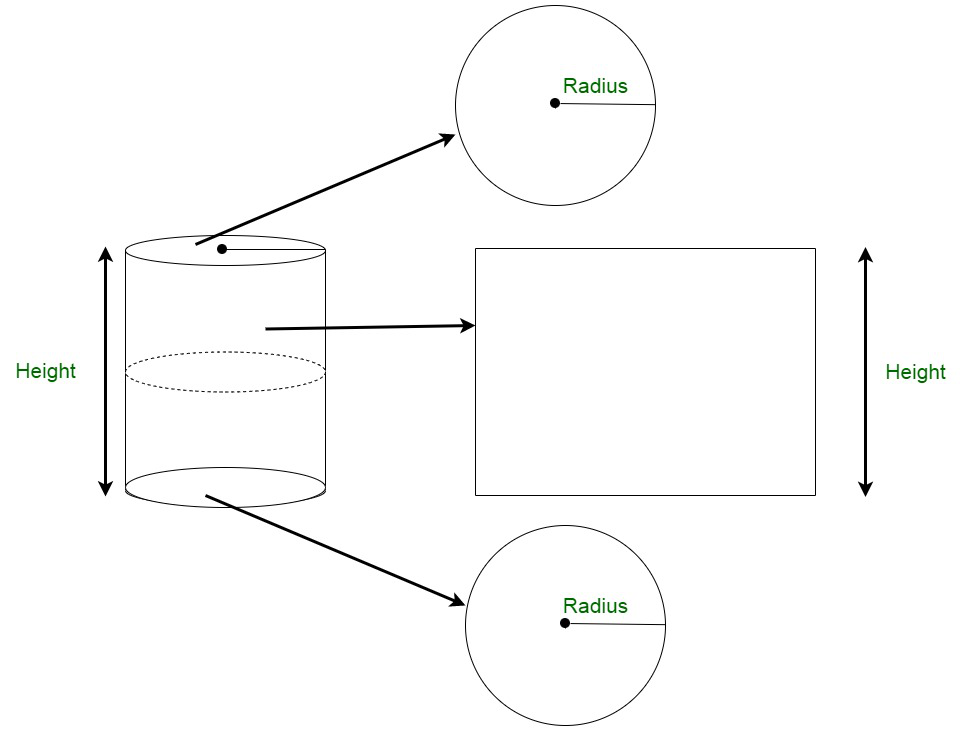
气缸类型

几何由四种类型的圆柱体组成，即，

  • 椭圆圆柱体：形成椭圆的圆柱体称为椭圆圆柱体。
  • 直圆柱：直圆柱包含两条平行线的轴线，两条平行线垂直于底座的中心。
  • 斜圆柱体：斜圆柱体是侧面靠在底座上的圆柱体。在斜圆柱体中，边不垂直于底面的中心。比萨斜塔是斜圆柱的真实例子。
  • 正圆形空心圆柱体或圆柱壳：也称为圆柱壳，包含两个以一侧为界的直圆柱体。轴的点是交点的公共点，并且垂直于中心底。由于圆柱体内部有一些空间，因此从内部看是中空的。

圆柱体公式

圆柱体与三个公式相关联，发现其在面积和体积方面的应用：

  • 横向表面积或曲面面积
  • 总表面积
  • 圆柱体积

圆柱体的侧表面积或曲面面积

曲面区域也称为侧表面区域。由圆柱的曲面形成的区域，即两个平行的圆形底座之间占据的空间称为CSA。 CSA 的公式如下：

圆柱体总表面积

因此，为了找出圆柱体的总表面积，我们计算曲面面积和两个圆的面积。

圆柱体的总表面积定义为它占据的总面积。圆柱体由两个圆和一个弯曲的薄片组成。圆柱的总表面积可以通过曲面面积和两个圆的面积的组合来计算。

曲面面积（CSA）=圆的周长×高度

CSA = 2r × h

圆的面积 = πr 2

总表面积 (TSA) = 曲面面积 + 2（圆的面积）

我们知道，

曲面面积 = 2πrh

圆的面积 = πr 2

气缸体积

圆柱体的体积被称为它所占据的空间的密度或数量。

我们有，

圆柱的体积=圆的面积×高

因为，我们有一个圆的面积 = πr 2

示例问题

问题1：计算圆柱体的总表面积，半径为5cm，高度为10cm？

解决方案：

问题2：一个圆柱形的水容器，高7cm，直径10cm，体积是多少？

解决方案：

问题 3：Alex 想购买一个半径等于 5 英寸的圆柱形罐头。罐子里有 1 加仑的油。求圆柱体的高度。

解决方案：

问题 4. 水箱的半径为 40 英寸，高度为 150 英寸。找到该区域。

解决方案：

问题 5. 求半径为 5 个单位，高为 8 个单位的圆柱体的体积？

解决方案：