四边形在生活中无处不在,每个正方形,具有四个边的任何形状都是四边形。我们知道,三个非共线的点组成一个三角形。类似地,四个非共线点采用的形状称为四边形。它具有四个边,四个角度和四个顶点。
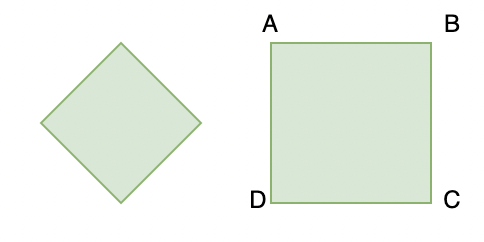
上面的两个图都是四边形的示例。 ABCD是四边形的。 AB,BC,CD和DA是四边形的四个边。 A,B,C和D是四个顶点,而∠A,∠B,∠C和∠D 是这个四边形的角度。
一些重要术语
让我们看一些与四边形有关的术语和约定。
对边:如果没有公共顶点,则四边形的两个边称为对边。
For example: In the figure given above look at the quad ABCD. Here, AB and CD are opposite sides. Similarly, AD and BC are opposite sides.
对角:如果没有共同的手臂,则四边形的两个角是相反的。
For example: In the figure ABCD again, angle A and angle C don’t have any common arm. Thus, they can be considered as opposite angles. Similarly, angles B and D are also opposite angles.
相邻侧面:如果侧面具有相同的顶点,则将其称为相邻的两个侧面。
For example: AB and AD have common vertex “A”. So, they are called adjacent sides. Similarly, AB, BC; BC, CD and AD, DC are adjacent sides.
相邻角:如果两个角具有相同的手臂,则称为相邻角。
For example: ∠A, ∠B are adjacent angles.
问题:列出下面给出的四边形中的一对相对的边和相邻的角。

回答:
Pair of opposite sides are the sides which don’t have any common vertices.
So, in this case (AB, CD) and (AC, BD) are two pairs of opposite sides.
Similarly, going by the definition given above. Pair of adjacent sides are,
(AC, AB); (AB, BD); (BD, DC); (CD, AC)
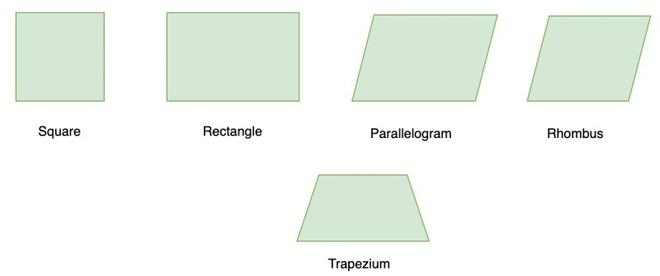
四边形的类型
四边形可以分为五种类型:
- 平行四边形:是四边形,其相对的边彼此平行且全等。相对的角度也相等。
- 矩形:是一个四边形,其相对的边相等,并且所有角度都为直角(90°)。
- 正方形:是一个四边形,其所有边均等长,并且所有角度都为直角(90°)。
- 菱形:这是一个平行四边形,其所有边均等长。
- 梯形:具有一对平行的侧面。其侧面的长度可以相等或可以不相等。
角度和属性
此属性表明四边形的所有角度之和为360°。让我们证明这一点。
Theorem: The sum of all the four angles of a quadrilateral is 360°.
Proof:
Let ABCD be a quadrilateral.
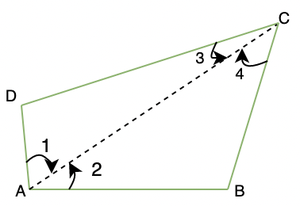
Join AC.
Now notice,
∠1 + ∠2 = ∠A
∠3 + ∠4 = ∠C
Therefore, from triangle ABC
∠4 + ∠2 + ∠B = 180o
Similarly, from triangle ADC
∠3 + ∠1 + ∠D = 180o
Adding these two equations,
∠4 + ∠2 + ∠B + ∠3 + ∠1 + ∠D = 360o
⇒ (∠1 + ∠2) + (∠3+ ∠4) + ∠B + ∠D = 360o
⇒ ∠A + ∠C + ∠B + ∠D = 360o
Thus, this proves that sum of all interior angles of a quadrilateral is 360°.
样本问题
问题1:四边形的角度是60°,90°,90°。找到第四个剩余角度。
解决方案:
We know from the angle sum property that the sum of the angles of a quadrilateral are 360o.
Let the fourth angle be denoted by “x”.
So,
60° + 90°+ 90° + x = 360°
⇒ 180° + 60° + x = 360°
⇒ 240° + x = 360°
⇒ x = 120°
问题2:四边形的角度分别为(3x) ° ,(3x + 30) ° ,(6x + 60) ° ,90 ° 。求出所有四边形角度的值。
解决方案:
We know, sum of all the angles of quadrilateral are 360°.
3x + (3x + 30) + (6x + 60) + 90 = 360
⇒ (3x + 3x + 6x) + (30 + 60 + 90) = 360
⇒ (12x) + (180) = 360
⇒ 12x = 360 – 180
⇒ 12x = 180
⇒ x = 15°
Thus, the angles are 45°, 75°, 150° and 90°
问题3:如果四边形的角度比例为1:2:3:4,请问该四边形的最大角度值是多少?
解决方案:
Since the sum of all 4 angles of a quadrilateral is 360°, we can equate the values (by multiplying with a constant) of these ratios to 360°
Suppose the constant that is getting multiplied is ‘x’
We can write, x+ 2x+ 3x+ 4x = 360
10x = 360
x = 36°
Therefore, the largest angle will be 4x = 4×36 = 144°
问题4:在下面的梯形中,∠A= 100°,∠C= 80°,求出其余角度。
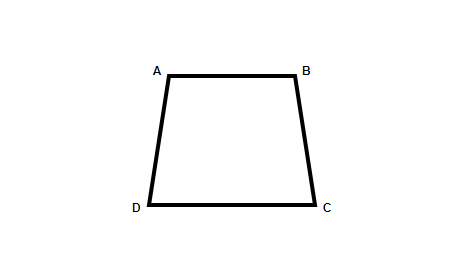
解决方案:
We already know, In a Trapezium, two opposite sides are parallel to each other, here, AB is parallel to CD
The Interior angles formed by two parallel lines have a sum of 180°(Property of parallel lines)
Therefore, we can write, ∠A + ∠D = 180°
100° + ∠D = 180°
∠D = 80°
Similarly, ∠B+ ∠C = 180°
∠B + 80° = 180°
∠B = 100°
问题5:在下图中,四边形的内角为
∠ABC= 50°,∠BAD= 20°,∠BCD= 10°
求出外倾角∠ADC的值?

解决方案:
In a quadrilateral, the sum of all the interior angles is 360°,
∠ABC + ∠BAD + ∠BCD + ∠ADC = 360°
50° + 20° + 10° + ∠ADC = 360°
∠ADC = 280°
The angle that came out is the interior angle, the sum of interior angle and the exterior angle will be 360,
Exterior angle ∠ADC = 360 – 280 = 80°
问题6:在给定的平行四边形ABCD中,内角值为60 ° 。查找所有其他角度的值。

解决方案:
The value of ∠D is given to be 60°. We need to find other angles.
We know that sum of adjacent angles in a parallelogram is 180°. So let the value of ∠A be x.
x + 60° =180°
x = 120°
∠A = 120°
We also know that opposite angles in a parallelogram are equal.
So,
∠A = ∠C and ∠D = ∠B
So, ∠A = 120°, ∠B = 60°, ∠C = 120° and ∠D = 60°
问题7:在给定的四边形中,∠A= 2x ° ,∠B= x ° ,∠C= 90 °和andD = 3x °。 找到最大角度的值。
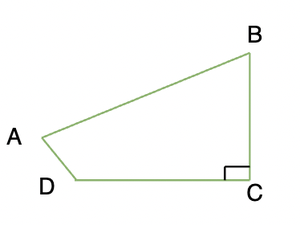
解决方案:
We know that by the angle sum property, sum of all the interior angles of quadrilateral is 360o
So, ∠A + ∠B + ∠C + ∠D = 360°
Given that ∠C = 90°
Let’s plug in the rest of the values given,
2x + x + 90 + 3x = 360
⇒ 6x = 360 – 90
⇒ 6x = 270
⇒ x = 45°
So, the largest angle is ∠D = 3x = 3(45) = 135°