两个立方体的体积比为 1:27，然后求它们的表面积比

立方体是由六个正方形面、小平面或边组成的三维图形，每个顶点有三个交汇点。有正方形的片材堆叠在一起，其特征是三个维度，即长、宽和高。所有这些尺寸的长度都是相等的。立方体由 12 条边和 6 个面组成。

立方体的面积

立方体的面积被定义为包含在其中的空间。形成立方体面的任何正方形片的面积被定义为任何两个维度的乘积，例如长度和宽度。让我们假设 A 是立方体的面积。它以平方单位定义。

现在，

A = 长 × 宽

因为，立方体的所有边都是相等的，

A = a × a

A = ²

立方体的体积

立方体的体积定义为图形中包含的物质。它是立方体内所含物质的总量。立方体的体积定义为立方体所有尺寸的乘积，使用它的长度、宽度和高度来定义。立方体的体积以立方单位定义。

现在，由于立方体的所有边都是相等的，

体积=长×宽×高

让我们假设 V 是体积，a 是立方体的同一侧。

V = a × a × a

V = 一个³

两个立方体的体积比为 1:27，然后求它们的表面积比

解决方案：

Let us assume a₁ and a₂ to be the side of cube1 and cube2 respectively. 

Now, 

Let the volume of cube1 be V₁ and cube2 be V₂ .

Upon equating, 

$\frac{V_1}{V_2} = \frac{1}{27}$

On solving, 

$\frac{(a_1)^3}{(a_2)^3} = \frac{1}{27} \\ \frac{(a_1)^3}{(a_2)^3} = \frac{1 * 1 * 1}{3 * 3 * 3}\\$

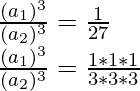
On taking the cube root, we get, 

$\frac{a_1}{a_2} = \frac{1}{3}$

Let us assume the area A₁ to be of cube1 and A₂ of cube2. 

On squaring both sides, we get,

$\frac{(a_1)^2}{(a_2)^2} = \frac{1}{3^2} \\ \frac{(a_1)^2}{(a_2)^2} = \frac{1}{9}$

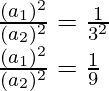
Therefore, the ratio of areas of both the cubes is 1 : 9. 

类似问题

问题 1：两个立方体的体积比为 1:64。一个立方体的边与另一个立方体的边之比是？

解决方案：

We have, 

$\frac{V_1}{V_2} = \frac{1}{64} \\ \frac{a_1^3}{a_2^3} = \frac{1}{64} \\ On \space taking \space cuberoot \\ \frac{a_1}{a_2} = \frac{1}{4}$

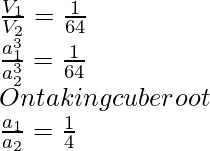
Therefore, 

The ratio of their sides is 1:4. 

问题2：根据上述问题求面积比。

解决方案：

$\frac{V_1}{V_2} = \frac{1}{64} \\ \frac{a_1^3}{a_2^3} = \frac{1}{64} \\ On \space taking \space cuberoot \\ \frac{a_1}{a_2} = \frac{1}{4} \\ On \space taking \space square \\ \frac{a_1^2}{a_2^2} = \frac{1^2}{4^2} \\ \frac{a_1^2}{a_2^2} = \frac{1}{16}$

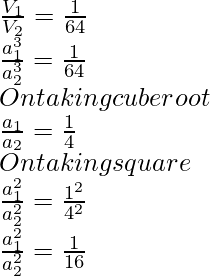
Therefore, the ratio of their areas is 1:16. 

问题 3：两个立方体的体积比为 n:n/8。一个立方体的边与另一个立方体的边之比为

解决方案：

On simplifying the equation, 

The volumes of the cubes are in the ratio 8n : n. 

On striking off, n from both sides, we obtain the final ratio as 8:1.

$\frac{V_1}{V_2} = \frac{8}{1} \\ \frac{a_1^3}{a_2^3} = \frac{8}{1} \\ On \space taking \space cuberoot \\ \frac{a_1}{a_2} = \frac{2}{1} \\ \frac{a_1}{a_2} = 2$

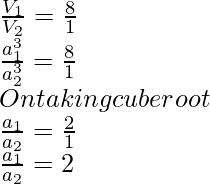
问题 4：计算边长为 n 的立方体的面积与体积之比。

解决方案：

Area of a cube = n²

Volume of a cube = n³ 

The ratio of area : volume is equivalent to, 

$\frac{Area}{Volume} = \frac{a^2}{a^3} \\ \frac{Area}{Volume} = \frac{1}{a}$

This implies that the volume is a times greater than area. 

问题 5：如果一个边长为 7 cm 的立方体的体积是 343 cm ³ 。计算它的面积。

解决方案：

Area is given by Volume/Side

= 343/7 cm²

= 49 cm²