速度 - 时间图
任何包含动能的东西都处于某种运动中,无论是一维、二维、旋转运动等。动能负责运动,众所周知,任何物体的运动都是由于某种原因而发生的。外力,物体中存在的惯性不允许它们自己移动或停止,因此,需要一些外力来为物体提供一些动能,而提供能量本身就表明物体具有一定的速度。运动中的物体可以有几种类型的速度,用图形来解释速度的特性更容易。
物体的速度
物体的速度可以定义为位移的变化率,也可以定义为根据给定参考系相对于时间的物体位置的变化。物体的速度是一个向量,也就是说。速度既有大小又有方向,这就是它与速度的不同之处,因为物体的速度不包含方向。
| SI unit | m/sec |
| symbol | v |
| dimension | LT-1 |
示例 1:Tinkesh 在 30 分钟内从他的办公室步行到他家,从办公室到家的位移为 2 公里。找出 Tinkesh 行走的恒定速度的值?
解决方案:
It is known that the velocity of any person/object is the rate of change of its displacement.
Therefore, Velocity= displacement/Time
Given, displacement = 2km = 2000 meters
Time = 30 minutes = 1800 seconds.
Velocity = 2000/1800
Velocity= 1.11m/sec
示例 2:两个物体以 20m/sec 和 40m/sec 的速度移动,以便到达距离两个物体起点 8000 米的同一目的地。找出两个对象所用的时间。
解决方案:
Time taken by the first object (T1)= displacement/Velocity
T1= 8000/20
T1= 400seconds
T1= 400/60= 20/3 minutes.
Time taken by the second object (T2) = 8000/40
T2= 200 seconds
T2= 200/60 minutes
T2= 10/3 minutes
速度-时间图
速度-时间图在学习任何物体的运动时非常重要,该图讲述了物体的加速度。根据不同类型的速度,例如,一个物体可能具有恒定的速度、增加的速度或减少的速度,可以很容易地确定该物体是在加速、减速还是根本不加速。
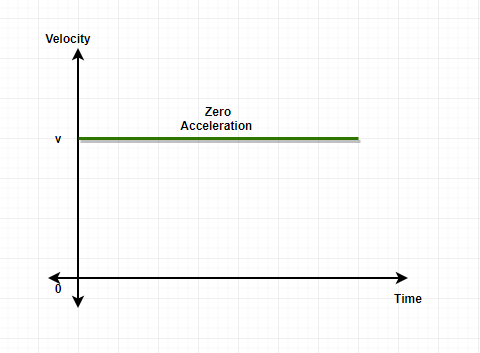
案例 1:等速(无加速)
当物体的运动速度相同时,初速度和终速度保持不变,物体会有一定的初速度,不能有零初速度,得到的速度-时间图的斜率是恒定的。恒定斜率表示物体的加速度没有增加或没有减少。因此,在这种情况下,物体的加速度为零。
在下图中,x 轴代表时间,y 轴代表速度。加速度为零,因此,运动方程在这里不适用,上面给出的简单公式用于找出物体的速度。
案例 2:增加速度(恒定加速度)
当物体的速度在整个运动过程中不断增加时,最终速度变得大于初始速度,在这种情况下,初始速度也可以为零,因为物体不断增加其速度。在这种情况下获得的速度-时间图的斜率本质上是增加的,增加的斜率表明物体处于恒定加速度。
图表上的 x 轴代表时间,y 轴代表速度。运动方程适用于找出运动中物体的参数,因为方程适用于经历恒定加速度的物体。

案例 3:增加速度变化率(增加加速度)
当速度的变化率在整个运动过程中不断增加时,最终速度将远大于初始速度,在这种情况下,初始速度也可以为零,因为物体的加速度每秒都在以一定的速度增加。在这种情况下,速度-时间图的斜率将采用曲线的形式,斜率中的曲线表明身体具有增加/减少的加速度。
图表上的 x 轴代表时间,y 轴代表速度。图中任意点的斜率将给出该点的加速度值。

Note: Just like increasing velocity-time graph, there can be decreasing velocity-time graph where the slope is decreasing since the velocity is decreasing in nature, and just like the increasing acceleration graph, there exist graph for decreasing acceleration as well with a negative slope curve since the object is undergoing the rate of change of deceleration.
示例问题
问题 1:画出一个物体的图形,该物体以 20m/sec 的速度开始,在 T=10 秒时最终速度变为 0m/sec。该物体在本质上正在减速。
回答:
The graph will have a negative slope since the object is deceleration in nature.
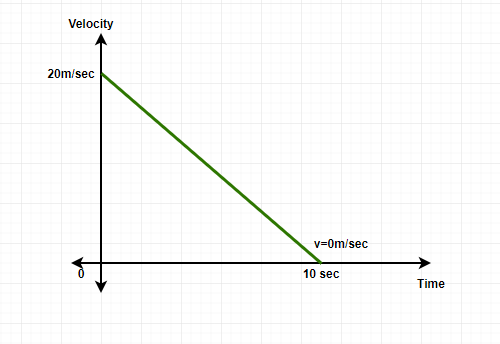
Velocity-time graph
问题 2:一个初速为 15m/sec 的物体正在减速并最终在 T=20 秒时停止,求该物体所覆盖的位移。

解决方案:
The displacement of the object can be found out by finding the area of the velocity-time graph.
The graph given forms a right angle triangle and the area of this right angle triangle will give the displacement covered by the object.
Area of the right angle triangle= Displacement of the object= 1/2× Base × Height
Displacement= 1/2 × 20× 15
Displacement = 150 meters.
问题 3:求物体的加速度,它本质上是恒定的,物体的速度曲线如下图所示。

回答:
The acceleration in the velocity-time graph is found out through the slope, the slope is the ratio of the value at y-axis and x-axis, solving this will easily give the value of acceleration of the object.
At time T= 8 sec, the value of the velocity of the object is 8 m/sec.
Hence, the slope of the graph at T= 10 sec will be,
Slope of the graph= Acceleration of the object = 8/10
Acceleration = 0.8 m/sec2
问题 4:Alakh 决定骑 10 分钟的短途自行车。他以 2 m/sec2 的恒定加速度骑行,在骑行结束时,Alakh 的自行车的速度是多少?
解决方案:
Applying first Equation of Motion to find out the velocity of Alakh’s bike,
v= u+ at
Here, the initial velocity (u)= 0 m/sec
Acceleration (a)= 2 m/sec2
Time = 10 minutes= 60 seconds
v= 0+ 60× 2
v= 120m/sec