杨氏双缝实验

光学是材料科学的一部分，专注于光的行为和特性，将其与问题的联系以及利用或识别它的仪器的开发结合起来。光学通常描述明显、明亮和红外光的传导。由于光是一种电磁波，不同类型的电磁辐射，例如 X 射线、微波和无线电波显示出比较特性。大多数光学奇迹都可以通过利用传统的电磁光描绘来表示。

无论如何，光的完整电磁描述通常很难实际应用。脚踏实地的光学通常是利用工作模型完成的。其中最广为人知的是数学光学，将光视为各种光束，当它们穿过或从表面反射时，它们会以直线和曲线移动。实际光学是一种更全面的光模型，它包含了波的影响，例如数学光学中无法表示的衍射和障碍。总的来说，基于光束的光模型首先发展起来，然后是光的波动模型。 19 世纪电磁假说的进展促使人们发现光波确实是电磁辐射。

杨氏双缝实验

Young’s double-slit experiment employs two coherent light sources separated by a modest distance, generally a few orders of magnitude larger than the wavelength of light. 

杨的双缝实验有助于理解光波理论。狭缝与屏幕或光电探测器相隔很大距离“D”。 Young 最初的双缝实验采用来自单一光源的衍射光，然后通过两个额外的狭缝传输作为相干光源。在今天的研究中，激光经常被用作相干光源。

每个源都可以被认为是一个相干光波源。波行进长度为 l1 和 l2，以在屏幕上距中心距离为“y”的任何位置产生 l 的路径差。在源处，该点大致对角 （由于距离 D 很大，源处对角的差异很小）。

杨氏双缝实验推导

考虑一个远离两个狭缝 s ₁和 s ₂的单色光源“S”。 S与s ₁和s ₂在同一平面上。因为 s ₁和 s ₂都是从 S 中提取的，所以它们充当了两个一致的来源。

光穿过这些狭缝并落在屏幕上，该屏幕位于距孔径 s ₁和 s ₂距离“D”处。两个狭缝之间的距离用字母“d”表示。

如果 s ₁打开而 s ₂关闭，则 s ₁对面的屏幕变暗，仅 s ₂对面的屏幕亮起。只有当狭缝s ₁和s ₂都打开时，才能形成干涉图案。

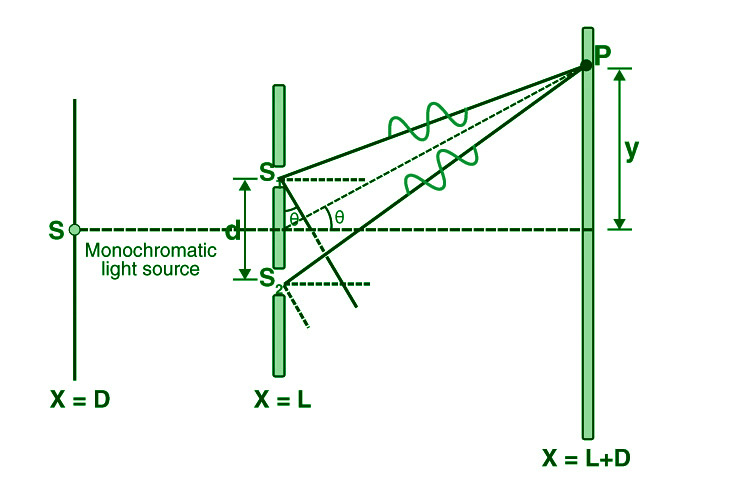
在切割分区 (d) 和屏幕距离 (D) 保持不变的点，为了到达 P，来自 s ₁和 s ₂的光波应该传播不同的距离。这表明来自 s ₁和 s ₂的两个光波在 Young 的双重切割测试中存在方式对比。

Approximations in Young’s twofold cut analysis- 

Guess 1: D > d 

Since D > d, the two light beams are thought to be equal.  

Guess 2: d/λ >> 1

Often, d is a small amount of a millimeter and λ is a negligible portion of a micrometer for noticeable light.  

在这些条件下 θ 很小，因此，我们可以利用估计，

sin θ = tan θ ≈ θ = λ/d

∴路径差，

Δz = λ/d

这是在屏幕上某一点相遇的两个波之间的路径差。正因为杨双切调查的这种路径差异，银幕上的焦点有的精彩，有的焦点平淡。

杨氏双缝实验中条纹的位置

• 亮边的位置

为了在 P 处形成最大强度或明亮的条纹

路径差， Δz = nλ (n = 0, ±1, ±2, ...)

IE

xd/D = nλ

要么

x = nλD/d

第 n 个亮条纹到中心的距离为

x _n = nλD/d

同理，第（ ⁿ -1）条亮条纹到中心的距离为

x _(n-1) = (n -1)λD/d

条纹宽度， β = x _n – x _(n-1) = nλD/d – (n -1)λD/d = λD/d

（n = 0、±1、±2、……）

• 黑边的位置

为了在 P 处形成最小强度或暗条纹，

路径差， Δz = (2n + 1) (λ/2) (n = 0, ±1, ±2, ...)

IE

x = (2n +1)λD/2d

第 n 个暗条纹到中心的距离为

x _n = (2n+1)λD/2d

同理，第（ ⁿ -1）条亮条纹到中心的距离为

x _(n-1) = (2(n-1) +1)λD/2d

条纹宽度， β = x _n – x _(n-1) = (2n + 1) λD/2d – (2(n -1) + 1)λD/2d = λD/d

（n = 0、±1、±2、……）

边缘宽度

The fringe width is the distance between two consecutive bright (or dark) fringes.

β = λD/d

当杨氏双缝实验设备浸入折射率为（μ）的液体中时，光的波长和条纹宽度均下降“倍”。

β ¹ = β/μ

当使用白光代替单色光时，屏幕上会出现彩色条纹，红色条纹大于紫色条纹。

干涉条纹的最大阶数

在屏幕上，第 n^阶最大值的位置是，

γ = nλD/d

其中 n=0, ±1, ±2, …

但是，因为会违反^第二个近似值，所以“n”值不能取无限高的值。即 θ 很小（或）y << D。

⇒ γ/D = nλ/d <<1

因此，可以使用上述干扰最大值公式n<< d/λ。当“n”的值等于 d/λ 的值时，路径差不再可以计算为 dγ/D。

因此对于最大值，路径差 = nλ

⇒ dsinθ = nλ

要么

​n = dsinθ/λ

和

n_最大值= d/λ

上面表示的是框函数或最大整数函数。类似地，干扰最小值的最高阶由下式给出，

​n _min =[d/λ+1/2]

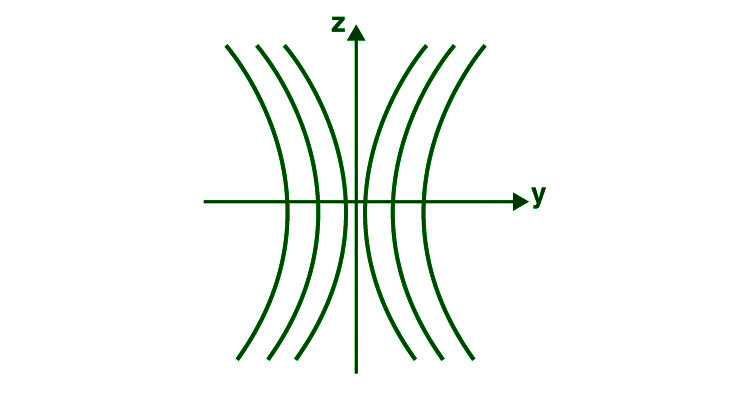
YDSE中干涉条纹的形状

两个狭缝之间的路径差异由 YDSE 图表示。

s ₂ p−s ₁ p≈dsinθ （常数）

前面的方程描述了一条双曲线，它有两个焦点，用字母 s ₁和 s ₂表示。

当我们在轴 s ₁ s ₂上旋转一条双曲线时，我们会在屏幕上获得一个干涉图案，它是一段双曲线。边缘是双曲线的，屏幕的直线中间部分位于 yz 平面中。

YDSE 中的条纹强度

两个相干源 s1 和 s2 在位置 p 处得到的强度由下式给出

I = I ₁ + I ₂ + 2 √(I ₁ . I ₂ ) cos φ

假设，I ₁ = I ₂ = I ₀ （因为，d<<

I = I ₀ + I ₀ + 2 √(I ₀ .I ₀ ) cos φ

= 2I ₀ + 2 (I ₀ ) cos φ

= 2I ₀ (1 + cos φ)

= 4I ₀ cos ² (φ/2)

建设性和破坏性干扰

路径差必须是波长的整数倍，才能发生相长干涉。结果，如果明亮的边缘位于“y”，

nλ = yd/D

其中 n = ±0,1,2,3…..

中心明亮边缘由第 0 边缘表示。类似地，在 Young 的双缝实验中，可以通过将路径差设置为：

Δl = (2n+1)λ/2

这简化为，

(2n+1)λ/2 = yd/D

要么

y = (2n+1)λD/2d

杨的双缝实验是科学的一个突破点，因为它毫无疑问地证明了光的行为就像波一样。后来，用电子重复了双缝实验，令所有人惊讶的是，产生的图案几乎与用光看到的相同。这将永远改变我们对物质和粒子的看法，要求我们相信物质就像光一样，就像波一样。

示例问题

问题1：杨氏双缝实验中，最小值与峰值的亮度比为9:25。计算两个狭缝宽度的比率。

回答：

I_max/I_min = (a₁+a₂)²/(a₁-a₂)²                

              = 9/25

Solving, a₁/a₂ = 4/1 

Therefore, Ratio of slit width , w₁/w₂ = a₁²/a₂²=16.

问题2：双缝实验中的缝间距为3mm，缝距屏幕2m。在屏幕上，可以观察到两种干涉图案，一种是由波长为 480 nm 的光引起的，另一种是由波长为 600 nm 的光引起的。屏幕上两个干涉图案的五阶亮边之间的距离是多少？

回答：

Separation is given by,

y= nλD/d

​where,

d = 3 mm = 3 × 10 ⁻³ m

D = 2 m

λ₁ = 480 nm = 480×10 ⁻⁹ m

λ₂ = 600 nm = 600×10 ⁻⁹ m

n₁​=n₂=5

So,

y_1​=  nλ₁D/d

y₁= 5×480×10 ⁻⁹×2 / 3×10 ⁻³

​y₁=1.6×10⁻³m

Also,  

y₂= nλ₂D /d

​y₂=  5×600×10⁻⁹×2 / 3×10⁻³

​y₂=2×10⁻³m

As , y₂ > y ₁

​y ₂ − y₁ ​= 2×10 ⁻³−1.6×10 ⁻³

            = 4×10 ⁻⁴ m

Therefore the separation on the screen between the fifth order bright fringes of the two interference patterns is 4×10⁻⁴ m.

问题3：杨氏实验中两条狭缝的宽度为1：25。干涉图样的峰与最小值的强度比，I _max /I _min为

回答：

Intensity is proportional to width of the slit. thus, I₁/I₂

= 1/25

or a₁/a₂ =  1/5

Imax/Imin = (a₁+a₂)²/(a₁-a₂)²

                 = (a₁+5a₁)²/(a₁-5a₁)²

                 = 36/16

                 = 9/4

问题4：舞台上振动的两个清醒点源S ₁和S ₂辐射出频率为λ的光。源之间的划分为 2λ。考虑一条穿过 S ₂并与线 S ₁ S ₂相对的线。与至少发生功率的 S ₂的最小分离是什么？

回答：

For minimum  intensity of light, path difference must be equal to path difference for dark e.g Δx=λ/2,3λ/2,5λ/2

Consider Δx=(2λ−λ/2)=3λ/2

Now, path difference=S₁P−S₂P

Δx=  √(4λ²+x²)−x

3λ/2+x=  √4λ ²+x ²

 Taking square on both sides,

9λ²/4+x ²+3λx=4λ ²+x ²

 3λx=4λ²−9λ²/4

x=7λ/12

The smallest distance from S₂ where a minimum of intensity occurs is 7λ/12

问题 5：玻璃镜片上镀有一层折射率为 1.50 的薄层。玻璃的折射率为 1.60。可以极强反射 546 nm 光（相长薄膜干涉）的最薄薄膜涂层是什么？

回答：

Light is entering in a medium of higher refractive index (n₂=1.60) from a medium of lower index (n₁=1.50), therefore net path difference in the reflected rays from the two interfaces will be equal to 2t (where t is thickness of thin film) , because when light goes from air to thin film path shift in reflected ray =λ’/2, when light goes from  thin film to glass path shift in reflected ray =λ’/2+2t

for constructive interference,    

2t=mλ’ or 2t=mλ/n₁

where λ’= wavelength in thin film  ,

λ=546nm(given) wavelength in vacuum ,

for thinnest coating , m=0(minimum) ,

therefore, t_min = λ/2n₁

                     ​= 546/(2×1.50) =182nm

问题 6：对于宽度为“a”的单个狭缝，波长为 e 的单色光的干涉图案的初始最小值出现在角度 λ/a 处。我们发现在相同角度 λ/a 处相隔距离“a”的两个薄狭缝的最大值。解释。

回答：

Width of the slit is a.

The path difference between two secondary wavelets is given by,

Nλ=asinθ

Since, θ is very small, sinθ=θ

So, for the first order diffraction n=1, the angle is  λ/a 

Now, we know that θ must be very small θ=0 (nearly) because of which the diffraction pattern is minimum.

Now for interference case, for two interfering waves of intensity l₁ and l₂

​we must have two slits separated by a distance.

We have the resultant intensity, l=l₁+l−2+ 2 √l₁l ₂cosθ

​Since, θ=0 (nearly) corresponding to angle λ/a, so cosθ=1 (nearly)

So,

l=l₁+l₂  +2 √ l₁l ₂cosθ

​l=l₁+l₂+2 √ l₁l₂cos0

​l=l₁+l₂+2 √ l₁l₂

​We see the resultant intensity is sum of the two intensities, so there is a maxima corresponding to the angle  λ/a

​This is why at the same angle λ/a we get a maximum for two narrow slits separated by a distance a.

问题 7：由两个相干光源产生的干涉图中的最高和最低强度的比率为 9:1。使用的灯的亮度是多少？

回答：

Imax /Imin = (a₁+a₂)² / (a₁-a₂)²

9/1 = (a₁+a₂)² / (a₁-a₂)²

a₁/a₂ = 2/1 

a₁² / a₂² = l₁ / l₂ 

             = 4/1