📌  相关文章
📜  河内DAA塔-JavaPoint

📅  最后修改于: 2020-12-10 03:56:26             🧑  作者: Mango

河内塔

1.这是一个经典问题，您尝试仅使用三个销将所有磁盘从一个销移动到另一个销。

2.最初，所有磁盘都堆叠在一起，较大的磁盘位于较小的磁盘下面。

3.尝试重新放置所有磁盘时，可以将磁盘移动到三个销钉中的任何一个，但是不能将较大的磁盘放在较小的磁盘上，并且一次只能转移一个磁盘。

通过分而治之算法可以轻松解决此问题

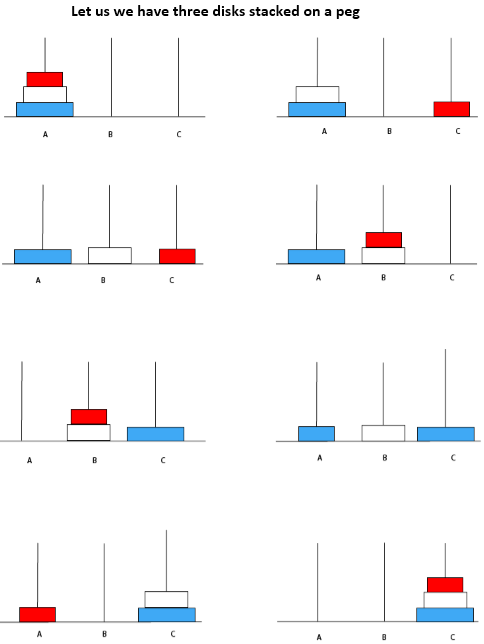
在上述第7步中，将钉销A的所有磁盘都转移到C并满足以下条件：

  • 一次只能移动一个磁盘。
  • 较小的磁盘可以放在较大的磁盘上。

令T(n)为将n个磁盘从钉A移到钉C所需的总时间

  • 将n-1个磁盘从第一个钉移到第二个钉。这可以在T(n-1)个步骤中完成。
  • 将较大的磁盘从第一钉移到第三钉将需要第一步。
  • 将n-1个磁盘从第二个桩递归移动到第三个桩将再次需要T(n-1)步骤。

因此，总耗时T(n)= T(n-1)+1+ T(n-1)

河内塔的关系公式为：

我们得到

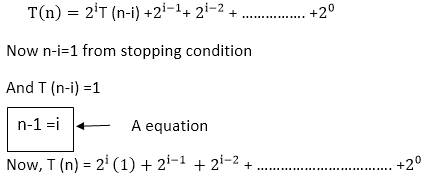
它是具有公共比率，r = 2的几何级数级数
第一项，a = 1(2 0 )

当我们必须将n个磁盘从一个钉移到另一个钉时，B方程是河内技术塔所需的复杂性。

T(3)= 2 3-1
= 8-1 = 7安

[在概念上，我们已经证明了将由通用方程式证明的7个步骤]

河内塔计划：

#include
void towers(int, char, char, char);
 int main()
 {
       int num;
       printf ("Enter the number of disks : ");
        scanf ("%d", &num);
      printf ("The sequence of moves involved in the Tower of Hanoi are :\n");
      towers (num, 'A', 'C', 'B');
      return 0;
 
}
     void towers( int num, char from peg, char topeg, char auxpeg)
 {
           if (num == 1)
 {
       printf ("\n Move disk 1 from peg %c to peg %c", from peg, topeg);
           return;
 }
   Towers (num - 1, from peg, auxpeg, topeg);
    Printf ("\n Move disk %d from peg %c to peg %c", num, from peg, topeg);
   Towers (num - 1, auxpeg, topeg, from peg);
 }

输出：

Enter the number of disks: 3
The sequence of moves involved in the Tower of Hanoi is
Move disk 1 from peg A to peg C
Move disk 2 from peg A to peg B
Move disk 1 from peg C to peg B
Move disk 3 from peg A to peg C
Move disk 1 from peg B to peg A
Move disk 2 from peg B to peg C
Move disk 1 from peg A to peg