简化 8/5x ^-2/3

代数是数学的一个分支，它通过为未知量分配各种字母、符号或字母（称为变量）来处理未知量。它们之所以被称为是因为它们在一段时间内会根据给定的任何情况而发生变化，但是始终需要表示这些变化的参数。代数中最常用的变量通常是英文字母，特别是 x、y、z。

代数表达式

简单来说，借助代数形成的表达式称为代数表达式。在数学中，通常使用数字来表示数量。在某些情况下，虽然数字可以用字母或字母或其他符号表示，但不提及它们的实际数量。此外，这些单独的量可以加、减、升为指数、激进化、相乘，甚至除以形成与手头主题相关的某种表达。

• 5b + 69 is an algebraic expression with b as the variable, 5 as the coefficient, 69 as the constant term and addition as the arithmetic operator.
• 420p² – 6900 is an algebraic expression with p as the variable, 420 as the coefficient, 6900 as the constant term and subtraction and exponent as the arithmetic operators.
• 24v² – 69vr + 420r – 78 is an algebraic expression with v and r as the variables, 24, 69, 420 as the coefficients, 78 as the constant term with addition, exponent, subtraction and multiplication as the arithmetic operators.

指数定律

为指数制定了不同的定律以使复杂的计算更容易，例如，指数可以分为两个，如果两个项具有相同的基数，则可以使用一个定律来解决，等等。让我们来看看这些法律更详细，

• 法则 1（乘积法则）：当同一个底以不同的指数与自身相乘时，这些指数相加，即 p ^u × p ^v = p ^{u + v} 。

例子：

1. 62³⁷ × 62⁵⁰ = 62^{37 + 50} = 62⁸⁷
2. 74^-12 × 74³² × 74¹⁰¹ = 74^{-12 + 32 + 101} = 74¹²¹

• 法则 2（商法则）：如果同一个底与它自己的除法具有不同的指数，则在分子中减去这些指数，即 p ^m ÷ p ⁿ = p ^mn 。

例子：

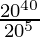1. $\frac{20^{40}}{20^5}$= 2^{40 – 5} = 2³⁵
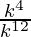2. $\frac{k^4}{k^{12}}$= k^{4 – 12} = k^-8

• 定律 3（零指数定律）：当一个基数被提升到 0 次方时，它的值总是 1，p ⁰ = 1。

例子：

• 20⁰ = 1
• 100⁰ = 1
• 69420⁰ = 1

• 法则 4（幂律）：如果将指数进一步提升为指数，则首先将两个指数相乘，然后进行进一步计算，即 (p ^m ) ⁿ = p ^mn 。

例子：

1. (112³⁰)⁴⁰ = (112)^{30 × 40} = 112¹²⁰⁰
2. [(-13)^-90]² = (-13)^{-90 × 2} = (-13)^-180

• 定律 5：如果两个具有相同指数的不同基在相乘中，它们的乘积会上升到给定的指数，即 p ^m × q ^m = (p × q) ^m 。

例子：

1. 40³⁶ × 100³⁶ = (40 × 100)³⁶ = 4000³⁶
2. 20¹³ × 16¹³ = (20 × 16)¹³ = 320¹³

• 法则 6：如果给定小数指数 p ^m/n = $\sqrt[n]{p^m}$ .

例子：

1. 20^1/2 = √2
2. 20^1/3 = $\sqrt[3]{20}$
3. 692^44/5 = $\sqrt[5]{692^{44}}$

• 法则 7（负指数法则）：如果底的指数为负，则倒数底，即 p ^-m = $\frac{1}{p^m}$ .

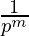例子：

1. 2^-91 = $\frac{1}{2^{91}}$
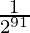
2. 69420^-80 = $\frac{1}{69420^{80}}$

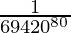
简化 8/5x ^-2/3 。

解决方案：

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

 8/ 5x^-2/3 = $\frac{8}{5}x^{\frac{2}{3}}$

Therefore the answer is $\frac{8}{5}x^{\frac{2}{3}}$

类似问题

问题 1：简化：1/ 2x ^-99 。

解决方案：

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

1/ 2x^-99 = $\frac{1}{2}x^{99}$

= x⁹⁹/ 2.

问题 2：简化：4/3x ^-9 。

解决方案：

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

4/3x^-9 = $\frac{4}{3}x^9$

问题 3：简化：12x ⁹ / 5x ⁶⁰ 。

解决方案：

Using the property a^m/ aⁿ = a^{m – n}, which is known as the quotient law,

12×9/ 5×60 = $\frac{12x^{9-60}}{5}$

= 12x^-51/ 5

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

12x^-51/ 5 = $\frac{12}{5x^{51}}$.

问题 4. 简化：3x ² / 10x ⁵ 。

解决方案：

Using the property a^m/ aⁿ = a^m-n, which is known as the quotient law,

3x²/ 10x⁵ = $\frac{3x^{2-5}}{10}$

= 3x^-3/ 5

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

3x^-3/ 5 = $\frac{3}{10x^{3}}.$

问题 5. 化简： 2x ⁴ / 5y ^-10 。

解决方案：

Using the property a^-m = 1/ a^m, which is known as the Negative exponent law,

2x⁴/ 5y^-10 = $\frac{2x^4y^{10}}{5}$