简化流程

在外力作用下能改变其形态的物质被定义为流体。每当对流体施加外力时，它就会开始流动。对运动中的流体的研究被定义为流体动力学。你有没有注意到桥下有一条小溪流过？当您看到流线型时，首先想到的是什么？最典型的溪流品质，例如速度、宽度和水流的体积，就是答案。任何流体的流动都是最重要的特性之一。溪流的位置或来源是决定其中水流的重要因素。溪流的源站有可能改变溪流内的流速和流动方向。

影响流的流动的另一个关键方面是流中对象的物理存在。溪流中的物体决定了流水的方向和速度。液体流动的速度是其最重要的特征。由于上述特性，水可以分为流线或层流或湍流。

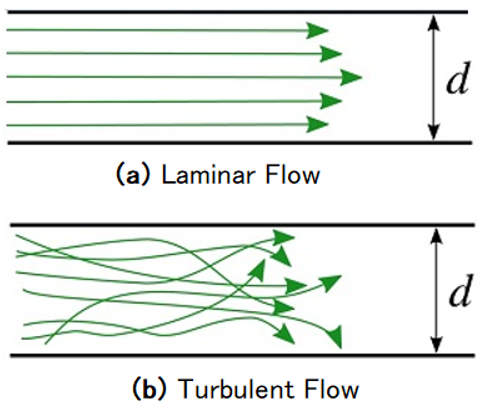
流体可以多种形式流动；可以通过自来水的例子来理解。来自水龙头的水在流速较低时具有光滑的外观，但当流速增加时，会在某个点出现空隙和中断。

如果在流量恒定时添加墨水流，则墨水会在不与其他层混合的情况下流动，但如果在流动湍流时引入墨水，则墨水层会与其他水层混合，如图所示以下。第一种类型，称为流线或层流，将在本节中讨论。另一个是湍流，稍后将讨论。

简化流程

流体中的流线流动被描述为这样一种流动，其中流体在平行层中流动，没有层间中断或混合，并且通过的每个流体粒子的速度在特定位置随时间保持恒定。

在低流体速度下没有湍流速度变化，并且流体倾向于在没有横向混合的情况下流动。流体颗粒相对于沿平行于管壁的直线行进的颗粒以特定顺序移动，使得相邻层像扑克牌一样滑过彼此。

精简

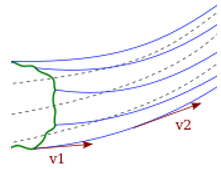
流体粒子在稳定流动情况下所经过的路径被定义为流线。如果流线表示为曲线，则曲线上任意点的切线揭示了当时流体速度的方向。如下图所示，曲线表示流体粒子如何随时间移动。

该曲线表示流体粒子在稳态下的流动。这张地图是时间静止的，这意味着每个通过一个点的粒子的行为都与之前的粒子完全相同。

Streamline 流的表示

In a laminar flow, the streamlines follow the equation of continuity. i.e., 

Av = Constant, 

where A is the fluid flow’s cross-sectional area and v is the fluid’s velocity at that location.

The term Av is defined as the fluid’s volume flux or flow rate, which is constant in a steady flow. 

Let us consider the cross-sectional area at Point 1 to be A₁ and at point 2 to be A₂, 

Then by the equation of continuity: 

A₁v₁ = A₂v₂

This represents the inverse relationship between area and velocity. The velocity of the liquid decreases as the cross-section area increases, and vice versa.

此外，水的流动也可以通过称为雷诺数的量来确定，该量确定流动模式是层流还是湍流，这将在下面讨论：

雷诺数

雷诺数是一个无量纲量，用于确定流型在通过管道时是层流还是湍流。惯性力与粘性力之比决定了雷诺数。

The formula for the Reynolds number is given as:

R_e = (p × V × D) / u

where:

• R_e is the Reynolds number,
• p is the density of the fluid,
• V is the velocity of flow,
• D is the diameter of the pipe and
• u is the viscosity of the fluid.

以下是流体流动和雷诺数的要点：

• 如果计算出的雷诺数很高，则称通过管道的流动是湍流。
• 如果雷诺数较低，则称流动为层流。
• 这些是可接受的数值，而层流和湍流通常在一个范围内分组。
• 层流的雷诺数小于 2000，而湍流的雷诺数大于 2000。

示例问题

问题1：连续性方程基于哪个定理？

解决方案：

The flow of the fluid is assumed to be incompressible, laminar and non-viscous for using the continuity equation. The continuity equation is based on the conservation of mass. The product of area and velocity gives the volume flow rate of fluid. Since, the density of fluid is same at all points, the mass of fluid passing through two different regions is same at the same time.

问题2：具有均匀横截面的管子保持垂直，这样水从顶部进入并从底部离开。如果开口下方 A 点的速度为“v”，那么 A 和 B 之间的距离为“2h”的垂直下方 A 点 B 处的速度是多少？

解决方案：

According to the equation of continuity, the velocity at B will be the same as that at A since the area of the cross-section is the same at both points. Although, gravity will increase its velocity, but pressure will also decrease downwards and therefore net effect will be zero change in velocity.

问题3：水平管道以流线型输送水。在管道沿线的一点，横截面积为 25 cm^2，水流速度为 0.5 m/s。求截面积为 2 cm^2 处的水流速度。

解决方案：

Given:

Area at point 1, A₁ = 25 cm²

Area at point 2, A₂ = 2 cm²

Velocity of point 1, v₁ = 0.5 m/s

Let us consider the unknown velocity be v₂.

According to continuity equation,

A₁v₁ = A₂v₂

Rearrange the formula in terms of v₂.

v₂ = A₁v₁ / A₂

Substitute all the values in the above expression.

v₂ = (25 cm²) × (0.5 m/s) / (2 cm²)

    = 6.25 m/s

Thus, the velocity of water at a point of second cross-section is 6.25 m/s.

问题 4：确定相对密度为 100 kg/m ³ 、粘度为 0.5 N s/m ²的流体以 5 m/s 的速度通过 0.2 m 管道的流量。

解决方案：

The type of flow can be determined by the value of Reynolds number.

Given:

Velocity of fluid, V = 5 m/s

Diameter of pipe, D = 0.2 m

Relative density of fluid, p =100 kg/m³

Viscosity of fluid = 0.5 N s/m²

The formula of Reynolds number is given as:

R_e = (p × V × D) / u

Substitute all the values in the formula to calculate the Reynolds number.

R_e = (100 kg/m³) × (5 m/s) × (0.2 m) / (0.5 N s/m²)

   = 200

Since, the Reynolds number is less than 2000, therefore the flow of liquid is laminar.

问题5：为什么两条流线永远不会相交？

解决方案：

The direction of net velocity of the flow is given by the tangent at a point to the streamline. 

If the two streamlines cross, it denotes two different directions of velocity at a point, which are not feasible. As a result, two streamlines cannot intersect.