最大化由给定矩形边上的点形成的三角形面积

给定一个矩形[(x1, y1), (x2, y2)]表示左下角和右上角的坐标，其边平行于坐标轴，其周边有N个点（每边至少一个） .任务是最大化由这些点形成的三角形的面积。

例子：

Input: rectangle[][]= {{0, 0}, {6, 6}}, 
coordinates[][] = {{0, 2}, {0, 3}, {0, 5}, {2, 0}, {3, 0}, {6, 0}, {6, 4}, {1, 6}, {6, 6}}
Output: 18
Explanation: Refer to the image below for explanation. 

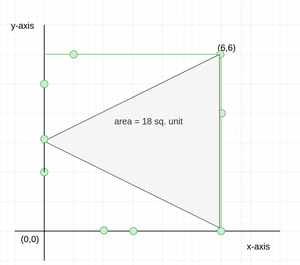
方法：要通过给定矩形上的坐标找到最大面积三角形，请找到相距最远的每一边的坐标。因此，假设有四个边a、b、c、d ，其中a 和 c是矩形的长度， b、d 是宽度。现在最大面积将是
MAX（长度*（任一宽度上最远坐标之间的距离）/2，宽度*（任一长度上最远坐标之间的距离）/2）。
请按照以下步骤解决给定的问题。

• 计算长度 = abs(x2 – x1)和宽度 = abs(y2 – y1)
• 找到每边最远的坐标。
• 使用上述公式计算面积。
• 返回找到的区域。

下面是上述方法的实现。

C++

// C++ program for above approach
#include 
#include 
using namespace std;
 
// To find the maximum area of triangle
void maxTriangleArea(int rectangle[2][2],
                     int coordinates[][2],
                     int numberOfCoordinates)
{
 
    int l1min = INT_MAX, l2min = INT_MAX,
        l1max = INT_MIN, l2max = INT_MIN,
        b1min = INT_MAX, b1max = INT_MIN,
        b2min = INT_MAX, b2max = INT_MIN;
 
    int l1Ycoordinate = rectangle[0][1];
    int l2Ycoordinate = rectangle[1][1];
 
    int b1Xcoordinate = rectangle[0][0];
    int b2Xcoordinate = rectangle[1][0];
 
    // Always consider side parallel
    // to x-axis as length and
    // side parallel to y-axis as breadth
    for (int i = 0; i < numberOfCoordinates;
         i++) {
        coordinates[i][1];
 
        // coordinate on l1
        if (coordinates[i][1] == l1Ycoordinate) {
            l1min = min(l1min,
                        coordinates[i][0]);
            l1max = max(l1max,
                        coordinates[i][0]);
        }
 
        // Coordinate on l2
        if (coordinates[i][1] == l2Ycoordinate) {
            l2min = min(l2min,
                        coordinates[i][0]);
            l2max = max(l2max,
                        coordinates[i][0]);
        }
 
        // Coordinate on b1
        if (coordinates[i][0] == b1Xcoordinate) {
            b1min = min(b1min,
                        coordinates[i][1]);
            b1max = max(b1max,
                        coordinates[i][1]);
        }
 
        // Coordinate on b2
        if (coordinates[i][0] == b2Xcoordinate) {
            b2min = min(b2min,
                        coordinates[i][1]);
            b2max = max(b2max,
                        coordinates[i][1]);
        }
    }
 
    // Find maximum possible distance
    // on length
    int maxOfLength = max(abs(l1max - l1min),
                          abs(l2max - l2min));
 
    // Find maximum possible distance
    // on breadth
    int maxofBreadth = max(abs(b1max - b1min),
                           abs(b2max - b2min));
 
    // Calculate result base * height / 2
    float result
        = max((maxofBreadth
               * (abs(rectangle[0][0]
                      - rectangle[1][0]))),
              (maxOfLength
               * (abs(rectangle[0][1]
                      - rectangle[1][1]))))
          / 2.0;
 
    // Print the result
    cout << result;
}
 
// Driver Code
int main()
{
    // Rectangle with x1, y1 and x2, y2
    int rectangle[2][2] = { { 0, 0 },
                            { 6, 6 } };
 
    // Coordinates on sides of given rectangle
    int coordinates[9][2]
        = { { 0, 2 }, { 0, 3 }, { 0, 5 },
            { 2, 0 }, { 3, 0 }, { 6, 0 },
            { 6, 4 }, { 1, 6 }, { 6, 6 } };
 
    int numberOfCoordinates
        = sizeof(coordinates) / sizeof(coordinates[0]);
 
    maxTriangleArea(rectangle, coordinates,
                    numberOfCoordinates);
    return 0;
}

Java

// Java program for the above approach
import java.io.*;
import java.lang.*;
import java.util.*;
 
class GFG {
 
  // To find the maximum area of triangle
  static void maxTriangleArea(int[ ][ ] rectangle,
                              int[ ][ ] coordinates,
                              int numberOfCoordinates)
  {
 
    int l1min = Integer.MAX_VALUE, l2min = Integer.MAX_VALUE,
    l1max = Integer.MIN_VALUE, l2max = Integer.MIN_VALUE,
    b1min = Integer.MAX_VALUE, b1max = Integer.MIN_VALUE,
    b2min = Integer.MAX_VALUE, b2max = Integer.MIN_VALUE;
 
    int l1Ycoordinate = rectangle[0][1];
    int l2Ycoordinate = rectangle[1][1];
 
    int b1Xcoordinate = rectangle[0][0];
    int b2Xcoordinate = rectangle[1][0];
 
    // Always consider side parallel
    // to x-axis as length and
    // side parallel to y-axis as breadth
    for (int i = 0; i < numberOfCoordinates; i++) {
 
      // coordinate on l1
      if (coordinates[i][1] == l1Ycoordinate) {
        l1min = Math.min(l1min,
                         coordinates[i][0]);
        l1max = Math.max(l1max,
                         coordinates[i][0]);
      }
 
      // Coordinate on l2
      if (coordinates[i][1] == l2Ycoordinate) {
        l2min = Math.min(l2min,
                         coordinates[i][0]);
        l2max = Math.max(l2max,
                         coordinates[i][0]);
      }
 
      // Coordinate on b1
      if (coordinates[i][0] == b1Xcoordinate) {
        b1min = Math.min(b1min,
                         coordinates[i][1]);
        b1max = Math.max(b1max,
                         coordinates[i][1]);
      }
 
      // Coordinate on b2
      if (coordinates[i][0] == b2Xcoordinate) {
        b2min = Math.min(b2min,
                         coordinates[i][1]);
        b2max = Math.max(b2max,
                         coordinates[i][1]);
      }
    }
 
    // Find maximum possible distance
    // on length
    int maxOfLength = Math.max(Math.abs(l1max - l1min),
                               Math.abs(l2max - l2min));
 
    // Find maximum possible distance
    // on breadth
    int maxofBreadth = Math.max(Math.abs(b1max - b1min),
                                Math.abs(b2max - b2min));
 
    // Calculate result base * height / 2
    int result
      = Math.max((maxofBreadth
                  * (Math.abs(rectangle[0][0]
                              - rectangle[1][0]))),
                 (maxOfLength
                  * (Math.abs(rectangle[0][1]
                              - rectangle[1][1]))))
      / 2;
 
    // Print the result
    System.out.print(result);
  }
 
  // Driver Code
  public static void main (String[] args)
  {
     
    // Rectangle with x1, y1 and x2, y2
    int[ ][ ] rectangle = { { 0, 0 },
                           { 6, 6 } };
 
    // Coordinates on sides of given rectangle
    int[ ][ ] coordinates
      = { { 0, 2 }, { 0, 3 }, { 0, 5 },
         { 2, 0 }, { 3, 0 }, { 6, 0 },
         { 6, 4 }, { 1, 6 }, { 6, 6 } };
 
    int numberOfCoordinates
      = coordinates.length;
 
    maxTriangleArea(rectangle, coordinates,
                    numberOfCoordinates);
  }
}
 
// This code is contributed by hrithikgarg03188.

Python3

# Python code for the above approach
 
# To find the maximum area of triangle
def maxTriangleArea(rectangle, coordinates, numberOfCoordinates):
 
    l1min = 10 ** 9
    l2min = 10 ** 9
    l1max = 10 ** -9
    l2max = 10 ** -9
    b1min = 10 ** 9
    b1max = 10 ** -9
    b2min = 10 ** 9
    b2max = 10 ** -9
 
    l1Ycoordinate = rectangle[0][1];
    l2Ycoordinate = rectangle[1][1];
 
    b1Xcoordinate = rectangle[0][0];
    b2Xcoordinate = rectangle[1][0];
 
    # Always consider side parallel
    # to x-axis as length and
    # side parallel to y-axis as breadth
    for i in range(numberOfCoordinates):
        coordinates[i][1];
 
        # coordinate on l1
        if (coordinates[i][1] == l1Ycoordinate) :
            l1min = min(l1min, coordinates[i][0]);
            l1max = max(l1max, coordinates[i][0]);
         
 
        # Coordinate on l2
        if (coordinates[i][1] == l2Ycoordinate):
            l2min = min(l2min, coordinates[i][0]);
            l2max = max(l2max, coordinates[i][0]);
 
 
        # Coordinate on b1
        if (coordinates[i][0] == b1Xcoordinate):
            b1min = min(b1min, coordinates[i][1]);
            b1max = max(b1max, coordinates[i][1]);
         
 
        # Coordinate on b2
        if (coordinates[i][0] == b2Xcoordinate):
            b2min = min(b2min, coordinates[i][1]);
            b2max = max(b2max, coordinates[i][1]);
         
 
    # Find maximum possible distance
    # on length
    maxOfLength = max(abs(l1max - l1min), abs(l2max - l2min));
 
    # Find maximum possible distance
    # on breadth
    maxofBreadth = max(abs(b1max - b1min), abs(b2max - b2min));
 
    # Calculate result base * height / 2
    result = max((maxofBreadth * (abs(rectangle[0][0] - rectangle[1][0]))),
            (maxOfLength * (abs(rectangle[0][1] - rectangle[1][1])))) / 2.0;
 
    # Print the result
    print(int(result));
 
 
# Driver Code
 
# Rectangle with x1, y1 and x2, y2
rectangle = [[0, 0],[6, 6]];
 
# Coordinates on sides of given rectangle
coordinates = [[0, 2], [0, 3], [0, 5],
    [2, 0], [3, 0], [6, 0],
    [6, 4], [1, 6], [6, 6]];
 
numberOfCoordinates = len(coordinates)
 
maxTriangleArea(rectangle, coordinates, numberOfCoordinates);
 
# This code is contributed by gfgking

C#

// C# program for the above approach
using System;
class GFG {
 
  // To find the maximum area of triangle
  static void maxTriangleArea(int[,] rectangle,
                              int[,] coordinates,
                              int numberOfCoordinates)
  {
 
    int l1min = Int32.MaxValue, l2min = Int32.MaxValue,
    l1max = Int32.MinValue, l2max = Int32.MinValue,
    b1min = Int32.MaxValue, b1max = Int32.MinValue,
    b2min = Int32.MaxValue, b2max = Int32.MinValue;
 
    int l1Ycoordinate = rectangle[0,1];
    int l2Ycoordinate = rectangle[1,1];
 
    int b1Xcoordinate = rectangle[0,0];
    int b2Xcoordinate = rectangle[1,0];
 
    // Always consider side parallel
    // to x-axis as length and
    // side parallel to y-axis as breadth
    for (int i = 0; i < numberOfCoordinates; i++) {
 
      // coordinate on l1
      if (coordinates[i,1] == l1Ycoordinate) {
        l1min = Math.Min(l1min,
                         coordinates[i,0]);
        l1max = Math.Max(l1max,
                         coordinates[i,0]);
      }
 
      // Coordinate on l2
      if (coordinates[i,1] == l2Ycoordinate) {
        l2min = Math.Min(l2min,
                         coordinates[i,0]);
        l2max = Math.Max(l2max,
                         coordinates[i,0]);
      }
 
      // Coordinate on b1
      if (coordinates[i,0] == b1Xcoordinate) {
        b1min = Math.Min(b1min,
                         coordinates[i,1]);
        b1max = Math.Max(b1max,
                         coordinates[i,1]);
      }
 
      // Coordinate on b2
      if (coordinates[i,0] == b2Xcoordinate) {
        b2min = Math.Min(b2min,
                         coordinates[i,1]);
        b2max = Math.Max(b2max,
                         coordinates[i,1]);
      }
    }
 
    // Find maximum possible distance
    // on length
    int maxOfLength = Math.Max(Math.Abs(l1max - l1min),
                               Math.Abs(l2max - l2min));
 
    // Find maximum possible distance
    // on breadth
    int maxofBreadth = Math.Max(Math.Abs(b1max - b1min),
                                Math.Abs(b2max - b2min));
 
    // Calculate result base * height / 2
    int result
      = Math.Max((maxofBreadth
                  * (Math.Abs(rectangle[0,0]
                              - rectangle[1,0]))),
                 (maxOfLength
                  * (Math.Abs(rectangle[0,1]
                              - rectangle[1,1]))))
      / 2;
 
    // Print the result
    Console.Write(result);
  }
 
  // Driver Code
  public static void Main ()
  {
 
    // Rectangle with x1, y1 and x2, y2
    int[,] rectangle = { { 0, 0 },
                        { 6, 6 } };
 
    // Coordinates on sides of given rectangle
    int[,] coordinates
      = { { 0, 2 }, { 0, 3 }, { 0, 5 },
         { 2, 0 }, { 3, 0 }, { 6, 0 },
         { 6, 4 }, { 1, 6 }, { 6, 6 } };
 
    int numberOfCoordinates
      = coordinates.GetLength(0);
 
    maxTriangleArea(rectangle, coordinates,
                    numberOfCoordinates);
  }
}
 
// This code is contributed by Samim Hossain Mondal.

Javascript

       // JavaScript code for the above approach
 
 
       // To find the maximum area of triangle
       function maxTriangleArea(rectangle,
           coordinates,
           numberOfCoordinates) {
 
           let l1min = Number.MAX_VALUE, l2min = Number.MAX_VALUE,
               l1max = Number.MIN_VALUE, l2max = Number.MIN_VALUE,
               b1min = Number.MAX_VALUE, b1max = Number.MIN_VALUE,
               b2min = Number.MAX_VALUE, b2max = Number.MIN_VALUE;
 
           let l1Ycoordinate = rectangle[0][1];
           let l2Ycoordinate = rectangle[1][1];
 
           let b1Xcoordinate = rectangle[0][0];
           let b2Xcoordinate = rectangle[1][0];
 
           // Always consider side parallel
           // to x-axis as length and
           // side parallel to y-axis as breadth
           for (let i = 0; i < numberOfCoordinates;
               i++) {
               coordinates[i][1];
 
               // coordinate on l1
               if (coordinates[i][1] == l1Ycoordinate) {
                   l1min = Math.min(l1min,
                       coordinates[i][0]);
                   l1max = Math.max(l1max,
                       coordinates[i][0]);
               }
 
               // Coordinate on l2
               if (coordinates[i][1] == l2Ycoordinate) {
                   l2min = Math.min(l2min,
                       coordinates[i][0]);
                   l2max = Math.max(l2max,
                       coordinates[i][0]);
               }
 
               // Coordinate on b1
               if (coordinates[i][0] == b1Xcoordinate) {
                   b1min = Math.min(b1min,
                       coordinates[i][1]);
                   b1max = Math.max(b1max,
                       coordinates[i][1]);
               }
 
               // Coordinate on b2
               if (coordinates[i][0] == b2Xcoordinate) {
                   b2min = Math.min(b2min,
                       coordinates[i][1]);
                   b2max = Math.max(b2max,
                       coordinates[i][1]);
               }
           }
 
           // Find maximum possible distance
           // on length
           let maxOfLength = Math.max(Math.abs(l1max - l1min),
               Math.abs(l2max - l2min));
 
           // Find maximum possible distance
           // on breadth
           let maxofBreadth = Math.max(Math.abs(b1max - b1min),
               Math.abs(b2max - b2min));
 
           // Calculate result base * height / 2
           let result
               = Math.max((maxofBreadth
                   * (Math.abs(rectangle[0][0]
                       - rectangle[1][0]))),
                   (maxOfLength
                       * (Math.abs(rectangle[0][1]
                           - rectangle[1][1]))))
               / 2.0;
 
           // Print the result
           document.write(result);
       }
 
       // Driver Code
 
       // Rectangle with x1, y1 and x2, y2
       let rectangle = [[0, 0],
       [6, 6]];
 
       // Coordinates on sides of given rectangle
       let coordinates
           = [[0, 2], [0, 3], [0, 5],
           [2, 0], [3, 0], [6, 0],
           [6, 4], [1, 6], [6, 6]];
 
       let numberOfCoordinates
           = coordinates.length;
 
       maxTriangleArea(rectangle, coordinates,
           numberOfCoordinates);
 
      // This code is contributed by Potta Lokesh
   

输出

18

时间复杂度： O(N)，其中 N 是给定的坐标数。
辅助空间： O(1)