阿尔法粒子散射和卢瑟福的原子核模型

在今天的宇宙中，最小的物质粒子是原子。这是通过将物质分开而不释放带电粒子而获得的最小可能结果。它最初是由约翰道尔顿以原子论的名义提出的。这个理论有自己的演变，由 JJ Thompson、Ernest Rutherford、Niels Bohr 和 Erwin Schrödinger 等不同的科学家给出。今天将讨论原子的卢瑟福模型。

卢瑟福模型

欧内斯特·卢瑟福 (Ernest Rutherford) 正在研究原子发射 α 粒子以了解原子的结构，关于卢瑟福进行的实验，盖革和马斯登自己进行了一些实验，如图所示。

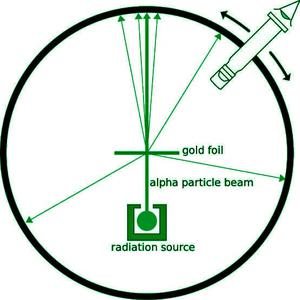
Geiger-Marsden 实验的实验装置

他们在一层薄薄的金箔上使用从放射源（铋）发射的 5.5 MeV 光束。光源发出的α粒子集中成一束光，照射在金箔上，集中后的光束在击中后被散射，在显微镜下可以在屏幕上看到。

进行了该实验，结果是在散射光束中，许多粒子无串通地穿过箔片，只有 0.14% 的入射粒子散射超过 1 ^o并且 8000 个粒子中有 1 个偏转超过 90 ^o 。这意味着粒子完全向后散射，这意味着一定有某种大的排斥力

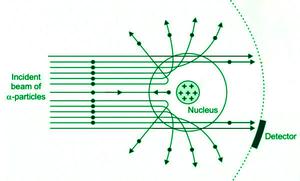
Why did that 1 in 8000 deflect?

Rutherford said from the observation that the greater repulsive force can be provided if the mass and positive charge is concentrated at the center which will cause the reflection. 

所以，在卢瑟福的原子模型中，所有的正电荷和质量都在中心，电子会像行星在一定距离内围绕太阳移动一样围绕它移动。

卢瑟福说原子的大小大约是 10 ^-15但实际上它是 10 ^-10并且随着电子在一定距离处移动，原子大部分是空的空间。因此，由于原子中有这么大的空白空间，很明显大多数α粒子直接穿过原子，当任何α粒子靠近原子核时，它们会因电场而散射。

α粒子的散射

众所周知，金箔很薄，因此我们可以假设α粒子不会遭受一次以上的碰撞。因此，需要对单个核进行计算。 α 粒子是氦原子的原子核，因此带有 2 个单元 (2e)。金核的电荷是Ze，其中Z是金的原子序数。

在这个实验中，金粒子比α粒子重得多，发生散射时金粒子不会移动。该轨迹可以利用牛顿第二运动定律和库仑定律计算出α粒子与带正电的原子核之间的静电排斥力，可以写为：

F = 1/(4πε ₀ ) × (2e)(Ze)/r ²

在哪里，

• r = α粒子和原子核之间的距离。
• Ze = 金核的电荷

阿尔法粒子轨迹

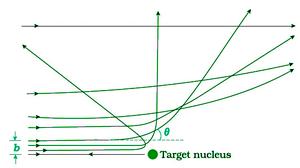
α粒子的运动轨迹取决于撞击参数（α粒子初速度矢量与原子核中心的垂直距离。上图中，α粒子有多种散射的概率，和可以看到靠近原子核的α粒子受到的散射比较大，现在直接撞击原子核的α粒子甚至可以面对180度的散射。

但是通过观察可以看出，由于返回的粒子数量很大，因此发生这种 180 度偏差的粒子并不多。

卢瑟福核模型中的电子轨道

该模型涉及经典概念，它们是，

• 它看起来原子是一个电中性球体。
• 它的中心有一个非常小、巨大且带正电的原子核。
• 中心的原子核被旋转的电子包围。
• 这些电子在各自的轨道上围绕原子核旋转。
• 旋转的电子和原子核之间的静电吸引力 (F _e ) 提供了维持电子围绕原子核旋转所需的向心力 (F _c )]

因此，这可以表示为，

F _e – F _c

[1/(4πε _o )] × [e ² /r ² ] = mv ² /r

因此轨道半径和电子速度之间的关系是

r = e ² /(4πε _o mv ² )

众所周知，动能 (k) = 1/2 × (mv ² )

那么动能就变成了，

K = e ² /(8πε _o r)

同样，势能 (U) 变为，

U = -e ² /(4πε _o )

因此总能量变成动能和势能之和

T = K + U = [e ² /(8πε _o r) + (-e ² )/(4πε _o r))]

T = -e ² /(8πε _o r)

因此，电子的总能量为负，这意味着电子与原子核电键合。

示例问题

问题 1：在卢瑟福的原子核模型中，原子核类似于太阳，电子围绕其围绕轨道（半径 ≅ 10 ^-10 m）移动，就像地球围绕太阳运行一样。如果太阳系的尺寸与原子的尺寸比例相同，那么地球会比实际更接近还是远离太阳？地球轨道的半径约为1.5×10 ¹¹ m。太阳的半径取为 8 × 10 ⁸

解决方案：

The ratio of the radius of electron’s orbit to the radius of nucleus is (10^-10m/10^-15m) = 10⁵ 

therefore it is seen that the radius of the electron’s orbit is 10⁵ times larger than radius of nucleus.

If we consider the same situation for the earth and the sun i.e. earth’s orbit around sun were 10⁵ times larger than radius of the sun, then the radius of the earth according this condition will be 

10⁵ × 8 × 10⁸m = 8 × 10¹³ m which is without doubt prolonged than the actual orbit of earth and hence earth would be much farther away from the sun.

It also implies that an atom contains a much greater fraction of empty space as compared to our solar system.

问题2：实验发现，将氢原子分离成质子和电子需要13.6 eV的能量。计算氢原子中电子的轨道半径和速度。

解决方案：

Total energy of electron in hydrogen atom is -13.6 eV = -13.6 × 1.6 × 10^-19  J

According to relation between orbit radius and electron velocity we get,

E = -e²/(8πε_o r)

E = -2.2 × 10^-18 J

Thus the orbital radius becomes,

r = -e²/(8πε_o E)

r = [(9 ×10⁹  N m² /C²) (1.6 × 10^-19 C)² ] / [(2) (-2.2 × 10^-18 J)]

r = 5.3 × 10^-11 m. 

Thus velocity of the electron can be computed by 

v = e / (√4 πε_omr)

v =2.2 × 10⁶ m/s

概念问题

问题 1：盖革和马斯登的 α 粒子散射实验中使用了哪种放射源？

回答：

 The radioactive element used in the alpha scattering experiment is an radioactive isotope of Bismuth (214 Bi 83).

问题 2： 90 ^o的 Alpha 散射实验中的偏转比是多少？

回答：

1 out of 8000 of the Alpha particles were deflected over 90^o .

问题 3：解释 Alpha 散射实验中 Alpha 粒子撞击金箔时的行为。

回答：

When the alpha particles from the source hit the gold foil, many of them pass straight through the gold foil, just about 0.14 % of the particles which collide with the nucleus scatter more than 1^o, 1 in 8000 particle deflect more than 90° and Very few of them hit the nucleus head on and returned back to the source’s direction i.e. 180^o.

问题4：哪两个定律用于计算碰撞的阿尔法粒子的轨迹？

回答：

The Newton’s second law of motion and coulomb’s law of electrostatic force of repulsion between α-particles and positively charged nucleus.

问题 5：陈述卢瑟福核模型给出的原子的任意两个性质。

回答：

The properties of an atom given by Rutherford’s Nuclear Model are:

1. The nucleus at the center is surrounded by revolving electrons.
2. These electrons revolves at their respective orbits around nucleus.

问题6：为什么阿尔法粒子与原子核碰撞时会改变轨迹？为什么没有阿尔法粒子？

回答：

Alpha particles are very lighter then the Gold particles. When two substance have different masses, and the lighter one collided with the heavier one, the lighter one is deflected from its path.

问题7：为什么粒子在原子核不同区域碰撞的轨迹不同？

回答：

According to the observation from the scattering experiment the nucleus have a repulsive force and it is concentrated at the center. So When the electron is collided head on it is deflected back at 180^o  and when the particles are collided further above or below the center or even passes close to the nucleus its is deflected to its respective angle.