可以内切到立方体的最大球体，再切入右圆锥体的立方体

这里给出半径为r且垂直高度为h的右圆锥体，该圆锥体被刻在一个立方体中，而立方体又被刻在一个球体中，任务是找到球体的半径。
例子：

Input: h = 5, r = 6 
Output: 1.57306

Input: h = 8, r = 11
Output: 2.64156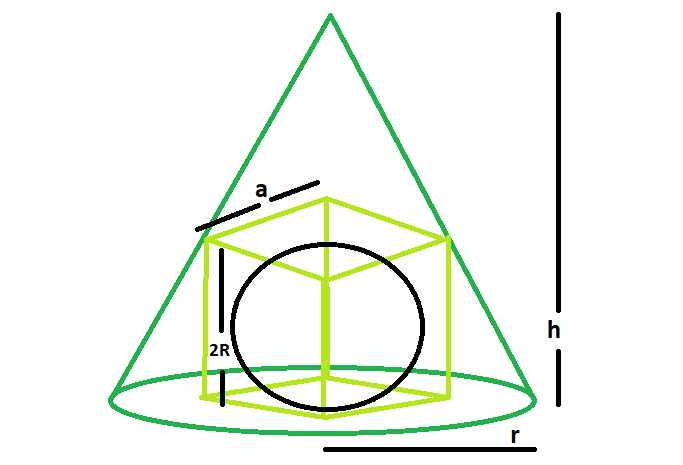

方法：

• 令立方体的边= a
• 令球体的半径= R
• 我们知道， a = h *r√2//(h +√2* r) (请参阅此处)
• 另外， R = a / 2 (请参阅此处)
• 因此， R =(h *r√2/ 2 /(h +√2* r))/ 2

下面是上述方法的实现：

C++

// C++ Program to find the biggest sphere
// which is inscribed within a cube which in turn
// inscribed within a right circular cone
 
#include 
using namespace std;
 
// Function to find the radius of the sphere
float sphereSide(float h, float r)
{
    // height and radius cannot be negative
    if (h < 0 && r < 0)
        return -1;
 
    // radius of the sphere
    float R = ((h * r * sqrt(2)) / (h + sqrt(2) * r)) / 2;
 
    return R;
}
 
// Driver code
int main()
{
    float h = 5, r = 6;
 
    cout << sphereSide(h, r) << endl;
 
    return 0;
}

Java

// Java Program to find the biggest sphere
// which is inscribed within a cube which in turn
// inscribed within a right circular cone
import java.lang.Math;
 
class GFG
{
     
// Function to find the radius of the sphere
static float sphereSide(float h, float r)
{
    // height and radius cannot be negative
    if (h < 0 && r < 0)
        return -1;
 
    // radius of the sphere
    float R = (float)((h * r * Math.sqrt(2)) /
                    (h + Math.sqrt(2) * r)) / 2;
 
    return R;
}
 
// Driver code
public static void main(String[] args)
{
    float h = 5, r = 6;
 
    System.out.println(sphereSide(h, r));
 
}
}
 
// This code is contributed by Code_Mech.

Python3

# Program to find the biggest sphere
# which is inscribed within a cube which in turn
# inscribed within a right circular cone
import math
 
# Function to find the radius of the sphere
def sphereSide(h, r):
 
    # height and radius cannot be negative
    if h < 0 and r < 0:
        return -1
 
    # radius of the sphere
    R = (((h * r * math.sqrt(2))) /
              (h + math.sqrt(2) * r) / 2)
 
    return R
 
# Driver code
h = 5; r = 6
print(sphereSide(h, r))
 
# This code is contributed by Shrikant13

C#

// C# Program to find the biggest sphere
// which is inscribed within a cube which in turn
// inscribed within a right circular cone
using System;
 
class GFG
{
     
// Function to find the radius of the sphere
static float sphereSide(float h, float r)
{
    // height and radius cannot be negative
    if (h < 0 && r < 0)
        return -1;
 
    // radius of the sphere
    float R = (float)((h * r * Math.Sqrt(2)) /
                      (h + Math.Sqrt(2) * r)) / 2;
 
    return R;
}
 
// Driver code
public static void Main()
{
    float h = 5, r = 6;
 
    Console.WriteLine(sphereSide(h, r));
}
}
 
// This code is contributed by Code_Mech

Javascript

 
// javascript Program to find the biggest sphere
// which is inscribed within a cube which in turn
// inscribed within a right circular cone
 
// Function to find the radius of the sphere
function sphereSide(h , r)
{
    // height and radius cannot be negative
    if (h < 0 && r < 0)
        return -1;
 
    // radius of the sphere
    var R = ((h * r * Math.sqrt(2)) /
                    (h + Math.sqrt(2) * r)) / 2;
 
    return R;
}
 
// Driver code
var h = 5, r = 6;
 
document.write(sphereSide(h, r).toFixed(5));
 
 
// This code is contributed by Amit Katiyar
 

输出：

1.57306