给定整数N ,任务是检查整数的Digit Cube极限是否到达固定点或极限循环。
A Digit Cube Limit is a number which repeatedly arrives at a point if its value is computed as the sum of cubes of its digits, i.e. they have the following properties:
- Arrive at a fixed point if it is an Armstrong number.
- Arrive at a limit cycle if it is repeating in a cycle.
例子:
Input: N = 3
Output: Reached to fixed point 153
Explanation:
F(3) = 3 * 3 * 3 = 27
F(27) = 2*2*2 + 7*7*7 = 351
F(351) = 3*3*3 + 5*5*5 + 1*1*1 = 153
F(153) = 1*1*1 + 5*5*5 + 3*3*3 = 153
Since a fixed point(= 153) was obtained, which is an Armstrong number of order 3. Below is the illustration:

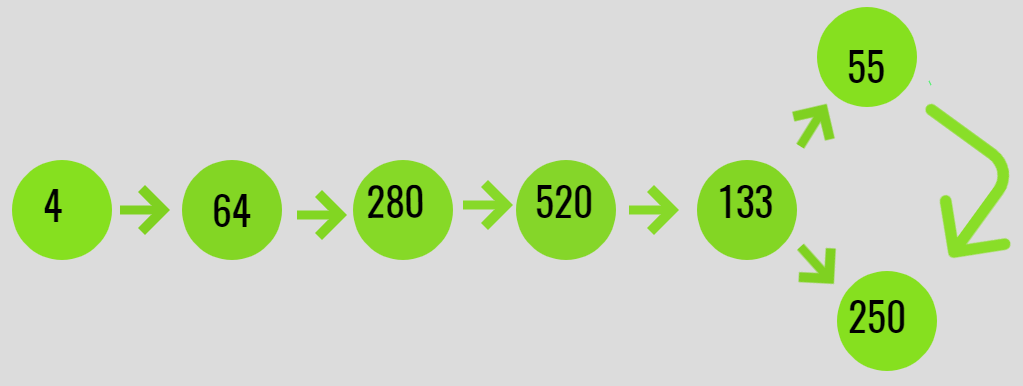
Input: N = 4
Output: Arrived at cycle
Explanation:
F(4) = 4 * 4 * 4 = 64
F(64) = 6 * 6 * 6 + 4 * 4 * 4 = 280
F(280) = 2 * 2 * 2 + 8 * 8 * 8 + 0 * 0 * 0 = 520
F(520) = 5 * 5 * 5 + 2 * 2 * 2 + 0*0*0 = 133
F(133) = 1*1*1 + 3*3*3 + 3*3*3 = 55
F(55) = 5*5*5 + 5*5*5 = 250
F(250) = 5*5*5 + 2*2*2 + 0*0*0 = 133
A cycle between
133 -> 55 -> 250 -> 133 is obtained.
Below is the illustration of the same:
方法:请按照以下步骤解决问题:
- 创建一个hashMap来存储迭代过程中数字的数字立方的总和。
- 迭代一个数字的位的总和的下一个值,并检查hashMap中是否存在下一个的数字的总和。
- 如果该数字已经在哈希图中,则检查该数字是否为阿姆斯特朗数字。如果发现为真,则数字达到固定点。
- 否则,如果该号码不是Armstrong号码,则继续。
下面是上述方法的实现:
C++
// C++ program for the above approach
#include
#include
// Define the limit
#define limit 1000000000
using namespace std;
// Function to get the sum of cube
// of digits of a number
long long F(long long N)
{
// Convert to string to get sum
// of the cubes of its digits
string str = to_string(N);
long long sum = 0;
for (long long i = 0;
i < str.size(); i++) {
long long val
= int(str[i] - '0');
sum += val * val * val;
}
// return sum
return sum;
}
// Function to check if the number
// arrives at a fixed point or a cycle
long long findDestination(long long N)
{
// Stores the values obtained
set s;
long long prev = N, next;
// Insert N to set s
s.insert(N);
while (N <= limit) {
// Get the next number using F(N)
next = F(N);
// Check if the next number is
// repeated or not
auto it = s.find(next);
if (it != s.end()) {
return next;
}
prev = next;
s.insert(prev);
N = next;
}
return next;
}
// Function to check if digit cube
// limit of an integer arrives at
// fixed point or in a limit cycle
void digitCubeLimit(long long N)
{
// N is a non negative integer
if (N < 0)
cout << "N cannot be negative\n";
else {
// Function Call
long long ans
= findDestination(N);
// If the value received is
// greater than limit
if (ans > limit)
cout << "Limit exceeded\n";
// If the value received is
// an Armstrong number
else if (ans == F(ans)) {
cout << N;
cout << " reaches to a"
<< " fixed point: ";
cout << ans;
}
else {
cout << N;
cout << " reaches to a"
<< " limit cycle: ";
cout << ans;
}
}
}
// Driver Code
int main()
{
long long N = 3;
// Function Call
digitCubeLimit(N);
return 0;
} Java
// Java program for the
// above approach
import java.util.*;
class GFG{
// Define the limit
static final int limit = 1000000000;
// Function to get the sum of cube
// of digits of a number
static int F(int N)
{
// Convert to String to get sum
// of the cubes of its digits
String str = String.valueOf(N);
int sum = 0;
for (int i = 0;
i < str.length(); i++)
{
int val = (int)(str.charAt(i) - '0');
sum += val * val * val;
}
// return sum
return sum;
}
// Function to check if the number
// arrives at a fixed point or a cycle
static int findDestination(int N)
{
// Stores the values obtained
HashSet s = new HashSet<>();
int prev = N, next =0;
// Insert N to set s
s.add(N);
while (N <= limit)
{
// Get the next number
// using F(N)
next = F(N);
// Check if the next number is
// repeated or not
if (s.contains(next))
{
return next;
}
prev = next;
s.add(prev);
N = next;
}
return next;
}
// Function to check if digit cube
// limit of an integer arrives at
// fixed point or in a limit cycle
static void digitCubeLimit(int N)
{
// N is a non negative integer
if (N < 0)
System.out.print("N cannot be negative\n");
else
{
// Function Call
int ans = findDestination(N);
// If the value received is
// greater than limit
if (ans > limit)
System.out.print("Limit exceeded\n");
// If the value received is
// an Armstrong number
else if (ans == F(ans))
{
System.out.print(N);
System.out.print(" reaches to a" +
" fixed point: ");
System.out.print(ans);
}
else
{
System.out.print(N);
System.out.print(" reaches to a" +
" limit cycle: ");
System.out.print(ans);
}
}
}
// Driver Code
public static void main(String[] args)
{
int N = 3;
// Function Call
digitCubeLimit(N);
}
}
// This code is contributed by Rajput-Ji Python3
# Python3 program for the above approach
# Define the limit
LIMIT = 1000000000
# Function to get the sum of cube
# of digits of a number
def F(N: int) -> int:
# Convert to string to get sum
# of the cubes of its digits
string = str(N)
sum = 0
for i in range(len(string)):
val = int(ord(string[i]) - ord('0'))
sum += val * val * val
# Return sum
return sum
# Function to check if the number
# arrives at a fixed point or a cycle
def findDestination(N: int) -> int:
# Stores the values obtained
s = set()
prev = N
next = 0
# Insert N to set s
s.add(N)
while (N <= LIMIT):
# Get the next number using F(N)
next = F(N)
# Check if the next number is
# repeated or not
if next in s:
return next
prev = next
s.add(prev)
N = next
return next
# Function to check if digit cube
# limit of an integer arrives at
# fixed point or in a limit cycle
def digitCubeLimit(N: int) -> int:
# N is a non negative integer
if (N < 0):
print("N cannot be negative")
else:
# Function Call
ans = findDestination(N)
# If the value received is
# greater than limit
if (ans > LIMIT):
print("Limit exceeded")
# If the value received is
# an Armstrong number
elif (ans == F(ans)):
print("{} reaches to a fixed point: {}".format(
N, ans))
else:
print("{} reaches to a limit cycle: {}".format(
N, ans))
# Driver Code
if __name__ == "__main__":
N = 3
# Function Call
digitCubeLimit(N)
# This code is contributed by sanjeev2552C#
// C# program for the
// above approach
using System;
using System.Collections.Generic;
class GFG{
// Define the limit
static readonly int limit =
1000000000;
// Function to get the sum
// of cube of digits of a
// number
static int F(int N)
{
// Convert to String to get sum
// of the cubes of its digits
String str = String.Join("", N);
int sum = 0;
for (int i = 0;
i < str.Length; i++)
{
int val = (int)(str[i] - '0');
sum += val * val * val;
}
// return sum
return sum;
}
// Function to check if the
// number arrives at a fixed
// point or a cycle
static int findDestination(int N)
{
// Stores the values
// obtained
HashSet s =
new HashSet();
int prev = N, next = 0;
// Insert N to set s
s.Add(N);
while (N <= limit)
{
// Get the next number
// using F(N)
next = F(N);
// Check if the next
// number is repeated
// or not
if (s.Contains(next))
{
return next;
}
prev = next;
s.Add(prev);
N = next;
}
return next;
}
// Function to check if digit cube
// limit of an integer arrives at
// fixed point or in a limit cycle
static void digitCubeLimit(int N)
{
// N is a non negative integer
if (N < 0)
Console.Write("N cannot be negative\n");
else
{
// Function Call
int ans = findDestination(N);
// If the value received is
// greater than limit
if (ans > limit)
Console.Write("Limit exceeded\n");
// If the value received is
// an Armstrong number
else if (ans == F(ans))
{
Console.Write(N);
Console.Write(" reaches to a" +
" fixed point: ");
Console.Write(ans);
}
else
{
Console.Write(N);
Console.Write(" reaches to a" +
" limit cycle: ");
Console.Write(ans);
}
}
}
// Driver Code
public static void Main(String[] args)
{
int N = 3;
// Function Call
digitCubeLimit(N);
}
}
// This code is contributed by gauravrajput1 3 reaches to a fixed point: 153
时间复杂度: O(N)
辅助空间: O(N)