📌  相关文章
📜  基于库仑定律的问题

📅  最后修改于: 2022-05-13 01:57:37.090000             🧑  作者: Mango

基于库仑定律的问题

物理宇宙中的每一个物理事件都包含某种类型的吸引力或排斥力，从而导致宇宙以独特的方式存在。由于粒子之间的吸引和排斥，环境保持在设备齐全和平衡的状态。当这些吸引力和排斥力被操纵以扰乱或改变时，观察的结果可能是显着的。

考虑一个原子的电子：如果原子核中的质子和壳中的电子之间的吸引力被破坏，原子就会被破坏。物理学家长期以来一直对物理学的定量方面着迷，因为它有助于理解概念和发展新的理论和思想。

1785 年，一位名叫查尔斯·奥古斯丁·德·库伦的法国物理学家创造了两个带电体之间可测量的数学关系。库仑定律，通常称为库仑平方反比定律，是一个有助于确定两个带电粒子之间斥力或吸引力程度的方程。

库仑定律

库仑定律描述了两个点电荷之间存在的力。术语点电荷是指线性带电物体的尺寸与物理学中它们之间的距离相比相对较小的事实。因此，我们将它们视为点电荷，因为计算它们之间的吸引力/排斥力很简单。

因此，库仑定律可以表述为：

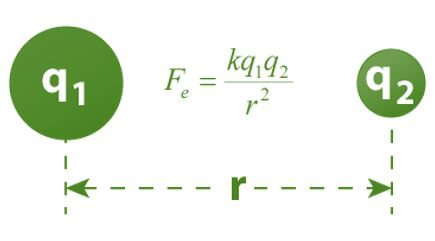
库仑定律的图示

一般来说，该语句有两个收费，q 1 和q 2 。电荷之间的吸引力/排斥力为“F”，它们之间的距离为“r”。然后在数学上给出库仑定律：

  • 静电力 F 与接触电荷量的乘积成正比，即F ∝ q 1 q 2
  • 静电力 F 与接触的两个电荷之间的距离的平方成反比，即F ∝ 1/r 2

让我们将上述与关系结合为

F ∝ q 1 q 2 / r 2

现在，引入一个新的比例常数 k，

F = kq 1 q 2 / r 2

其中 k 是比例常数，等于 1/4πε0，这里 ε0 称为 epsilon not，表示真空的介电常数。 k的计算值为9×10 9 Nm 2 / C 2 。

根据库仑的说法，同种电荷相互排斥，而异种电荷相互吸引。这意味着同号的电荷会相互排斥，而异号的电荷会相互吸引。

向量形式的库仑定律

有两种物理量：标量（只有一个量级）和向量（有很多量级）（具有大小和方向的量）。因为它既有大小又有方向，所以力是一个向量。以向量的形式，库仑定律可以改写。请记住，向量“F”表示为\vec{F} ，向量“r”表示为\vec{r} ， 等等。

假设有两个电荷 q 1和 q 2 ，其中 r 1和 r 2作为它们的位置向量。因为两个电荷的符号相同，所以它们之间会存在排斥力。令F 12是由于q 2对q 1电荷的作用力，而F 21是由于q 1电荷作用在q 2电荷上的作用力。 r 21向量是从 q 1到 q 2的匹配向量。

\overrightarrow{F}_{21}=\frac{1}{4\pi{\epsilon}_\circ}\frac{q_1q_2}{{r}_{21}^2}\hat{r}_{21}\\ \overrightarrow{F}_{21}=\frac{1}{4\pi{\epsilon}_\circ}\frac{q_1q_2}{{r}_{21}^3}\overrightarrow{r}_{21}

使用库仑定律计算两个点电荷之间的力时，请记住以下几点。因为这两种电荷本质上是相反的，所以方程的矢量形式不受它们的符号的影响。

由于位置矢量的变化，斥力 F 21是由于 q 2作用于电荷 q 1的力，和另一个斥力 F 21是由于 q 1作用于电荷 q 2的作用力。 .

F 12 = – F 21

这是因为力 F 12的位置矢量是 r 12 ，而力 F 21的位置矢量是 r 21 。

r 21 = r 2 – r 1

和

r 12 = r 1 – r 2

因为 r 21和 r 12的符号是相反的，所以它们会产生相反符号的力。这表明库仑定律与牛顿第三定律是一致的，牛顿第三定律指出每个动作都有一个相等和相反的反应。当真空中存在两个电荷时，库仑定律决定了它们之间的力。这是因为在真空中，电荷不受其他物质或粒子的影响。

库仑定律的局限性

库仑定律基于一组假设，不能以与其他通用公式相同的方式使用。法律涵盖以下几点：

  • 如果电荷是静态的，我们可以利用公式（在静止位置）
  • 当处理具有正常和平滑形状的电荷时，该公式易于使用；但是，当处理形状不规则的电荷时，它变得太复杂了。
  • 只有当粒子之间的溶剂分子明显大于两个电荷时，该公式才有效。

基于库仑定律的问题

问题1：两个点电荷+2μC和+4μC之间的静电力在20N的力下相互排斥，当它们中的每一个加入-6μC的电荷时，它们之间的静电力是多少？

解决方案：

问题 2：确定在空气中相隔 1m 的两个大小为 2 C 和 -1 C 的电荷之间的静电力。

解决方案：

问题3：接触的两个电子之间的距离等于1Å。确定它们之间的库仑力。

解决方案：

问题 4：当保持一定距离时，两个具有相似半径并带有相等电荷的球形导体 B 和 C 在力 F 的作用下相互排斥。第三个与 B 具有相同半径但不带电荷的球形导体被带入与 B 接触，然后与 C 接触，最终从两者中移除。 B 和 C 之间的新排斥力是多少？

解决方案：

问题 5：考虑一个由 2 × 10-7 C 和 4.5 × 10-7 C 两个电荷组成的系统，它们受到 0.1 N 的力的作用。这两个电荷之间的距离是多少？

解决方案：

问题 6：确定两个相同电荷的大小，当这两个相同电荷之间的静电力为 1000 N 且相距 0.1 m 时。

解决方案：

问题 7：考虑两个具有相同大小的相反电荷，彼此相距一定距离，使得 FN 的力作用在这两个电荷之间。如果 60% 的费用从一个转移到另一个。确定在这种情况下力值的变化幅度。

解决方案：

问题 8：特定电荷 Q 分为两个分量 q 和 Qq。如果两个部分被 r 隔开并且具有最大的库仑斥力，Q 和 q 之间的关系是什么？

解决方案：