库仑定律
当我们的合成服装或毛衣从我们身上脱下时,尤其是在干燥的天气里,会出现火花或噼啪声。对于像涤纶纱丽这样的女性服装,这几乎是不可避免的。雷暴期间天空中的闪电是另一种放电情况。从我们的座位上滑出后,打开车门或抓住公共汽车的铁条时,总是会感到触电。
这些感觉的原因是由于摩擦绝缘表面而收集的电荷的放电。这是由于静电产生。任何不随时间运动或变化的东西都称为静态的。对来自静电荷的力、场和电势的研究称为静电学。
现在让我们将静电的基本和重要定律之一称为库仑定律理解为:
库仑定律
库仑定律是描述两个点电荷之间的力的数学公式。当带电体的尺寸远小于它们之间的间距时,则不考虑或忽略尺寸。带电体可以被认为是点电荷。
The force of attraction or repulsion between two charged things is directly proportional to the product of their charges and inversely proportional to the square of the distance between them, according to Coulomb’s law. It acts along the line that connects the two charges that are regarded to be point charges.
库仑研究了两个点电荷之间的力,发现它与它们之间距离的平方成反比,与它们大小的乘积成正比,并且作用在连接它们的直线上。
库仑定律公式
The amount of the force (F) between two point charges q1 and q2 separated by a distance r in a vacuum is given by,
F ∝ q1q2
and
F ∝ 1/r2
or
F ∝ q1q2 / r2
or
F = k q1q2 / r2
where k is proportionality constant and equals to 1/4πε0.
The symbol ε0 is called epsilon not, and it signifies permittivity of a vacuum.
The value of k is 9 × 109 Nm2/ C2 when we take the S.I unit of value of ε0 is 8.854 × 10-12 C2 N-1 m-2.
向量形式的库仑定律
库仑定律最好用矢量符号表示,因为力是矢量。电荷q 1和q 2分别具有位置向量r 1和r 2 。 F 12表示由于q 2而作用在q 1上的力,而F 21表示由于q 1而作用在q 2上的力。为方便起见,两点电荷q 1和q 2已分别编号为1 和2,从1 到2 的向量已指定为r 21 。
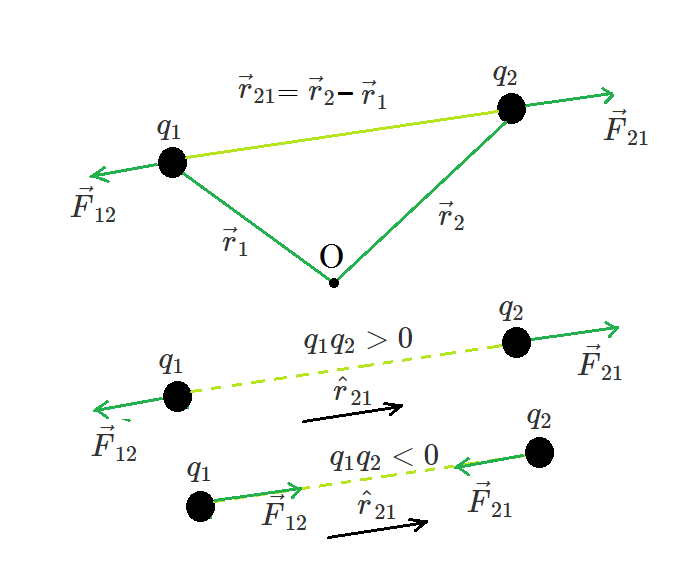
电荷之间的几何形状和力。
![]()
Similarly, the vector leading from 2 to 1 is denoted by r12,
![]()
r21 and r12 are the magnitudes of the vectors ![]() and
and ![]() , respectively and magnitude r12 is equal to r21. A unit vector along the vector specifies the vector’s direction. The unit vectors are used to denote the direction from 1 to 2 (or 2 to 1). The unit vectors define as,
, respectively and magnitude r12 is equal to r21. A unit vector along the vector specifies the vector’s direction. The unit vectors are used to denote the direction from 1 to 2 (or 2 to 1). The unit vectors define as,
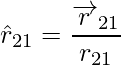
Similarly,
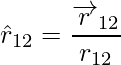
Coulomb’s force law between two point charges q1 and q2 located at vector r1 and r2 is then expressed as,
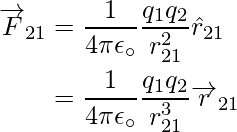
库仑定律的要点:
- 无论q 1和q 2是正还是负,上述表达式都成立。 F 21朝向
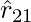 ,如果 q 1和 q 2具有相同的符号(要么都是正的,要么都是负的),它应该是排斥力。当q 1和q 2的符号相反或不喜欢电荷时,F 21朝向
,如果 q 1和 q 2具有相同的符号(要么都是正的,要么都是负的),它应该是排斥力。当q 1和q 2的符号相反或不喜欢电荷时,F 21朝向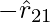 , 即朝向
, 即朝向 正如预期的不同收费一样,这显示了吸引力。因此,我们不需要为相似电荷和不同电荷构建单独的方程。上述库仑力定律的表达式都正确处理了这两种情况。
正如预期的不同收费一样,这显示了吸引力。因此,我们不需要为相似电荷和不同电荷构建单独的方程。上述库仑力定律的表达式都正确处理了这两种情况。 - 库仑力定律的上述表达式可用于计算电荷 q 1上由电荷 q 2产生的力 F 12 ,只需将 1 和 2 交换为,
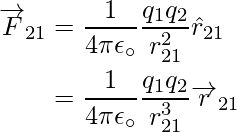
库仑定律因此与牛顿第三定律一致。
- 在真空中,库仑定律表达式决定了两个电荷 q 1和 q 2之间的力。如果电荷沉积在物质中或中间区域有物质,则由于存在带电物质成分,情况会变得更加复杂。
- 带电荷q 1和q 2的两个相同导体接触并随后分离,导致每个导体具有等于(q 1 +q 2 )/2 的电荷。如果电荷为 q 1和 –q 2 ,则每个电荷将等于 (q 1 -q 2 )/2。
多个电荷之间的力
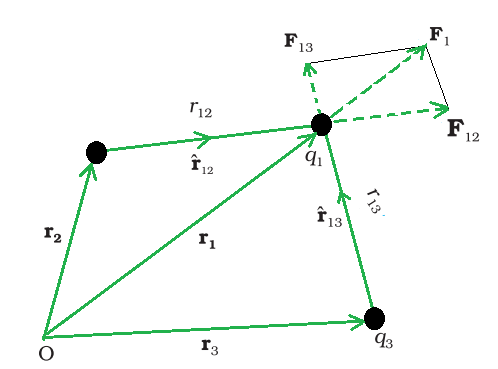
三费制度。
考虑一个真空系统,n 静止不动,电荷为 q 1 、q 2和 q 3 。实验证明,一个电荷上由于许多其他电荷而产生的所有力的矢量和,一次取一个,是该电荷上由于其他电荷而产生的所有力的矢量和。由于存在其他费用,单独的部队不受影响。这被称为叠加原理。
一个电荷上的力,比如说q 1 ,由于另外两个电荷q 2和q 3 ,可以通过对这些电荷中的每一个电荷的力进行矢量相加来确定。因此,如果 F 12表示由于 q 2 而施加在 q 1上的力,

类似地,F 13表示由于 q 3 而施加在 q 1上的力,即使存在其他电荷 q 2 ,这也是由于 q 3对 q 1的库仑力。
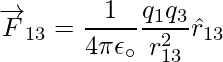
因此,由于两个电荷 q 2和 q 3对 q 1的总力 F 1可以表示为:
![由 QuickLaTeX.com 渲染 \begin{aligned}\overrightarrow{F}_{1}&=\overrightarrow{F}_{12}+\overrightarrow{F}_{13}\\&=\dfrac{1}{4\pi{\epsilon}_\circ}\dfrac{q_1q_2}{{r}_{12}^2}\hat{r}_{12}+\dfrac{1}{4\pi{\epsilon}_\circ}\dfrac{q_1q_3}{{r}_{13}^2}\hat{r}_{13}\\&=\dfrac{q_1}{4\pi{\epsilon}_\circ}\left[\dfrac{q_2}{{r}_{12}^2}\hat{r}_{12}+\dfrac{q_3}{{r}_{13}^2}\hat{r}_{13}\right]\end{aligned}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Coulomb%E2%80%99s_Law_15.jpg)
多重收费系统
上述力计算可以应用于具有三个以上电荷的系统。叠加原理表明,在电荷系统中,q 1 ,q 2 ……。 q n ,由于q 2对q 1的作用力与库仑定律相同,即不受其他电荷q 3 、q 4 、…、q n存在的影响。由于所有其他电荷,电荷 q 1上的力 F 12 ,F 13 ,…,F 1n的矢量和给出了总力 F 1可以写为
![由 QuickLaTeX.com 渲染 \overrightarrow{F}_{1}=\overrightarrow{F}_{12}+\overrightarrow{F}_{13}+....+\overrightarrow{F}_{1n}\\ \overrightarrow{F}_{1}=\frac{q_1}{4\pi{\epsilon}_\circ}\left[\frac{q_2}{{r}_{12}^2}\hat{r}_{12}+\frac{q_3}{{r}_{13}^2}\hat{r}_{13}+....+\frac{q_n}{{r}_{1n}^2}\hat{r}_{1n}\right]\\ \overrightarrow{F}_{1}=\frac{q_1}{4\pi{\epsilon}_\circ}\sum_{i=2}^n\frac{q_i}{{r}_{1i}^2}\hat{r}_{1i}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Coulomb%E2%80%99s_Law_17.jpg)
利用向量加法的平行四边形定律计算向量和。库仑定律和叠加原理是静电学的基础。
库仑定律的局限性
- 法律仅涵盖静止的点收费。
- 库仑定律仅适用于遵循平方反比定律的情况。
- 当电荷为任意形状时,很难应用库仑定律,因为它们之间的距离无法确定。
- 较大行星上的电荷不能直接使用该定律计算。
库仑定律的应用
- 计算两个电荷之间的距离以及它们之间的力。
- 库仑定律可用于计算电场:
E = F / q T
其中 E 是电场,F 是力,q T是测试电荷。
其国际单位制为NC -1 。
示例问题
问题 1:每个 100 微库仑大小的电荷位于真空中的等边三角形的角 A、B 和 C,每边长 4 米。如果 A 和 C 处的电荷为正,B 处的电荷为负,C 处的电荷合力的大小和方向是多少?
解决方案:
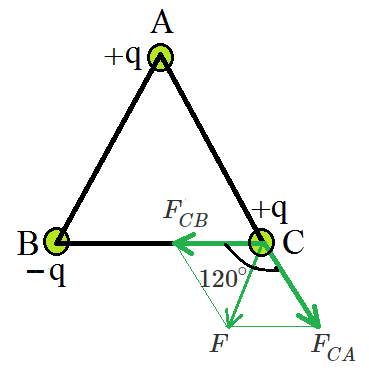
The Force FCA is applied toward AC and the expression for the FCA is expressed as

Substitute the values in the above expression,
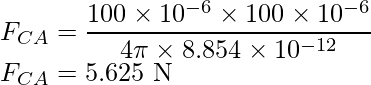
The Force FCB is applied toward CB and the expression for the FCB is expressed as
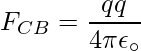
Substitute the values in the above expression,
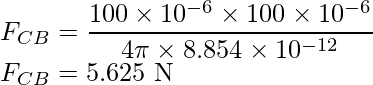
Therefore, the two forces are equal in magnitude but in different directions. The angle between them is 120º. The resultant force F is given by,

问题2:一个6×10 -6 C的正电荷距离第二个4×10 -6 C的正电荷0.040m。计算电荷之间的作用力。
解决方案:
Given,
A positive charge q1 is 6×10-6 C.
The second positive charge q2 is 4×10-6 C.
The distance between the charges r is 0.040 m.
![]()
Substitute the values in the above expression,
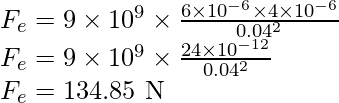
问题3:状态库仑定律及其表达式。
解决方案:
Coulomb’s law is a mathematical formula that describes the force between two point charges. When the size of charged bodies is substantially smaller than the separation between them, then the size is not considered or can be ignored. The charged bodies can be considered as point charges. Coulomb studied the force between two point charges and found that it is inversely proportional to the square of the distance between them, directly proportional to the product of their magnitudes, and acting in a line that connects them.
The amount of the force (F) between two point charges q1 and q2 separated by a distance r in a vacuum is given by
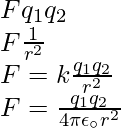
Where F is the force between two point charges, q1 and q2 are the point charge, r is the distance between the point charge and k is proportionality constant.
For subsequent simplicity, the constant k in the above expression is commonly written as
![]()
Here, is known as the permittivity of free space. The value of
![]() in SI units is
in SI units is ![]()
问题 4:为什么库仑力只在两个电荷之间作用在它们中心的连线上?
解决方案:
Because of the fundamental features of electrical charge, this is the case. Charges that are similar repel each other. Charges that are diametrically opposed attract each other.
The force of attraction or repulsion between two charges will be directed in the direction so that the force does the least amount of work. As a result of this requirement, the action is directed along the straight line connecting the two charges, which is the shortest distance between them.
问题 5:比较静电力和重力的性质。
解决方案:
Between two huge masses, a gravitational force acts. However, an electrostatic force is activated when two charged bodies come into contact.
Similarities:
- These two forces are central forces.
- Follow the law of inverse squares.
- They’re both long-range forces.
- Both forces are naturally conservative.
Dissimilarities:
- In nature, electrostatic force can be both attractive and repellent. In nature, gravitational force can only be attractive.
- The material medium between two charges affects the electric force between them. The material medium between huge bodies has little effect on gravitational force.
- Electric forces are extremely powerful (approximately 10 38 times stronger) than gravitational forces.