弹丸运动

平面内的运动也称为二维运动。例如圆周运动、抛射运动等。这种运动分析的参考点将是一个原点和两个坐标轴 X 和 Y。在本节中，我们将讨论抛射体运动。

物体抛向或抛向空中的运动，仅受重力加速度的影响，称为抛射运动。该物体称为弹丸，其路径称为其轨迹。如一维运动学问题解决基础中所述，下落物体运动是一种简单的一维类型的抛丸运动，没有水平运动。在本节中，我们将研究二维弹丸运动，例如足球或其他空气阻力可忽略不计的物体的运动。

这里要记住的最重要的事情是，沿垂直轴的运动相互独立，因此可以单独分析。这在二维运动学：简介中进行了讨论，其中证明了垂直和水平运动是独立的。理解二维弹丸运动的关键是将其分为两种运动，一种是水平的，一种是垂直的。（这是最合乎逻辑的轴选择，因为重力加速度是垂直的，所以当空气阻力可以忽略不计时，沿水平轴不会有加速度。）

当然，为了描述运动，我们必须考虑速度、加速度和位移。我们还必须沿 x 轴和 y 轴定位它们的分量。我们将假设除重力之外的所有力（例如空气阻力和摩擦力）都可以忽略不计。加速度的组成部分非常简单：

a _y = –9.80 m/s ² 。（请记住，此定义假设向上定义为正方向，如果坐标系以向下为正的方式排列，则重力加速度为正。）
a _x = 0 因为重力是垂直的。

因为加速度是恒定的，所以可以应用运动学方程。

弹丸运动

抛射物是任何被抛入太空的物体，只有重力作用于它。作用在弹丸上的主要力是重力。这并不是说其他力量不作用于它；相反，与重力相比，它们的影响微乎其微。轨迹是弹丸所走的路径。

When a particle is thrown obliquely near the earth’s surface, it follows a curved path with constant acceleration toward the earth’s center (we assume that the particle remains close to the surface of the earth). The path of such a particle is known as a projectile path, and its motion is known as projectile motion.

编程需要懂一点英语

抛射运动是平面中最常见的运动类型之一。弹丸运动中唯一的加速度是重力引起的垂直加速度 (g)。因此，可以在 X 轴和 Y 轴中分别使用运动方程来确定未知参数。

The motion of a projectile in two dimensions is divided into two parts:

Horizontal motion in the x-direction with no acceleration and
Vertical motion in the y-direction with constant acceleration due to gravity.

The equation for projectile motion is y = ax + bx².
To simplify calculations, projectile motion is typically calculated without accounting for air resistance.

编程需要懂一点英语

在了解弹丸运动关系的推导之前，让我们先介绍一些其中使用的术语，即：

投影角：物体相对于水平面投影的角度称为投影角。
投射速度：身体被抛出的速度称为投射速度。
投影点：投影点是身体在空中投影的点。
弹丸弹道：弹丸在空中所走的路径称为弹丸的弹道。
水平射程：弹体运动所经过的水平距离称为弹体的射程。

考虑以下示例，该球以初始速度 u 从点 O 相对于水平 x 轴以角度 θ 投影：

这里，

O点称为投影点。
θ 是投影角度和
OB = 水平范围。
H是粒子的高度。
粒子从 O 到 B 的总时间称为飞行时间。

我们可以使用运动微分方程来找到与弹丸运动相关的各种参数。

我们知道运动的线性方程是：

v = u + at

S = ut + 1/2(at²)

v² = u² + 2aS

Applying the above equation for projectile motion the equation will be:

v = u – gt

S = ut – 1/2(gt²)

v² = u² – 2gS

Where,

u = initial velocity

v = Final velocity

g = Acceleration due to gravity (Taking it -ve because gravity always work downward)

S = Displacement

t = Time

编程需要懂一点英语

总飞行时间：

Y 方向总位移 (S _y ) = 0。

因此沿 Y 方向运动，S _y = u _y t – 1/2(gt ² ) [这里，u _y = u sinθ 和 S _y = 0]

即 0 = usinθ – 1/2(gt ² )

t = 2usinθ/g

Total Time of Flight(t) = 2usinθ/g

编程需要懂一点英语

情况 1：如果 θ = 90°

从飞行时间公式可以看出，弹丸所用时间与投射角度成正比。对于任何给定的初始速度 (u) 将是恒定的，并且 g 始终是恒定的，即 g=-9.8 m/s2。

当弹丸以 90° 角投射时，飞行时间最长。

所以，t _max = 2usinθ/g = 2u/g [ sin 90° = 1]

情况 2：如果 θ = 30°

当弹丸以 30° 角投射时，飞行时间是 t _max的一半。

即 t = 2usin30°/g = t _max /2。 [ sin30° = 1/2 ]

水平范围：

水平范围是一个距离（OB），它可以给出：

OB = 速度的水平分量(u _x ) * 总时间(t) [这里，u _x = u cosθ 和 t = 2usinθ/g]

即 Range(R) = ucosθ * 2usinθ/g

因此，弹丸的水平射程由 (R) 给出：

Horizontal Range(R) = u²sin2θ/g [ Here, sin2θ = 2cosθsinθ]

编程需要懂一点英语

情况 1：如果 θ = 90°

当射弹以 90° 的角度投射时，水平范围将为零，因为射弹将击中射弹投射的同一点。

即 R = u ² sin2θ/g = 0。 [ sin 2θ = 0，在 θ = 90 处]

情况 2：如果 θ = 45°

当弹丸以 45° 水平投射时，弹丸的射程最大。

即R = u ² sin2θ/g = u ² /g。 [这里，sin90 = 1]

最大高度：

它是粒子的最高点（A点）。当球到达点 A 时，速度的垂直分量 (V _y ) 将为零。

即 0 = (usinθ) ² – 2gH _max [这里，S = H _max , v _y = 0 和 u _y = u sin θ]

因此，弹丸的最大高度由 (H _max ) 给出：

Maximum Height (H_max) = u²sin²θ/2g

编程需要懂一点英语

情况 1：如果 θ = 90°

如果我们以 90° 的角度投射弹丸，它会达到最大高度 (H _max )。

即H _max = u ² sin ² θ/2g = u ² /2g。 [这里，罪² 90 = 1]

情况 2：如果 θ = 45°

当弹丸以 45° 的角度投射时，弹丸的高度是其最大高度 (Hmax) 的一半。

即 H = u ² sin ² θ/2g = (1/2)u ² /2g = Hmax/2。 [ 罪² 45° = 1/2 ]

我们也可以说，如果弹丸角度为 45°，弹丸的水平射程将是弹丸高度的 4 倍。

即 H = u ² /4g = R/4 [这里，θ = 45°处的水平范围，R = u ² /g]

或 R = 4H。

轨迹方程：

轨迹方程是弹丸运动过程中粒子所遵循的路径。方程是：

y = x tanθ – gx²/2u²cos²θ

编程需要懂一点英语

这是抛物运动方程，它类似于抛物线（y = ax + bx ² ），所以我们可以说抛物运动本质上总是抛物线。

二维运动的例子如下，

投掷球或发射炮弹
台球在台球桌上的运动。
发射炮弹的运动。
地球绕太阳公转。
当一个球从移动的汽车上抛出时，球所经过的路径是抛射运动。
在板球比赛中，守场员将球扔向三柱门。
一颗子弹射向远距离目标。

抛射运动的一些应用：

火箭或导弹是现代生活中更复杂的射弹应用类型。
运动员通常使用抛射物，尤其是在标枪、铅球、铁饼和锤子投掷等方面。
射箭和射击也使用射弹。

示例问题

问题一：什么是弹丸？证明弹丸的路径是抛物线的。

解决方案：

Projectile: A projectile is any object thrown into space with only gravity acting on it.

We know that the equation of projectile is, y = x tan θ – gx²/2u²cos²θ comparing the equation with y = ax + bx²

Here, a = tanθ, and b = – g/2u²cos²θ.

The above equation of trajectory is similar to a parabola. So it is proved that the path of a projectile is parabolic.

编程需要懂一点英语

问题 2：应该以什么角度投射弹丸，以使弹丸的高度和射程相等？

解决方案：

If height and horizontal range will be equal than, H = R.

i.e.

u²sin²θ/2g = u²sin2θ/g

sin²θ = sin2θ [Here, sin2θ = 2 sinθ cosθ and tanθ = sinθ/cosθ]

tanθ = 4

So,

θ = tan^-1(4)

编程需要懂一点英语

问题 3：定义水平射程并找到以 98 m/s 的速度与水平面成 30 度角的射弹的射程？ (g =9.8 m/s ² )

解决方案：

Horizontal Range- The horizontal distance traveled by the body performing projectile motion is referred to as the range of the projectile.

Horizontal Range, R = u²sin2θ/g

= (98)² × (60°) / 9.8

= 490√3 m

编程需要懂一点英语

问题4：说出弹丸运动过程中保持不变的物理量？

解决方案：

Velocity, vertical component of velocity momentum, kinetic energy, and potential energy remain unchanged during the projectile motion.

编程需要懂一点英语

问题 5：质量为 100 g 的球以 30° 角从地面以 11 m/s 的初速度和 g = 10 m/s ²的重力加速度投射到的最大高度是多少？

解决方案：

We know that the formula for maximum height is,

H = (usinθ)²/2g.

Given,

u = 11 m/s, θ = 30°, g = 10 m/s²

Hence,

Putting the values we get,

H = 1.5125 m

编程需要懂一点英语

问题6：足球以10 m/s的初速度与地面成45°角发射；重力加速度为 g = 10 m/s ² 。飞行时间是几点？

解决方案：

We know that the formula for calculating the time of flight is,

t = 2(usinθ/g)

Given, θ = 45°, u = 10m/s, g = 10m/s²

Putting the values we get,

t = 1.4142 s

编程需要懂一点英语

问题 7：从 O 点以 30° 的角度以 30 m/s 的初速度投射弹丸。弹丸在 M 点撞击地面。（考虑重力加速度 g = 10m/s ² ）找到以下内容：

总飞行时间是多少？
弹丸的水平射程 (OM) 是多少？
弹丸的最大高度是多少？

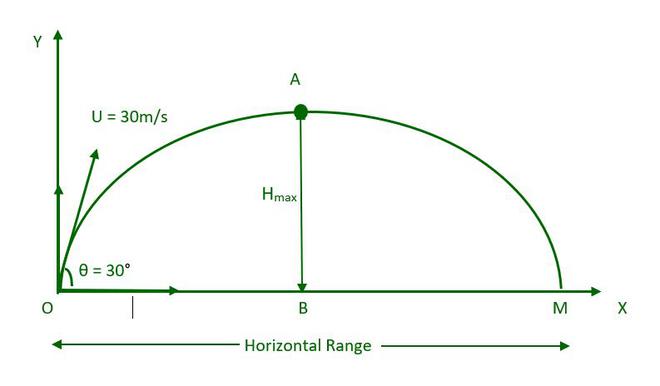
解决方案：

Given,

Initial velocity u = 30m/s.

The angle of projection, θ = 30°.

1. Total time of flight

We know that the total time of flight by the projectile is given by-

t = 2usinθ/g

Putting the given values,

t = 2 × 30 sin30°/10

= 3 s

2. Horizontal Range

We know the formula for horizontal range is:

R = u²sin2θ/g.

Putting the values we get,

R = (30)² sin60° /10

= 45 √3 m.

3. Maximum Height

Maximum height of the projectile is given by the formula:

H_max = u²sin²θ/2g

Putting the values we get,

H_max = (30)²sin²30°/2 × 10

= 11.25 m.

编程需要懂一点英语