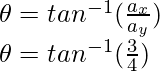二维运动

可以使用坐标来描述在平面中运动的粒子。但通常这些信息不足以完全描述粒子的状态和行为。还有一些问题没有得到解答。例如，它移动的速度有多快？粒子的加速度和加速度的方向是哪个方向？这些参数对于描述粒子在 2D 平面中的运动是必要的。幸运的是，所有这些都可以使用一点向量代数和微积分来计算。让我们详细看看如何做到这一点。

平面运动

假设一个粒子从笛卡尔平面上的 X 点移动到 Y 点。位置向量是描述粒子当前位置所必需的。这些向量总是相对于原点的参考系。需要以下参数来完整描述粒子在平面中移动的行为，

1. 位置
2. 速度
3. 加速

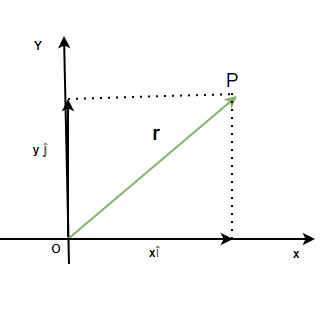
位置向量

表示粒子位置相对于原点的位置和方向的向量称为位置向量。位置向量$\vec{r}$因为一个粒子由下式给出，

$\vec{r} = x\hat{i} + y\hat{j}$

其中 x 和 y 是它们沿 x 和 y 轴的分量。

速度

粒子的速度可以用两种方式描述——平均速度和瞬时速度。当粒子处于加速状态时，它每秒都会改变它的速度。因此，不能将单个值分配给速度。在这种情况下，瞬时速度是首选，它描述了特定时刻的速度及其方向。它是由，

$\vec{v} = \lim_{\Delta t \to 0}\frac{\Delta \vec{r}}{\Delta t}\\ = \vec{v} = \frac{dr}{dt}$

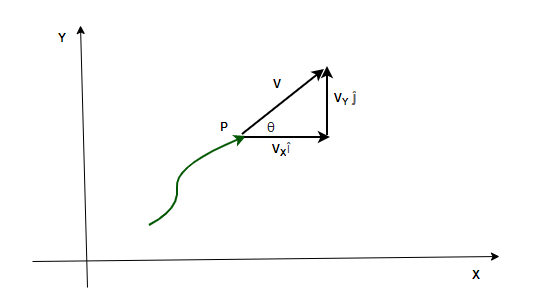
速度也可以以其分量的形式表示。

$v = \frac{dx}{dt}\hat{i} + \frac{dy}{dt}\hat{j}$

平均速度是总位移与总时间之比。假设一个粒子从$\vec{r}$到$\vec{r'}$在总时间$\Delta t$

速度由下式给出，

$\vec{v} = \frac{\vec{r} - \vec{r'}}{\Delta t}$

加速

物体在平面上运动的加速度由其速度的变化率给出。与速度类似，这里也有两种情况——平均加速度和瞬时加速度。平均加速度由物体速度的净变化与所用总时间的比率给出。让初始速度和最终速度表示为$\vec{v}_{i}$和$\vec{v_{f}}$

$\vec{v} = \frac{\vec{v_{f}} - \vec{v_{i}}}{\Delta t}$

当身体的加速度随时间变化时使用瞬时加速度。

$\vec{a} = \lim_{\Delta t \to 0}\frac{\Delta \vec{v}}{\Delta t}\\ = \vec{a} = \frac{dv}{dt}$

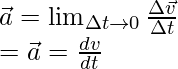
这也可以分解成它的组件。

$a = \frac{dv_{x}}{dt}\hat{i} + \frac{dv_{y}}{dt}\hat{j} \\ = a = \frac{d^2x}{dt^2}\hat{i} + \frac{d^2y}{dt^2}\hat{j}$

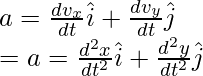
加速度的大小也可以使用速度的分量来计算，

$a = \sqrt{a_x^2 + a_y^2}$

方向由角度给出$\theta$ ,

$\theta = tan^{-1}(\frac{a_y}{a_x})$

示例问题

问题 1：求 t = 2 处的速度，对于在平面上运动的粒子，其位置如下所示，

r = t ² i + t ² j

回答：

Given: the initial and final position vectors, 

r = t²i + t²j

The position vector changes with time. The velocity in this case is given by the formula, 

$v = \frac{dx}{dt}\hat{i} + \frac{dy}{dt}\hat{j}$

Here x(t) = t² and y(t) = t²

Plugging these values into the equation, 

$v = \frac{dx}{dt}\hat{i} + \frac{dy}{dt}\hat{j} \\ v= \frac{d}{dt}(t^2)\hat{i} + \frac{d}{dt}(t^2)\hat{j} \\ v = 2t \hat{i} + 2t\hat{j}$

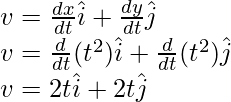
At t = 2, 

$v = 2t \hat{i} + 2t\hat{j} \\ = v = 2(2)\hat{i} + 2(2)\hat{j} \\ = v = 4\hat{i} + 4\hat{j}$

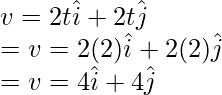
问题 2：求 t = 0 时的速度，对于在平面上运动的粒子，其位置如下所示，

r = (t+2)i + (4t ² +2)j

回答：

Given: the initial and final position vectors, 

r = (t+2)i + (4t²+2)j

The position vector changes with time. The velocity in this case is given by the formula, 

$v = \frac{dx}{dt}\hat{i} + \frac{dy}{dt}\hat{j}$

Here x(t) = t+2 and y(t) = 4t²+2

Plugging these values into the equation, 

$v = \frac{dx}{dt}\hat{i} + \frac{dy}{dt}\hat{j} \\ v= \frac{d}{dt}(t+2)\hat{i} + \frac{d}{dt}(4t^2+2)\hat{j} \\ v = \hat{i} + 8t\hat{j}$

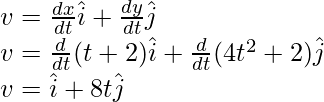
at t = 0, 

$v = \hat{i} + 8t\hat{j} \\ = v = 4\hat{i} + 8(0)\hat{j} \\ = v = 4\hat{i}$

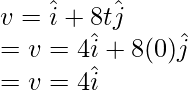
问题 3：求 t = 0 和 t = 3 之间的平均加速度，对于在平面上运动的粒子，其位置如下所示，

v = 3ti + 3t ³ j

回答：

Given: velocity as a function of time. 

v = 3ti + 3t³j

The velocity vector changes with time. The average acceleration is given by the formula, 

$\vec{a} = \frac{\vec{v_f} - \vec{v_i}}{\Delta t}$

At t = 0 

v_i = 0i + 0j 

At t = 3 

v_f = 9i + 81j 

$\Delta t = 3$

Plugging the values into the above equation, 

$\vec{a} = \frac{\vec{v_f} - \vec{v_i}}{\Delta t}\\ = \vec{a} = \frac{9\hat{i} + 81\hat{j}}{3} \\ = \vec{a} = 3\hat{i} + 27\hat{j}$

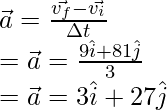
问题 4：求 t = 0 和 t = 2 之间的平均加速度，对于在平面上运动的粒子，其位置如下所示，

v = ti + 3tj

回答：

Given: velocity as a function of time. 

v = ti + 3tj

The velocity vector changes with time. The average acceleration is given by the formula, 

$\vec{a} = \frac{\vec{v_f} - \vec{v_i}}{\Delta t}$

At t = 0 

v_i = 0i + 0j 

At t = 2 

v_f = 2i + 6j 

$\Delta t = 2$

Plugging the values into the above equation, 

$\vec{a} = \frac{\vec{v_f} - \vec{v_i}}{\Delta t}\\ = \vec{a} = \frac{2\hat{i} + 6\hat{j}}{2} \\ = \vec{a} = \hat{i} + 3\hat{j}$

问题 5：求 t = 1 时的瞬时加速度，对于在平面上运动的粒子，其位置如下所示，

r = ti + tj

回答：

Given: the initial and final position vectors, 

r = ti + tj

The position vector changes with time. The acceleration in terms of position given by, 

$a = \frac{d^2x}{dt^2}\hat{i} + \frac{d^2y}{dt^2}\hat{j}$

x(t) = t and y(t) = t

Plugging the values into the equation, 

$a = \frac{d^2x}{dt^2}\hat{i} + \frac{d^2y}{dt^2}\hat{j} \\ a = \frac{d^2(t)}{dt^2}\hat{i} + \frac{d^2(t)}{dt^2}\hat{j} \\ a = 0\hat{i} + 0\hat{j}$

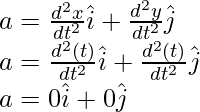
问题 6：求 t = 1 处的瞬时加速度，对于在平面上运动的粒子，其位置如下所示，

r = 3ti + t ³ j

回答：

Given: the initial and final position vectors, 

r = 3ti + t³j

The position vector changes with time. The acceleration in terms of position given by, 

$a = \frac{d^2x}{dt^2}\hat{i} + \frac{d^2y}{dt^2}\hat{j}$

x(t) = 3t and y(t) = t³

Plugging the values into the equation, 

$a = \frac{d^2x}{dt^2}\hat{i} + \frac{d^2y}{dt^2}\hat{j} \\ a = \frac{d^2(3t)}{dt^2}\hat{i} + \frac{d^2(t^3)}{dt^2}\hat{j} \\ a = 0\hat{i} + 6t\hat{j}$

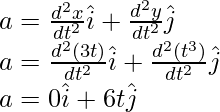
at t = 1 

a = 6j

问题 7：物体在二维平面上运动，沿 x 轴的加速度为 4m/s ² ，沿 y 轴的加速度为 3m/s ² 。求 x 轴上加速度的大小和角度。

回答：

The magnitude of acceleration can also be calculated using the components of velocity, 

$a = \sqrt{a_x^2 + a_y^2}$

a_x = 4 m/s² and a_y = 3 m/s²

Plugging the values in the equation, 

$a = \sqrt{a_x^2 + a_y^2} \\ = a = \sqrt{3^2 + 4^2} \\ = a = \sqrt{9 + 16} \\ = a = \sqrt{25} \\ = a = 5$

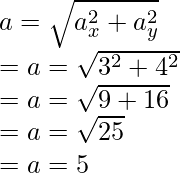
The angle can be calculated using the equation, 

$\theta = tan^{-1}(\frac{a_x}{a_y}) \\ \theta = tan^{-1}(\frac{3}{4})$