外场势能

当外力进行工作时，例如抵抗弹簧力或重力等力将物体从一个位置移动到另一个位置，该功被收集并作为势能存储在体内。当移除外力时，身体移动，获得等量的动能和失去势能。因此，总动能和势能是守恒的。保守势力是属于这个群体的人。弹簧力和重力是这些力的两个例子。

Coulomb force is a conservative force between two (stationary) charges. Both have an inverse-square relationship on distance and differ only in the proportionality constants. The masses in the expression of gravitational law are replaced by charges in Coulomb’s law expression. Thus, like the potential energy of a mass in a gravitational field, the electrostatic potential energy of a charge in an electrostatic field is defined.

外场势能

• 单次充电的势能：

电荷及其位置先前已作为电场源给出，并计算了这些电荷系统的势能。最显着的区别是，现在我们关注的是外部场中电荷（或电荷）的势能。要计算其势能的指定电荷不会产生外部场 E。E 是由所提供电荷之外的源产生的。可以识别外部来源，但是，它们通常是未知的或未指定的。

假设电荷 q 不影响产生外场的源。如果 q 非常小，或者如果未指定的力将外部源保持在适当位置，则这是正确的。即使 q 是有限的，它对外部源的影响也可能被忽略，因为在无穷远处的极强源在感兴趣的区域中产生有限场 E。

从点到点，外部电场 E 和相关的外部电势 V 可能不同。点 P 处的 V 定义为将单位正电荷从无穷远处传递到点 P 所需的功。因此，在外场中将电荷 q 从无穷远处传递到点 P 所需的功为 qV。

该功以 q 的势能形式存储。如果点 P 具有相对于某个原点的位置向量 r，则可以写为，

q 在外场中 r 处的势能 = qV(r)

其中 V(r) 是 r 点的外部电势。

因此，如果一个电荷为 q = e = 1.6×10 ^–19 C 的电子被 ΔV = 1 伏特的电势差加速，它将获得 qΔV = 1.6 × 10 ^–19 J 的能量。

该能量单位定义为 1 电子伏特或 1eV，

即 1 eV=1.6 × 10 ^–19 J。

• 两个电荷系统在外场中的势能：

两个电荷 q ₁和 q ₂分别位于 r ₁和 r ₂的系统在外场中的势能。首先，计算将电荷 q1 从无限变为 r1 所需的工作量。 q ₁ V(r ₁ ) 是这一步所做的工作。之后，将 q ₂变为 r ₂的工作。在这个阶段对外部场 E 和 q ₁创建的场做功。

q ₂对外部场所做的功 = q ₂ V(r2 )…………………………………………..(1)

由于 q ₁在 q ₂上对场所做的功等于 (q ₁ q ₂ /4πε _o r ₁₂ ) ………………………….(2)

其中 r12 是 q ₁和 q ₂之间的距离。通过场的叠加原理，将 q ₂上所做的功与两个场（E 和由于 q ₁的功）相加（等式（1）和等式（2））。

将 q ₂带入 r ₂所做的功等于 q ₂ V(r ₂ ) + (q ₁ q ₂ /4πε _o r ₁₂ )

因此，系统的势能 = 组装配置完成的总功

= q ₁ V(r ₁ ) + q ₂ V(r ₂ ) + (q ₁ q ₂ /4πε _o r ₁₂ ) ……………………………………….. (3)

• 偶极子在外场中的势能

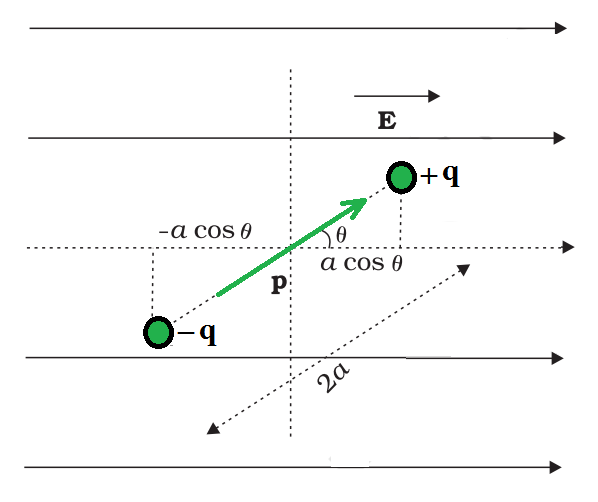
偶极子在均匀外场中的势能。

假设电荷为 q1 = +q 和 q2 = –q 的偶极子置于均匀电场 E 中。偶极子在均匀电场中感受不到净力，但确实经历了定义为

τ = p × E。

这将倾向于旋转它。假设以这样一种方式施加外部扭矩τ _ext ，它简单地抵消扭矩并在纸平面内以极小的角速度旋转它，并且没有从角度θ ₀到角度θ ₁的角加速度。外力矩所做的功可以写成：

$W=\int_{\theta_0}^{\theta_0} \tau_{ext}(\theta)d\theta\\ W=\int_{\theta_0}^{\theta_0}pE\sin\theta{d\theta}\\ W=pE(\cos\theta_0-\cos\theta_1)$

这项工作被保存为系统的势能。然后可以将势能 U(θ) 与偶极子的倾角 θ 联系起来。与其他势能一样，选择将势能 U 视为零的角度有一定的自由度。取 θ ₀ = π/ 2 是一个自然的决定。

$U(\theta)=pE(\cos\frac{\pi}{2}-\cos\theta)\\ U(\theta)=pE\cos\theta\\ U(\theta)=-p\cdot{E}$

该表达式也可以从等式(3)中理解。将等式 (3) 应用于当前的两个电荷 +q 和 -q 的系统。

$U'(\theta)=q\left[V(r_1)-V(r_2)\right]-\frac{q^2}{4\pi\epsilon_0\times2a}$ ……(4)

+q 和–q 的位置向量由r ₁和r ₂表示。将单位正电荷对电场从 r ₂传输到 r ₁所做的功现在等于位置 r ₁和 r ₂之间的电位差。 2a×cosθ 是平行于力的位移。

因此，[V(r ₁ ) – V(r ₂ )] = -E×2a×cosθ

因此，等式（4）可以写成，

$U'(\theta)=-pE\cos\theta-\frac{q^2}{4\pi\epsilon_0\times2a}\\ U'(\theta)=p\cdot{E}-\frac{q^2}{4\pi\epsilon_0\times2a}$

Note that, U′(θ) differs from U(θ) by a quantity which is just a constant for a given dipole.

静电势

一般考虑任何静电荷设置。测试电荷 q 的势能是根据对它所做的功来定义的。因为任何地方的力都是 qE，其中 E 是由于给定的电荷排列而导致的该点的电场，所以这项功显然与 q 成正比。结果，很方便将功除以电荷量 q，得到一个与 q 无关的量。换句话说，每单位测试电荷所做的功代表了与电荷配置相关的电场。由于给定的电荷配置，这达到了静电势 V 的概念。外力从 R 点到 P 点携带单位正电荷所做的功可以表示为：

$W=V_P-V_R\\ W= \frac{U_P-U_R}{q}$

其中，VP 和 VR 分别是 P 和 R 处的静电势。

“The work done by an external force to carry a unit positive charge from infinity to a location is equal to the electrostatic potential (V) at that point.”

换句话说，具有静电场的区域中任何位置的静电势 (V) 是将单位正电荷从无穷远处传输到该位置（没有加速）所需的功。电势能是一个标量，没有方向，只有大小。它用 V 表示，具有维数公式 ML ² T ^-3 A ^-1 。

示例问题

问题 1：推导出在均匀电场中将电偶极子旋转角度 θ 所做的总功的表达式？

解决方案：

The expression for the torque can be written as,

  T = PE sinθ

If an electric dipole is rotated through an angled against the torque acting on it, then small amount of work done is

dw =Tdθ = PE sinθ dθ

For rotating through on angle θ, from 90^o,

$w=\int_{90^o}^{\theta} PE\sin\theta{d\theta}\\ w=PE[-\cos\theta]_{90^o}^{\theta}\\ w=-PE\cos\theta$

问题 2：由分别放置在 (–9 cm, 0, 0) 和 (9 cm, 0, 0) 的两个电荷 8 µC 和 –6 µC（并且没有外场）组成的系统。确定将两个电荷无限地分开需要多少功？

解决方案：

Given,

The value of two charges are 8 µC and –6 µC.

Distance between them is 0.18 m.

The expression for the potential energy of a system of two charges q₁ and q₂ can be written as,

$U=\frac{1}{4\pi\epsilon_0}\frac{q_1q_2}{r}$

Substitute the value in the above expression,

$U=9\times10^9\times\frac{8\times(-6)\times10^{-12}}{0.18}\\ U=-2.4\text{ J}$

W = U₂ – U₁ 

W = 0 – U 

W = 0 – (–2.4) 

W =2.4 J.

问题 3：两个半径分别为 a 和 b 的带电导电球通过导线相互连接。两个球体表面的电场比是多少？使用获得的结果来解释为什么导体的尖端和尖端的电荷密度高于其平坦部分的电荷密度。

解决方案：

Let a be the radius of a sphere A, QA be the charge on the sphere, and CA be the capacitance of the sphere. Let b be the radius of a sphere B, QB be the charge on the sphere, and CB be the capacitance of the sphere. Since the two spheres are connected with a wire, their potential (V) will become equal.

Let EAbe the electric field of sphere A and EB be the electric field of sphere B. Therefore, their ratio,

$\frac{E_A}{E_B}=\frac{Q_A}{4\pi\ \epsilon_o\times{a^2}}\times\frac{4\pi\ \epsilon_o\times{b^2}}{Q_B}\\ \frac{E_A}{E_B}=\frac{Q_A}{Q_B}\times\frac{b^2}{a^2}...........(1)$

Since, \frac{Q_A}{Q_B}=\frac{C_AV}{C_BV} and $\frac{C_A}{C_A}=\frac{a}{b}$  

There fore the equation (1) can be expressed as,

$\frac{E_A}{E_B}=\frac{b}{a}$ 

问题 4：假设极板间距为 0.5 cm，2 F 平行板电容器的极板面积是多少？ [您将从您的回答中意识到为什么普通电容器在 µF 或更小的范围内。但是，由于导体之间的间隔非常小，电解电容器确实具有更大的电容（0.1 F）。]

解决方案：

Given,

Capacitance of a parallel capacitor, V = 2 F.

Distance between the two plates, d = 0.5 cm = 0.5×10^-2 m.

Capacitance of a parallel plate capacitor is given by the relation,

C = ε_oA/d

Where,

 ε_o = Permittivity of free space = 8.85×10^-12 C²N^-1m^-2.

A= (2×0.5×10^-2)/8.85×10^-12

=1130 km²

Hence, the area of the plates is too large. To avoid this situation, the capacitance is taken in the range of µF.

问题 5：将测试电荷 q 沿电偶极子的赤道轴移动 1 厘米的距离所做的功是多少？

解决方案：

Since potential for equatorial axis is  zero that is

V = 0

Therefore, 

W = qV = 0

问题 6：定义外部电场中距离 V 处的“电荷势能 q”。

解决方案：

It is defined as the amount of work done in bringing the charge from infinity to its position in the system in the electric field of another charge without acceleration.

V = Er.

问题 7：一个半径为 5 cm 的空心金属球带电，其表面的电势为 10 V。球心的电势是多少？

解决方案：

The electric field inside the shell is zero. This implies that potential is constant inside the shell (as no work is done in moving a charge inside the shell) and, therefore, equals its value at the surface, which is 10 V.