电荷系统的势能

当外力工作以完成工作时，例如将物体从一个位置移动到另一个位置以抵抗诸如弹簧力或重力之类的力，该功被收集并存储为身体的势能。当外力被移除时，身体移动，获得动能并失去相应量的势能。结果，保留了总动能和势能。保守势力就是这种类型的势力。弹簧力和重力是这些力的两个例子。

The Coulomb force is a conservative force that exists between two (stationary) charges. Both have an inverse-square relationship with respect to distance, with the only difference being the proportionality constants. The masses in the formulation of gravitational law are substituted by charges in the expression of Coulomb’s law. Thus, the electrostatic potential energy of a charge in an electrostatic field is defined in the same way as the gravitational potential energy of a mass in a gravitational field is.

什么是静电势？

The work done by an external force to carry a unit positive charge from infinity to a location is equal to the electrostatic potential (V) at that point is called the Electrostatic Potential.

电势能是一个标量，没有方向，只有大小。

它用 V 表示，具有维度公式 [ML ² T ^-3 A ^-1 ]。

点电荷产生的电势

考虑点电荷 Q 的起源。取 Q 为正。使用从原点开始的位置矢量 r，我们想要找到任意点 P 的电势。为此，我们必须计算将一个单位正测试电荷从无穷远传输到点 P 所需的工作量。当 Q > 0 时，对排斥力的测试电荷所做的工作是正的。我们选择一条方便的路径——沿着从无穷远到 P 点的径向——因为完成的工作与路径无关。

将一个单位正测试电荷从无穷远处带到 P 点，抵抗电荷排斥力 Q (Q > 0) 所做的功是电荷 Q 在 P 处的电势。

在路径上某个中间点 P' 处单位正电荷上的静电力等于

$\frac{Q\times1}{4\pi\epsilon_0r'^2}\hat{r'}$

在哪里$\hat{r'}$是沿 OP' 的单位向量，因此，从 r' 到 r' + Δr' 对这个力所做的功可以写成

$\Delta{W}=-\frac{Q}{4\pi\epsilon_0r'^2}\Delta{r'}$

负号表示 Δr′ < 0，ΔW 为正。外力完成的总功 (W) 由上述方程两边积分确定，从 r' = ∞ 到 r' = r，

$W=-\int_{∞}^{r} \frac{Q}{4\pi\epsilon_0r'^2}d{r'}\\ W=\left[\frac{Q}{4\pi\epsilon_0r'}\right]_∞^r\\ W=\frac{Q}{4\pi\epsilon_0r}$

由于电荷 Q 在 P 处的电位可以表示为

$V(r)=\frac{Q}{4\pi\epsilon_0r}$

电荷系统的势能

考虑两个电荷 q ₁和 q ₂的简单情况，位置向量 r ₁和 r ₂相对于一个点。让我们计算一下将这种安排放在一起的工作（从外部）。这意味着首先从无穷大的费用 q ₁和 q ₂开始，然后计算外部机构为将费用送到提供的目的地所做的工作量。假设电荷q ₁首先从无穷大转移到r ₁ 。因为没有必须对其进行工作的外部场，所以将 q ₁从无穷大变为 r ₁所需的工作量为零。这种电荷在空间中产生一个势能，可以写成，

$V_1=\frac{1}{4\pi\epsilon_0}\frac{q_1}{r_{1P}}$

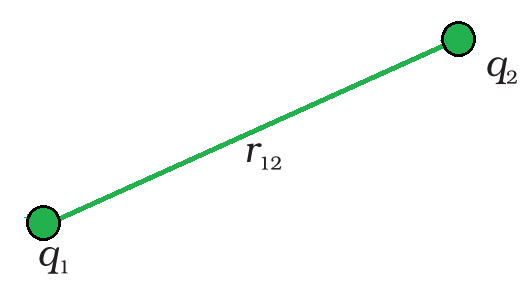
3 电荷系统 q ₁和 q ₂的势能与乘积电荷成正比，与它们之间的距离成反比。

其中 r _1P是空间中点 P 到 q ₁位置的距离。根据电势的定义，将电荷 q ₂从无穷大带到点 r2 所做的功是 q2 乘以 q ₁导致的 r2 处的电势，

$\text{work done on q}_2=\frac{1}{4\pi\epsilon_0}\frac{q_1q_2}{r_{12}}$

其中 r ₁₂是点 1 和 2 之间的距离。由于静电力是保守的，因此这项功以系统势能的形式收集。因此，具有两个电荷 q ₁和 q ₂的系统的势能可以写为：

$U=\frac{1}{4\pi\epsilon_0}\frac{q_1q_2}{r_{12}}$ ……..(1)

显然，如果先将 q ₂转移到其当前位置，然后将 q ₁带到其当前位置，则势能 U 将相同。

如果 q ₁ q ₂ > 0，势能为正。这是意料之中的，因为静电力排斥同种电荷（q ₁ q ₂ > 0），必须对它做正的努力才能得到从无穷远到相隔有限距离的电荷。静电力对不同的电荷具有吸引力（q ₁ q ₂ < 0）。要将电荷从指定点带到无穷大，需要对这个力做正的功。换句话说，反向路径（从无穷大到现在的地方）需要负的功，因此势能是负的。

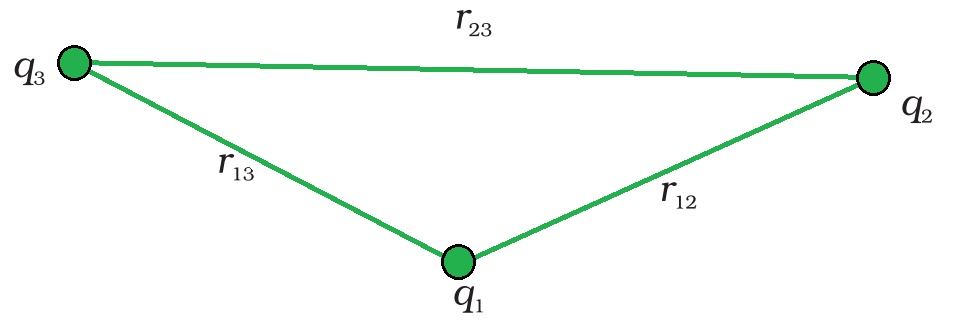
三电荷系统的势能。

方程（1）可以很容易地推广到系统中任意数量的点电荷。计算具有三个电荷 q _1、 q ₂和 q ₃的系统分别在距离 r ₁ 、r ₂和 r ₃处的势能。将 q ₁首先从无穷变为 r ₁不需要做任何工作。接下来，将 q ₂从无穷大带到 r ₂ 。如前所述，这一步完成的工作是

$q_2V_1(r_2)=\frac{1}{4\pi\epsilon_0}\frac{q_1q_2}{r_{12}}$ ……(2)

电荷 q ₁和 q ₂产生一个电势，在任何点 P 可以写为

$V_{1,2}=\frac{1}{4\pi\epsilon_0}\left(\frac{q_1}{r_{1P}}+\frac{q_2}{r_{2P}}\right)$

接下来将 q ₃从无穷大带到点 r ₃的工作是 q ₃乘以 V _1,2在 r ₃处，可以写为，

$q_3V_{1,2}(r_3)=\frac{1}{4\pi\epsilon_0}\left(\frac{q_1q_3}{r_{13}}+\frac{q_2q_3}{r_{23}}\right)$ …….(3)

通过添加等式（2）和（3）获得在给定位置收集电荷所做的总工作，

$U=\frac{1}{4\pi\epsilon_0}\left(\frac{q_1q_2}{r_{12}}+\frac{q_1q_3}{r_{13}}+\frac{q_2q_3}{r_{23}}\right)$

由于静电力的保守性质（或等效地，所做工作的路径独立性），U 的最终公式与形成配置的方法无关。势能是当前配置状态的属性，而不是产生它的方法。

收费系统带来的潜力

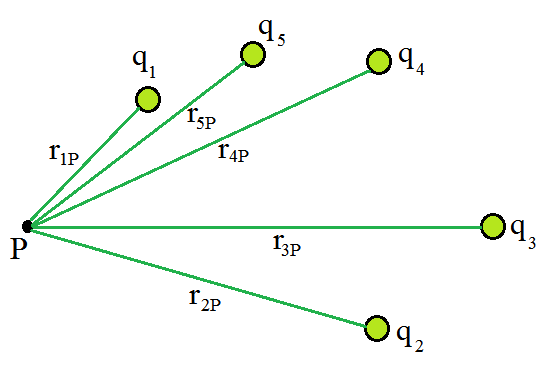
由电荷系统引起的某一点的电位是单个电荷引起的电位的总和。

假设一个电荷系统 q ₁ , q ₂ ,…, q _n具有相对于某个原点的位置向量 r ₁ , r ₂ ,…, r _n 。由于电荷 q _{1 在 P 处的电位 V 1是}

$V_1=\frac{1}{4\pi\epsilon_0}\frac{q_1}{r_{1P}}$

其中 r1P 是 q1 和 P 之间的距离。

类似地，由于 q ₂在 P 处的电位 V ₂和由于 q ₃引起的 V ₃可以写为：

$V_2=\frac{1}{4\pi\epsilon_0}\frac{q_2}{r_{2P}}\\ V_3=\frac{1}{4\pi\epsilon_0}\frac{q_3}{r_{3P}}$

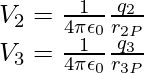
其中 r _2P和 r _3P分别是 P 与电荷 q ₂和 q ₃的距离；以此类推。根据叠加原理，由于总电荷配置，P 处的电位 V 是由于单个电荷引起的电位的代数和，即，

V = V ₁ + V ₂ + V ₃ +…… + V _n

上式可以表示为，

$V=\frac{1}{4\pi\epsilon_0}\frac{q_1}{r_{1P}}+\frac{1}{4\pi\epsilon_0}\frac{q_2}{r_{2P}}+ \frac{1}{4\pi\epsilon_0}\frac{q_3}{r_{3P}}+.....+\frac{1}{4\pi\epsilon_0}\frac{q_n}{r_{nP}}\\ V=\frac{1}{4\pi\epsilon_0}\left(\frac{q_1}{r_{1P}}+\frac{q_2}{r_{2P}}+\frac{q_3}{r_{3P}}+...+\frac{q_n}{r_{nP}}\right)$

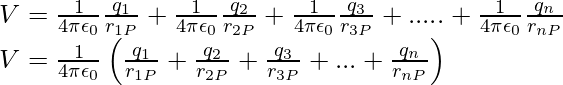
具有电荷密度 ρ (r) 的连续电荷分布必须分成大小为 Δv 的小体积元素，每个元素携带一个电荷 ρ Δv。然后计算每个体积元素的电位，并添加（或更准确地说，积分）所有这些贡献，以获得分布引起的总电位。

示例问题

问题 1：两个电荷 3 × 10 ^–8 C 和 –2 × 10 ^–8 C 相距 15 cm。连接两个电荷的线上的哪一点电位为零？取无穷大的势为零。

解决方案：

Let us take the origin O at the location of the positive charge. The line joining the two charges is taken to be the x-axis; the negative charge is taken to be on the right side of the origin.

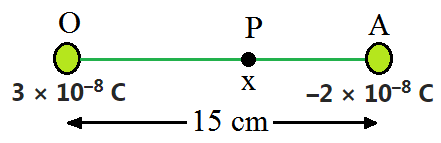
Let P be the expected point on the x-axis where the potential is zero. If x is the x-coordinate of P, and therefore x must be positive. If x lies between O and A, then

$\frac{1}{4\pi\epsilon_0}\left[\frac{3\times10^{-8}}{x\times10^{-2}}-\frac{2\times10^{-8}}{(15-x)\times10^{-2}}\right]=0$

Rearrange the above equation to find the value of x,

$\frac{3}{x}-\frac{2}{(15-x)}=0\\ 45-3x-2x=0\\ 5x=45\\ x=9$

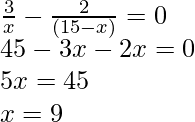
which gives x = 9 cm.

If x lies on the extended line OA, the required condition is

$\frac{3}{x}-\frac{2}{(x-15)}=0\\ 3x-45-2x=0\\ x=45$

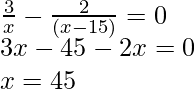
which results x = 45 cm.

On the side of the negative charge, an electric potential is zero at 9 cm and 45 cm away from the positive charge.

问题 2：图 (a) 和 (b) 分别显示了正点电荷和负点电荷的场线。

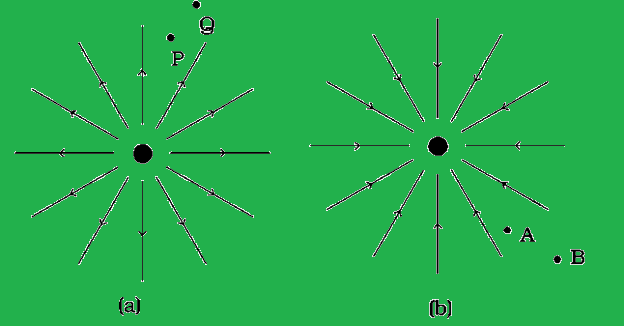
(a) 给出电位差 V _P – V _Q的符号； V _B - V _A 。

(b) 给出点 Q 和 P 之间小负电荷的势能差的符号； A和B。

(c) 给出场在将一个小的正电荷从 Q 移动到 P 时所做的功的符号。

(d) 给出外部机构在将小负电荷从 B 移动到 A 中所做的工作的符号。

(e) 一个小的负电荷从 B 到 A 的动能是增加还是减少？

解决方案：

(a) As V ∝ (1/r) and V_P> V_Q. Thus, (V_P – V_Q) is positive. Also, V_B is less negative than V_A. Thus, V_B > V_A or (V_B – V_A) is positive.

(b) A tiny negative charge will be attracted towards a positive charge. The negative charge moves from higher potential energy to lower potential energy. Therefore, the sign of potential energy difference of a small negative charge between Q and P is positive. Similarly, V_A > V_B and hence the sign of potential energy differences are positive.

(c) In moving a small positive charge from Q to P, work has to be done by an external agency against the electric field. Therefore, work done by the field is negative.

(d) In moving a small negative charge from B to A work has to be done by the external agency. It is positive.

(e) Due to the force of repulsion on the negative charge, velocity decreases and hence the kinetic energy decreases in going from B to A.

问题 3：500 µC 电荷位于边长为 10 cm 的正方形的中心。求在正方形的两个对角相对点之间移动 10 µC 的电荷所做的功。

解决方案：

Because these two locations are at equipotential, the work done in transporting a charge of 10 C between two diagonally opposite spots on the square will be zero.

问题 4： (a) 两个等势面可以相交吗？说明理由。

(b) 两个电荷 -q 和 +q 分别位于点 A (0, 0, – a) 和 B (0, 0, +a)。将测试电荷从点 P (7, 0, 0) 移动到 Q (-3,0,0) 需要做多少功？

解决方案：

(a) No, if they intersect, the electric field will be in two distinct directions, which is incorrect. If they cross, there will be two potential values at the same point of intersection. Because this isn’t conceivable, two equipotential surfaces can’t meet.

(b) Work done will be zero since both points P and Q are on the dipole’s equatorial line, which has V = 0 at all points. Furthermore, because any charge’s force is perpendicular to the equatorial line, no work is done.

问题 5：“对于任何电荷配置，通过一点的等势面垂直于电场。”证明合法。

解决方案：

Work done in moving a charge over an equipotential surface is zero, hence a point on it will be normal to the electric field.

W = Fs cos θ 

∴ cos θ = 0 

or 

θ = 90^o

问题6：为什么空心带电导体内的静电势必须在每一点都相同？

解决方案：

Because the electric field inside the hollow charged conductor is zero, no work is done in moving a small test charge within the conductor. As a result, the electrostatic potential inside a hollow charged conductor remains constant.