📌  相关文章
📜  弹簧的势能

📅  最后修改于: 2022-05-13 01:57:37.890000             🧑  作者: Mango

弹簧的势能

从汽车的减震器到厨房的打火机，我们日常生活的几乎所有机械方面都使用了弹簧。使用弹簧是因为它们具有变形并再次恢复其自然状态的特性。每当弹簧被拉伸或压缩时，就会在与这种变化相反的方向上感受到力。发生这种情况是因为当弹簧偏离其平均位置时，它会试图回到那里。这个力由胡克定律给出，帮助我们分析储存在弹簧中的能量。

胡克定律

拉伸金属弹簧或绳索等弹性物体需要用力。每当弹性物体被拉伸或压缩时。它倾向于施加力量来反对这种形状的变化。这个力是由胡克定律给出的。弹簧施加的力称为恢复力，因为它总是与变形方向相反。

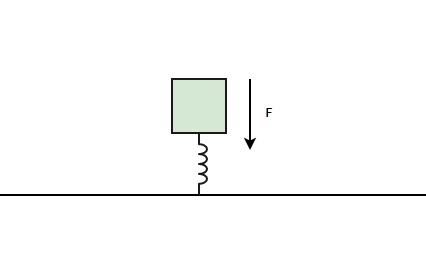
因此，每当弹簧向下拉伸时，力就会向上施加，反之亦然。

弹性势能

弹性势能是当对弹性物体施加力以使其形状和大小变形时存储在弹性物体中的能量。然后能量被储存，直到力被移除。之后，物体开始恢复正常形状，这种能量被转换成其他类型的能量。一些储存弹性势能的物体的例子是：

  1. 拉伸或压缩的弹簧。
  2. 一条扭曲的橡皮筋。
  3. 一个弹力球，在撞击墙壁并弹回的那一刻被压缩。

计算储存在弹簧中的势能

上面提到的胡克定律说明了弹簧中的恢复力如何随着弹簧平均位置的净位移而变化。考虑净位移为\Delta x恢复力用 F 表示，

F = -kx

这种力量是一种保守力量，而保守力量具有与之相关的势能。众所周知，所做的功定义为力和位移的乘积。

W = Fx

对于可变力 F 和净位移 x，

W = \int^{x}_{0}Fdx

现在在位移 x 处，对于无限小的位移\Delta x和力 F，

dW = Fdx

⇒ dW = -kxdx

将上述等式积分为完成的总功，

dW = kxdx

⇒∫dW = ∫kxdx

⇒ W = \frac{kx^2}{2}

因此，这是位移 x 所做的总功。所做的这项工作作为势能存储在弹簧中。这一事实也可以通过弹簧的力与位移图来验证。力-位移图中曲线下的面积给出了存储在弹簧中的弹性势能。

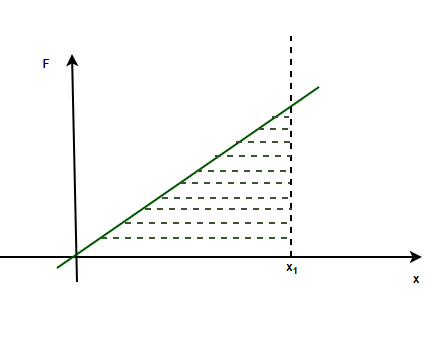
曲线下面积 = 曲线阴影区域的面积

= \frac{1}{2} \times base \times height

= \frac{1}{2} \times x \times (kx)

= \frac{1}{2}kx^2

两种方法都给出了相同的答案。

因此，以“x”位移存储在弹簧中的弹性势能由下式给出，

体育= \frac{1}{2}kx^2

示例问题

问题1：求弹簧压缩0.2m时k=50N/m时弹簧中储存的弹性势能。

回答：

问题2：求弹簧压缩0.1m时k=100 N/m时弹簧中储存的弹性势能。

回答：

问题 3：求弹簧从自然长度 0.5m 拉伸到 0.1m 时储存在弹簧中的弹性势能 k = 100 N/m。

回答：

问题 4：求弹簧从自然长度 1 m 拉伸到 0.5 m 时储存在 k = 100 N/m 的弹簧中的弹性势能。

回答：

问题 5：弹簧常数 k = 100 N/m 的弹簧最初被压缩 x = 0.4m，然后在 x = 0.2m 压缩时释放并停止。求恢复力在这个过程中所做的功。

回答：

问题 6：弹簧常数 k = 20 N/m 的弹簧最初压缩 x = 0.5m，然后在 x = 0.1m 压缩时释放并停止。求恢复力在这个过程中所做的功。

回答：