选择排序算法

选择排序是一种算法，它在每次迭代中从未排序列表中选择最小的元素，并将该元素放置在未排序列表的开头。

选择排序如何工作？

将第一个元素设置为minimum 。

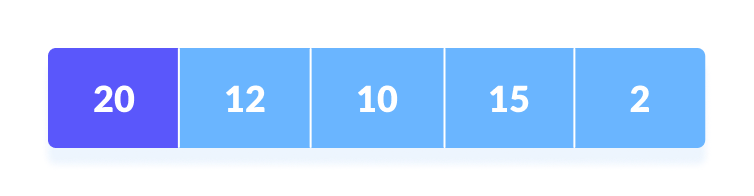
至少选择第一个元素
将minimum与第二个元素进行比较。如果第二个元素小于minimum ，则将第二个元素分配为minimum 。
将minimum与第三个元素进行比较。同样，如果第三个元素较小，则将minimum分配给第三个元素，否则不执行任何操作。该过程一直进行到最后一个元素。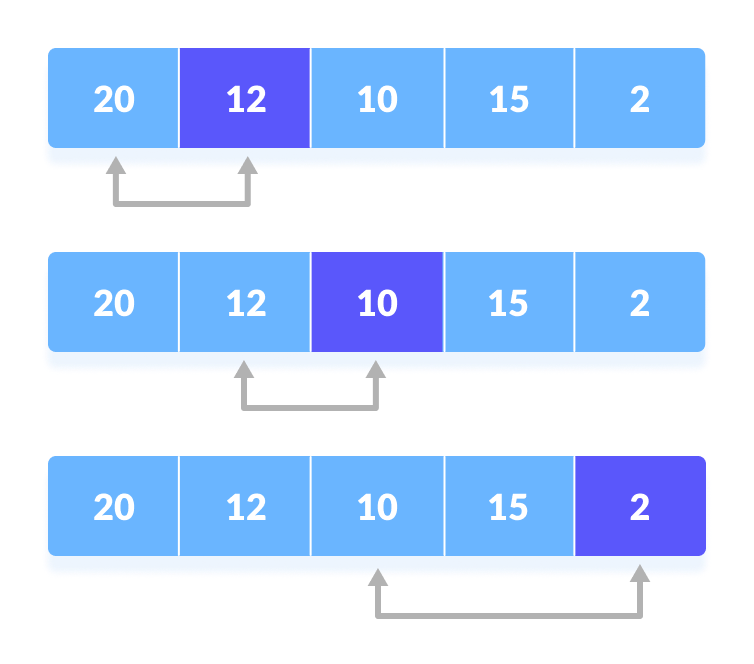

比较最小值与其余元素
每次迭代后， minimum被置于未排序列表的前面。

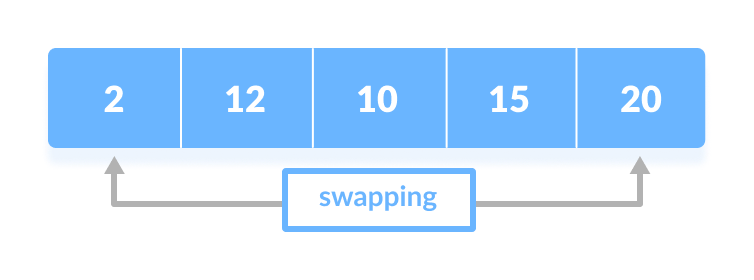
最小交换第一
对于每次迭代，索引从第一个未排序元素开始。重复步骤1到3，直到所有元素都放置在正确的位置。

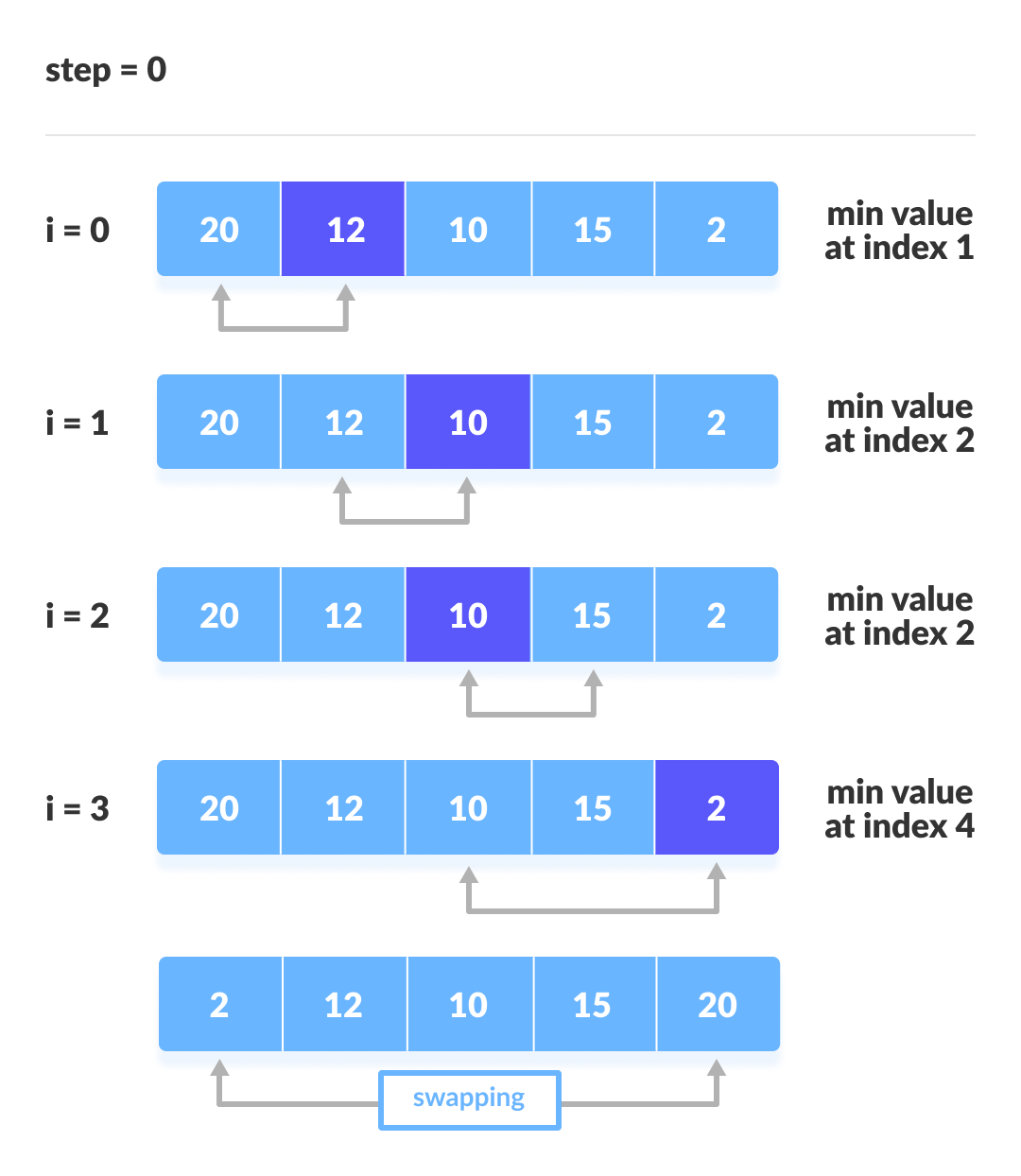
第一次迭代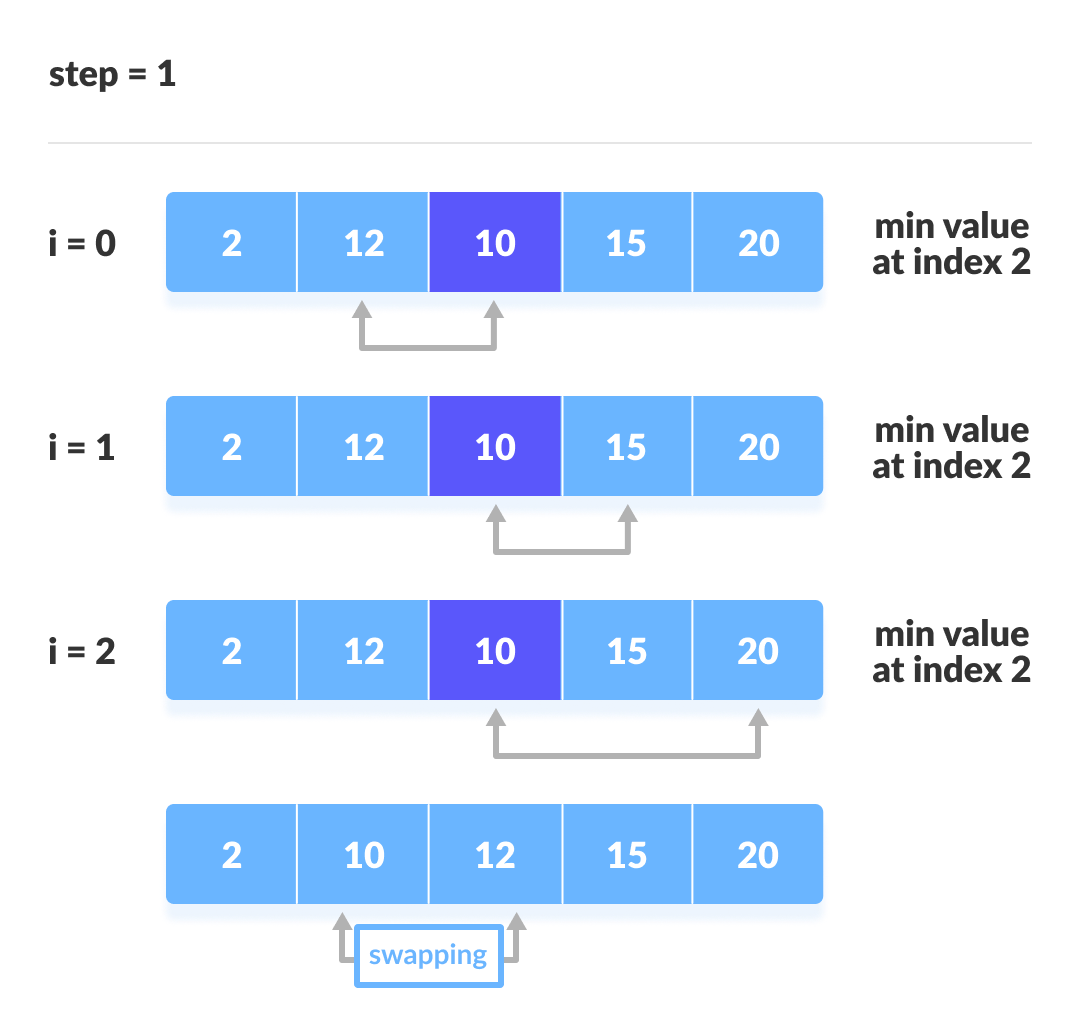

第二次迭代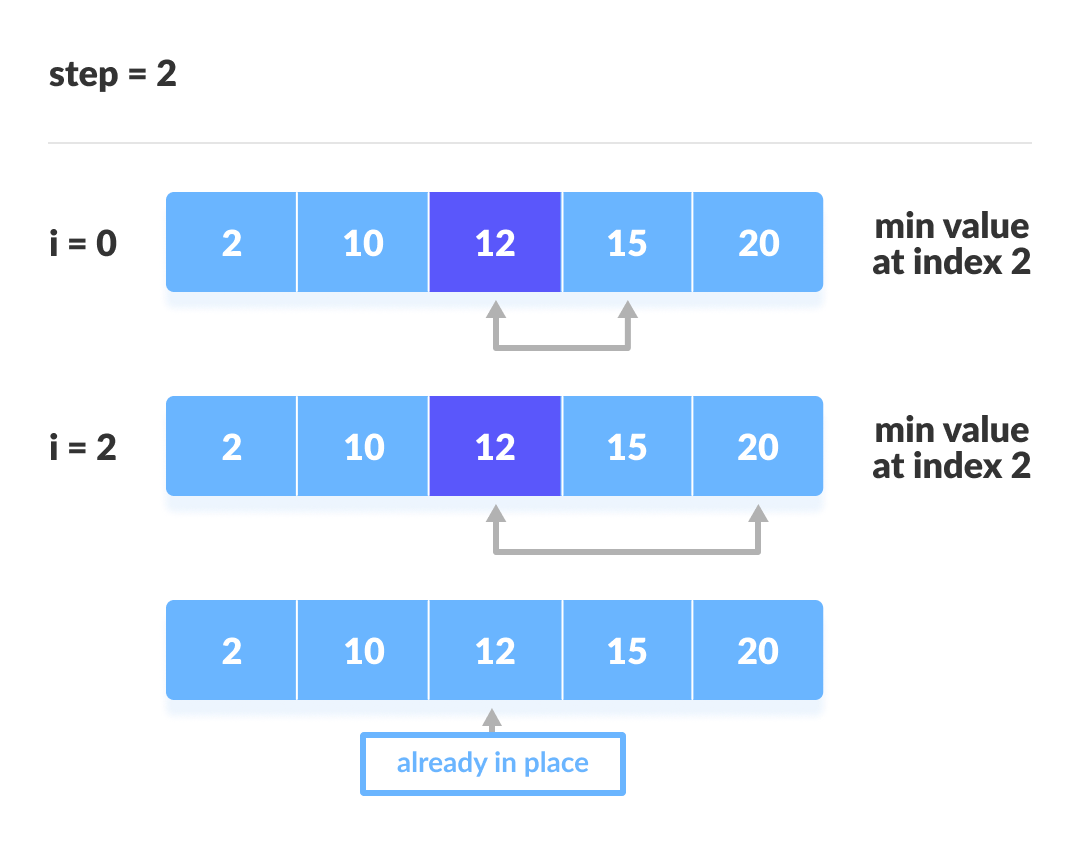

第三次迭代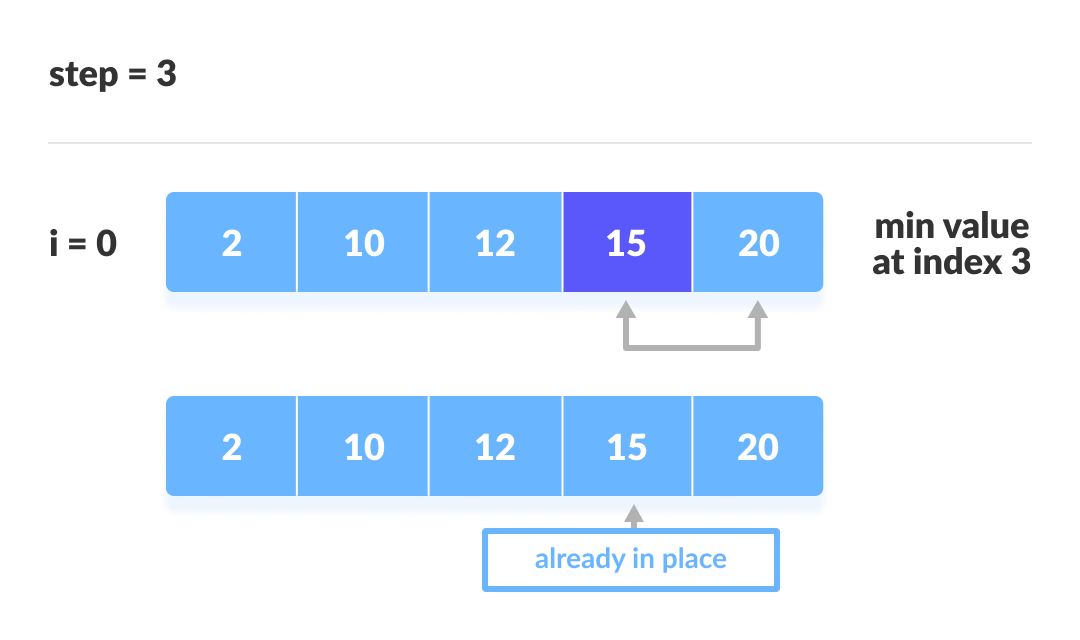

第四次迭代

selectionSort(array, size)
  repeat (size - 1) times
  set the first unsorted element as the minimum
  for each of the unsorted elements
    if element < currentMinimum
      set element as new minimum
  swap minimum with first unsorted position
end selectionSort

Python，Java和C / C++示例

Python

爪哇

C++

# Selection sort in Python


def selectionSort(array, size):
   
    for step in range(size):
        min_idx = step

        for i in range(step + 1, size):
         
            # to sort in descending order, change > to < in this line
            # select the minimum element in each loop
            if array[i] < array[min_idx]:
                min_idx = i
         
        # put min at the correct position
        (array[step], array[min_idx]) = (array[min_idx], array[step])


data = [-2, 45, 0, 11, -9]
size = len(data)
selectionSort(data, size)
print('Sorted Array in Ascending Order:')
print(data)

// Selection sort in Java

import java.util.Arrays;

class SelectionSort {
  void selectionSort(int array[]) {
    int size = array.length;

    for (int step = 0; step < size - 1; step++) {
      int min_idx = step;

      for (int i = step + 1; i < size; i++) {

        // To sort in descending order, change > to < in this line.
        // Select the minimum element in each loop.
        if (array[i] < array[min_idx]) {
          min_idx = i;
        }
      }

      // put min at the correct position
      int temp = array[step];
      array[step] = array[min_idx];
      array[min_idx] = temp;
    }
  }

  // driver code
  public static void main(String args[]) {
    int[] data = { 20, 12, 10, 15, 2 };
    SelectionSort ss = new SelectionSort();
    ss.selectionSort(data);
    System.out.println("Sorted Array in Ascending Order: ");
    System.out.println(Arrays.toString(data));
  }
}

// Selection sort in C

#include 

// function to swap the the position of two elements
void swap(int *a, int *b) {
  int temp = *a;
  *a = *b;
  *b = temp;
}

void selectionSort(int array[], int size) {
  for (int step = 0; step < size - 1; step++) {
    int min_idx = step;
    for (int i = step + 1; i < size; i++) {

      // To sort in descending order, change > to < in this line.
      // Select the minimum element in each loop.
      if (array[i] < array[min_idx])
        min_idx = i;
    }

    // put min at the correct position
    swap(&array[min_idx], &array[step]);
  }
}

// function to print an array
void printArray(int array[], int size) {
  for (int i = 0; i < size; ++i) {
    printf("%d  ", array[i]);
  }
  printf("\n");
}

// driver code
int main() {
  int data[] = {20, 12, 10, 15, 2};
  int size = sizeof(data) / sizeof(data[0]);
  selectionSort(data, size);
  printf("Sorted array in Acsending Order:\n");
  printArray(data, size);
}

// Selection sort in C++

#include 
using namespace std;

// function to swap the the position of two elements
void swap(int *a, int *b) {
  int temp = *a;
  *a = *b;
  *b = temp;
}

// function to print an array
void printArray(int array[], int size) {
  for (int i = 0; i < size; i++) {
    cout << array[i] << " ";
  }
  cout << endl;
}

void selectionSort(int array[], int size) {
  for (int step = 0; step < size - 1; step++) {
    int min_idx = step;
    for (int i = step + 1; i < size; i++) {

      // To sort in descending order, change > to < in this line.
      // Select the minimum element in each loop.
      if (array[i] < array[min_idx])
        min_idx = i;
    }

    // put min at the correct position
    swap(&array[min_idx], &array[step]);
  }
}

// driver code
int main() {
  int data[] = {20, 12, 10, 15, 2};
  int size = sizeof(data) / sizeof(data[0]);
  selectionSort(data, size);
  cout << "Sorted array in Acsending Order:\n";
  printArray(data, size);
}

复杂

Cycle	Number of Comparison
1st	(n-1)
2nd	(n-2)
3rd	(n-3)
…	…
last	1

比较次数： (n - 1) + (n - 2) + (n - 3) + ..... + 1 = n(n - 1) / 2几乎等于n ² 。

复杂度 = O(n ² )

同样，我们可以通过简单地观察循环数来分析复杂性。有2个循环，因此复杂度为n*n = n ² 。

时间复杂度：

最坏情况下的复杂度： O(n ² )
如果我们要按升序排序，而数组按降序排序，则会发生最坏的情况。
最佳情况复杂度： O(n ² )
当数组已经排序时发生
平均案例复杂度： O(n ² )
当数组中的元素处于混乱顺序(既不升也不降)时，就会发生这种情况。

选择排序的时间复杂度在所有情况下都是相同的。在每一步中，您都必须找到最小的元素并将其放在正确的位置。直到没有到达数组的末尾，才知道最小元素。

空间复杂度：

空间复杂度为O(1)因为使用了额外的可变temp 。

选择排序应用

在以下情况下使用选择排序：

一个小清单要排序
交换成本无所谓
必须对所有要素进行检查
写入存储器的成本很重要，就像在闪存中一样O(n ² )与冒泡排序的O(n ² )相比，写入/交换次数为O(n) O(n ² ) )

在本教程中，您将学习选择排序的工作方式。另外，您还将找到使用C，C++，Java和Python进行选择排序的工作示例。

选择排序如何工作？

选择排序算法

Python，Java和C / C++示例

复杂

选择排序应用