高级差异化

导数用于衡量任何数量的变化率。这个过程称为分化。它可以被认为是微积分理论的基石。从几何上讲，任何函数在特定点的导数给出了函数在该点的切线的斜率。有许多方法可以求解和计算函数的导数。通常的方法是通过链式法则和幂法则进行计算。在某些情况下，函数变得过于复杂而无法计算。在这种情况下，必须遵循某些方法以简化计算。

衍生品

导数被定义为观察到的某些变量/数量的变化率。假设变量或数量由方程 f(x) 控制。该函数的导数表示为$\frac{df(x)}{dx}$或 F(x)。必须牢记某些函数的导数。这些函数有助于简化导数的计算。下表显示了一些标准函数的派生值。

Function	Derivative
sin(x)	cos(x)
cos(x)	-sin(x)
xn	nxn-1
tan(x)	sec2(x)
cot(x)	cosec2(x)

Sometimes, functions are made up of products or division of two or more functions. In such cases, the product and quotient rule is used. Consider a function h(x), 

Product Rule: If h(x) = f(x).g(x) 

h'(x) = f'(x)g(x) + f(x)g'(x) 

Quotient Rule: If h(x) = f(x)/g(x) 

 $h'(x) = \frac{g(x)f'(x) - f(x)g'(x)}{(g(x))^2}$

链式法则

通常这些函数由两个或多个标准函数的组合组成。在这种情况下，找到导数的常用技术是不够的。在这种情况下，链式法则就派上用场了。将 f(x) 和 g(x) 视为两个可微函数，假设它们生成一个新函数h(x) = f(g(x))，它基本上是这些函数的组合。在这种情况下，可以应用如下所述的链式法则，

$h'(x) = \frac{dh(x)}{dx} \\ = \frac{df(g(x))}{dx} \\ = \frac{df(g(x))}{dx}\frac{dg}{dx}$

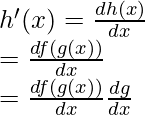
高级差异化

有些函数计算导数并不容易。在计算它们的导数之前，它需要一些代数操作。此类函数的示例是隐函数、参数函数、高阶导数和对数导数。所有这些函数都是由标准函数组成的，但是通过简单的链式法则或幂法则来解决它们是非常复杂的。

隐函数的导数

隐式函数是形式为 f(x, y) = 0 的函数。常见的函数是形式为 y = f(x)。这些函数是显式函数，因为 y 的定义被清楚地表达并以 x 表示。在隐函数中，x 和 y 之间的关系并不完全清楚。让我们以这种方法为例。

问题：求函数x ² + y ² = 4 的导数。

回答：

Notice that the given function is an implicit function, 

x² + y² = 4

⇒ x² + y² – 4 = 0 

This function is in the form of f(x, y) = 0. 

 $\frac{d(f(x,y))}{dx} = 0 \\ = \frac{d(x^2 + y^2 - 1)}{dx} = 0 \\ = \frac{d}{dx}(x^2) + \frac{d}{dx}y^2 - \frac{d}{dx}(1) = 0 \\ = 2x + 2y\frac{dy}{dx} = 0 \\ = \frac{dy}{dx} = \frac{-x}{y}$

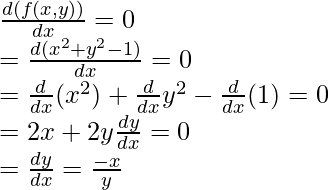
参数函数的导数

在上面研究的函数中，函数只有两个变量，并且在它们之间定义了关系。通常，在某些函数中，两个变量之间的关系使用第三个变量来描述。这些函数称为参数函数。例如，x = f(t) 和 y = g(t) 在一个这样的函数中。在这个函数中，变量 x 和 y 是相对于变量“t”定义的。在这种情况下，计算 x 相对于 y 的变化率并不那么清楚。

问题：计算以下函数的导数。

x = t ²和 y = t ³ + 3。

回答：

This is an example of a parametric function. In this case, the derivatives are calculated with respect to the third variable for both the functions. 

x = t²

Differentiating with respect to “t”,

$\frac{dx}{dt} = \frac{d}{dt}(t^2) \\ = \frac{dx}{dt} = 2t$

$\frac{dy}{dt} = \frac{d}{dt}(t^3 +3) \\ = \frac{dy}{dt} = 3t^2$

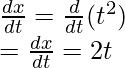
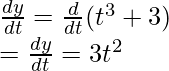
Now these derivatives can be combined. 

$\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}} \\ = \frac{dy}{dx} = \frac{3t^2}{2t} \\ = \frac{dy}{dx} = \frac{3}{2}t$

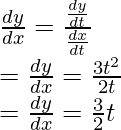
高阶导数

就像导数被定义为数量的变化率一样。同样，变化率的变化率也可以计算出来。这种衡量导数变化率的导数称为高阶导数。例如，位置的变化率由速度给出，加速度测量速度的变化率。对于函数f(x)，

$\frac{df}{dx}$表示一阶导数。相似地， $\frac{d^2f}{dx^2}$表示二阶导数，以此类推。

示例问题

问题 1：求 f(x) = x ³的二阶导数。

回答：

The first order derivative of this function is given by, 

f(x) = x³

⇒ f'(x) = 3x²

Differentiating the function again, 

f”(x) = 6x 

问题 2：求给定函数的导数，

f(x) = sin(x)cos(x)

答案：

Given: f(x) = sin(x)cos(x) 

The given function is the product of two functions, thus the product rule should be applied. 

f(x) = h(x)g(x) 

⇒f'(x) = h'(x)g(x) + h(x)g'(x)

Here, h(x) = sin(x) and g(x) = cos(x)

f'(x) = h'(x)g(x) + h(x)g'(x)

⇒ f'(x) = $\frac{d}{dx}(sin(x))cos(x) + sin(x)\frac{d}{dx}(cos(x)$

⇒f'(x) = $sin^2(x) - cos^2(x)$

问题 3：求给定函数的导数，

f(x) = xsin(x)

答案：

Given: f(x) = xcos(x) 

The given function is the product of two functions, thus the product rule should be applied. 

f(x) = h(x)g(x) 

⇒f'(x) = h'(x)g(x) + h(x)g'(x)

Here, h(x) = x and g(x) = sin(x)

f'(x) = h'(x)g(x) + h(x)g'(x)

⇒ f'(x) = $\frac{d}{dx}(x)sin(x) + x\frac{d}{dx}(sin(x))$

⇒f'(x) = sin(x) + xcos(x)

问题 4：求函数x ² + 2x + y = 1 的导数。

回答：

Notice that the given function is an implicit function, 

x² + 2x + y = 1. 

⇒ x² + 2x + y – 1 = 0 

This function is in the form of f(x, y) = 0. 

 $\frac{d(f(x,y))}{dx} = 0 \\ = \frac{d(x^2 + y + 2x - 1)}{dx} = 0 \\ = \frac{d}{dx}(x^2) + \frac{d}{dx}y + \frac{d}{dx}(2x) - \frac{d}{dx}(1) = 0 \\ = 2x + \frac{dy}{dx} + 2= 0 \\ = \frac{dy}{dx} = -(2 + 2x)$

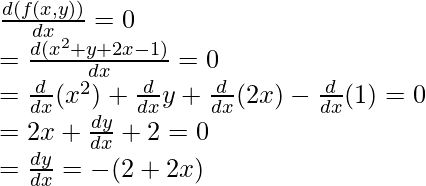
问题 5：计算以下函数的导数。

x = t + 5 和 y = 3t ³ + 6。

回答：

This is an example of a parametric function. In this case, the derivatives are calculated with respect to the third variable for both the functions. 

x = t + 5

Differentiating with respect to “t”,

$\frac{dx}{dt} = \frac{d}{dt}(t + 5) \\ = \frac{dx}{dt} = 1$

$\frac{dy}{dt} = \frac{d}{dt}(3t^3 +3) \\ = \frac{dy}{dt} = 9t^2$

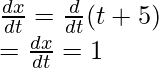
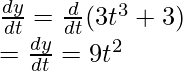
Now these derivatives can be combined. 

$\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}} \\ = \frac{dy}{dx} = \frac{9t^2}{1} \\ = \frac{dy}{dx} = 9t^2$

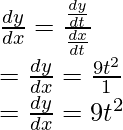
问题 6：求 f(x) = e ^x + sin(x) 的三阶导数。

回答：

The first order derivative of this function is given by, 

f(x) = e^x + sin(x)

⇒ f'(x) = e^x + cos(x)

Differentiating the function again, 

f”(x) = e^x – sin(x)

Differentiating the function again, 

f”'(x) = e^x – sin(x)