表示 1.3212121… 作为有理数

有理数是一种实数，公式为m/n，其中n≠0。有理数除以十进制数，可以结束也可以重复。这些数字写成 p/q，其中 p 和 q 是整数并且 q ≠ 零。由于数字的基本结构是 p/q 形式，大多数人发现很难区分分数和有理数。整数构成分数，而整数构成有理数的分子和分母。

十进制数到有理数的转换

以下是将十进制数转换为有理数的步骤，

• 第 1 步：识别重复小数并将其等于 x
• 第 2 步：通过从重复数字顶部删除条并列出重复数字至少两次，以十进制形式写出来。

For Example, write x = 0.3 bar as x = 0.333… and x = 0.33 bar as x = 0.333333…

• 第 3 步：检查有条的位数。
• 第4步：如果有重复小数的数字有1位重复则乘以10，如果有重复两位则乘以100，小数点后重复三位则乘以1000，以此类推。
• 第五步：然后从第四步得到的方程中减去第二步得到的方程。
• 第 6 步：无论剩下什么，将等式两边除以 x 系数。
• 第 7 步：最后以最简单的形式写出有理数。

表达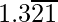$1.3\overline{21}$ = 1.3212121… 作为有理数

解决方案：

Given: 1.3212121… 

Let’s assume x = 1.3212121…. ⇢  (1)

And, there are two digits after decimal which are repeating, so multiply equation (1) both sides by 100,

So 100 x = $132. 12121\overline{21}$ ⇢ (2)

Now subtract equation (1) from equation (2)

100x – x = $132. 12121\overline{21} - 1.3\overline{21}$

99x = 130.8

x = 130.8/99    

= 1308/990

=  436/330

1.3212121…. can be expressed 436/330  in form of p/q as rational number.

示例问题

问题 1：将 5.959595... 表示为有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given: 5.959595…. or$5.\overline{95}$

Let’s assume x = 5.959595… ⇢  (1)

And, there are two digits after decimal which are repeating, so multiply equation (1) both sides by 100,

So 100 x =  $595.\overline{95}$⇢ (2)

Now subtract equation (1) from equation (2)

100x – x = $595.\overline{95}- 5.\overline{95}$

99x = 590

x = 590/99                    

5.959595… can be expressed 590/99 in form of p/q as rational number.

问题 2：将 26.333333... 表示为有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given: 26.333333… or $26.\overline{33}$

Let’s assume x = 26.333333…. ⇢  (1)

And, there are two digits after decimal which are repeating, so multiply equation (1) both sides by 100,

So 100 x = $2633.\overline{33}$ ⇢ (2)

Now subtract equation (1) from equation (2)

100x – x = $2633.\overline{33}- 26.\overline{33}$

99x = 2607

x = 2607 /99                    

26.333333… can be expressed 2607/99 in form of p/q as rational number.

问题 3：将 9.969696... 表示为有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given : 9.969696… or $9.\overline{9696}$

Let’s assume x = 9.969696… ⇢ (1)

And, there are two digits after decimal which are repeating, so multiply equation (1) both sides by 100,

So 100 x =  $996.\overline{96}$⇢ (2)

Now subtract equation (1) from equation (2)

100x – x = $996.\overline{96}- 9.\overline{96}$

99x = 987

x = 987/99       

=  329/33             

9.969696…. can be expressed 329/33 in form of p/q as rational number.

问题 4：将 10.65656565... 表示为一个有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given: 10.65656565… or $10.\overline{65}$

Let’s assume x = 10.65656565… ⇢  (1)

And, there are two digits after decimal which are repeating, so multiply equation (1) both sides by 100,

So 100 x =$1065.\overline{65}$ ⇢ (2)

Now subtract equation (1) from equation (2)

100x – x = $1065.\overline{65} - 10.\overline{65}$

99x = 1055

x = 1055/99    

= 1055/99

10.656565…. can be expressed 10555/99 in form of p/q as rational number.

问题 5：将 159.986986986... 表示为一个有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given: 159.986986986… or $159.\overline{986}$

Let’s assume x = 159.986986986… ⇢ 1

And, there are three digits after decimal which are repeating,

So multiply equation 1 both sides by 1000

So 1000 x = $159986.\overline{986}$ ⇢ (2)

Now subtract equation (1) from equation (2)

1000x – x = $159986.\overline{986}- 159.\overline{986}$

999x = 159827

x = 159827 / 99

159.986986986 can be expressed 159827 / 99 in form of p/q as rational number.

问题 6：将 56.55555.. 表示为 p/q 形式的有理数，其中 p 和 q 没有公因数。

解决方案：

Given: 56.55555 or$56.\overline{5}$

Lets assume x = 56.55555… ⇢ (1)

And, there are one digit after decimal which are repeating,

So, multiply equation (1) both sides by 10,

So 10 x = $565.\overline{5}$ ⇢ (2)

Now subtract equation (1) from equation (2)

 10x – x = $565.\overline{5}- 56.\overline{5}$

9x = 509

x = 509 /9

56.55555… can be expressed 509/9 as rational number.

问题 7：将 0.99999... 表示为一个有理数，形式为 p/q，其中 p 和 q 没有公因数。

解决方案：

Given: 0.99999… or$0.\overline{9}$

Let’s assume x = 0.99999…. ⇢  (1)

And, there are one digit after decimal which are repeating, so multiply equation (1) both sides by 10,

So 10 x = $9.\overline{9}$⇢ (2)

Now subtract equation (1) from equation (2)

10x – x = $9.\overline{9} - 0.\overline{9}$

99x = 9

x = 9/99    

= 1/11

0.99999…. can be expressed 1/11 in form of p/q as rational number.