正则金字塔公式的平截头体

金字塔是一个数学图形，具有三个或四个三角形面作为边和一个平坦的多边形底座，可以是三角形，正方形或矩形等。边三角形面称为侧面。所有三角形面的共同交汇点称为顶点。对于给定的金字塔，其底边为“b”边，边为“2b”，面和顶点为“b + 1”。

无花果。 1：金字塔

无花果。 2：金字塔

正则金字塔公式的平截头体

正金字塔的平截头体是在锥体顶端的顶部被切掉时形成的。将金字塔切掉后形成的剩余几何图形称为平截头体。如此形成的平截头体有 2 个底座，一个是金字塔的实际平底，另一个是当金字塔顶部分离时形成的平底。

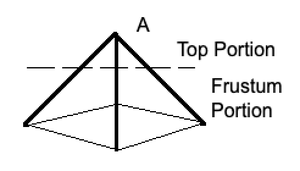
无花果。 3：当金字塔的顶部被切掉时，会形成一个截锥体

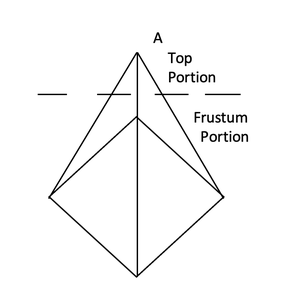
无花果。 4：当金字塔的顶部被切掉时，会形成一个截锥体

它们用“b ₁ ”和“b ₂ ”表示。平截头体的平顶底和平截头体平底之间的垂直距离称为高度，用“h”表示。同样，平截头体的两个基部之间的倾斜高度用“s”表示。知道截头体体积的两个底面面积和高度值，求出面的侧面的两个底面的周长和斜高值，就可以计算出截头体的侧面面积和体积.我们将看到如何通过一个简单的公式计算两者。

截锥体体积

Volume of Frustum is given as,

(b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

Here,

b₁ is an area of first base.

b₂ is Ares of second base.

h is the height of the frustum.

截锥体的侧表面积

Lateral Surface Area of Frustum is given as,

(p₁ + p₂) × s/2

Here,

p₁ is the perimeter of first base

p₂ is the perimeter of the second base

s is the slant height of the frustum

示例问题

问题1：Frustum的底边b ₁的面积是80 m ² ，底边b ₂的面积是20 m ² 。如果截锥体的高度是 3 m，那么截锥体的体积是多少。

解决方案：

We know, Volume of Frustum = (b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

Given b₁ = 80 m²

b₂ = 20 m²

h = 30 m

Putting values in the given Volume of Frustum formula,

(b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

= (80 +  20 + (80 × 20)^1/2) × 30/3

= (100 + (1600)^1/2) × 1

= 100 + 40

= 140 m³

问题2：平截头体底边₁周长为50m，平截头体底边₂周长为10m。如果截头体的倾斜高度为 6 m，那么截头体的侧表面积是多少。

解决方案：

We know, Lateral Surface Area of Frustum = (p₁ + p₂) × s/2 

Given p₁ = 50 m

p₂ = 10 m

s = 6 m

Putting values in the given Lateral Surface Area of Frustum 

= (p₁ + p₂) × s/2

= (50 + 10) × 6/2

= 60 × 6 / 2

= 180 m

问题3：Frustum的底边b ₁的面积是25 m ² ，底边b ₂的面积是4 m ² 。如果截锥体的高度是 0.3 m，截锥体的体积是多少。

解决方案：

We know, Volume of Frustum = (b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

Given b₁ = 25 m²

b₂ = 4 m²

h = 0.3 m

Putting values in the given Volume of Frustum formula

(b₁ + b₂ + (b₁ x b₂)^1/2) × h/3

(25 +  4 + (25 x 4)^1/2) × 0.3/3

= (29 + 10)/ 10

= 39/10

= 3.9 m³

问题4：平截头体基部₁周长为45m，平截头体基部₂周长为15m。如果截头体的倾斜高度为 5 m，那么截头体的侧表面积是多少。

解决方案：

We know, Lateral Surface Area of Frustum = (p₁ + p₂) × s/2

Given p₁ = 45 m

p₂ = 15 m

s = 5 m

Putting values in the given Lateral Surface Area of Frustum

= (p₁ + p₂) × s/2

= (45 + 15) × 5/2

= 60 × 5 / 2

= 30 × 5

= 150 m

问题 5：Frustum 的底边 b ₁的面积是 10 m ² ， Frustum 的底边 b ₂的面积是 40 m ² 。如果截锥体的高度是 6 m，那么截锥体的体积是多少。

解决方案：

We know, Volume of Frustum = (b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

Given b₁ = 10 m²

b₂ = 40 m²

h = 6 m

Putting values in the given Volume of Frustum formula

(b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

(10 +  40 + (10 × 40)^1/2) × 6/3

= (50 + 20) × 2

= 140 m³

问题6：平截头体基部₁周长为75m，平截头体基部₂周长为25m。如果截头体的倾斜高度为 7 m，那么截头体的侧表面积是多少。

解决方案：

We know, Lateral Surface Area of Frustum = (p₁ + p₂) × s/2

Given p₁ = 75 m

p₂ = 25 m

s = 7 m

Putting values in the given Lateral Surface Area of Frustum

= (p₁ + p₂) × s/2

= (75 + 25) × 7/2

= 100 × 7 / 2

= 100 × 3.5

= 350 m

问题 7：Frustum 的底边 b ₁的面积是 80 m ² ， Frustum 的底边 b ₂的面积是 20 m ² 。如果截锥体的高度是 3 m，那么截锥体的体积是多少。

解决方案：

We know, Volume of Frustum = (b₁ + b₂ + (b₁ × b₂)^1/2) × h/3

Given b₁ = 80 m²

b₂ = 20 m²

h = 30 m

Putting values in the given Volume of Frustum formula

(b₁ + b₂ + (b₁ x b₂)^1/2) × h/3

= (80 +  20 + (80 x 20)^1/2) × 30/3

= (100 + (1600)^1/2) × 1

= 100 + 40

= 140 m³