圆柱体公式的表面积
测量是一门数学学科,通过计算单个项目或一组对象的高度、宽度、深度、周长、面积和体积来处理测量。它描述了许多几何形状,例如二维形状,以及它们的属性和使用的公式。它适用于正方形、矩形和三角形等二维形式,以及立方体、长方体和圆柱体等三维对象。
圆筒
圆柱体是由两个平行的圆形底面通过曲面连接而成的三维结构。圆形底座的中心相互重叠形成一个直圆柱体。轴是连接两个中心的线段,代表圆柱体的高度。圆柱体的顶视图类似于圆形,而侧视图类似于矩形。与圆锥体、立方体或长方体不同,圆柱体由于其弯曲形式和缺乏直线而缺乏顶点。它的脸上有两个同心圆。
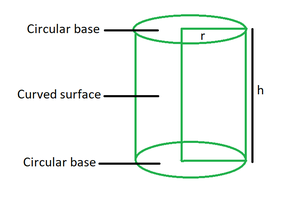
圆柱表面积
圆柱体的表面积定义为圆柱体底部的平面和圆柱体的曲面所覆盖的空间量。圆柱体的总表面积包括圆柱体的两个圆形底面的面积以及曲面的面积。圆柱体表面积公式用于计算圆柱体的底面和曲面所占的表面积。因为圆柱体有曲面,我们可以同时表示它的曲面面积和总表面积。圆柱体有两种表面积:总表面积和曲面面积。我们将一一讨论它们。
圆柱的曲面面积
圆柱体的曲面区域被包围在两个平行的圆形底座之间。它也被称为侧表面积。公式如下:
Curved surface area = 2πrh
where,
r = radius of the cylinder
h = height of cylinder
圆柱体的总表面积
圆柱体的总表面积是它的曲面面积和它的两个圆形底面的面积之和。它是通过将两个底面和曲面的面积相加来计算的。因此,圆柱体总表面积的公式如下:
Total cylinder surface area = 2πr2 + 2πrh = 2πr(r + h)
where,
r = radius of the cylinder
h = height of cylinder
推导
Consider a cylinder whose radius is r and height is h.
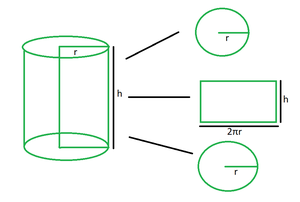
The cylinder is divided into three parts: one circular base, one rectangular area and another circular base.
The rectangular area has length of 2πr and breadth of h. So, the area is, A1 = 2πrh, which is also the curved surface area of the cylinder.
The area of a circular base with radius r is given by, πr2. So, the area of two such bases is, A2 = (πr2 + πr2) = 2πr2.
Now, the total surface area of the cylinder is the sum of above two areas.
A = A1 + A2
= 2πr2 + 2πrh
= 2πr(r + h)
This derives the formula for surface area of a cylinder.
示例问题
问题 1. 求半径为 3 cm、高为 7 cm 的圆柱的曲面面积。
解决方案:
We have, r = 3 and h = 7.
Curved surface area of cylinder = 2πrh
= 2 (22/7) (3) (7)
= 2 (22) (3)
= 132 cm2
问题 2. 求半径为 5 厘米,高为 14 厘米的圆柱体的曲面面积。
解决方案:
We have, r = 5 and h = 14.
Curved surface area of cylinder = 2πrh
= 2 (22/7) (5) (14)
= 2 (22) (5) (2)
= 440 cm2
问题 3. 求曲面面积为 220 平方厘米、高 7 厘米的圆柱体的半径。
解决方案:
We have, A = 220 and h = 7.
Curved surface area of cylinder = 2πrh
220 = 2 (22/7) (r) (7)
220 = 44r
r = 220/44
r = 5 cm
问题 4. 求半径为 21 厘米、高为 42 厘米的圆柱体的总表面积。
解决方案:
We have, r = 21 and h = 42.
Total surface area = 2πr2 + 2πrh
= 2 (22/7) (21) (21) + 2 (22/7) (21) (42)
= 2 (22) (3) (21) + 2 (22) (3) (42)
= 2772 + 5544
= 8316 sq. cm
问题 5. 求曲面面积 176 平方厘米、高 21 厘米的圆柱体的总面积。
解决方案:
We have, A = 176 and h = 21.
Curved surface area of cylinder = 2πrh
176 = 2 (22/7) (r) (21)
176 = 2 (22) (r) (3)
r = 176/132
r = 1.33 cm
Total surface area = 2πr2 + 2πrh
= 2 (3.14) (1.33) (1.33) + 176
= 11.10 + 176
= 187.1 sq. cm
问题 6. 如果圆柱的高度和半径之和为 7 厘米,则求圆柱的半径,使得总表面积为 440 平方厘米。
解决方案:
We have, (r + h) = 7 and A = 440.
Total surface area = 2πr(r + h)
440 = 2 (22/7) (r) (7)
2 (22) (r) = 440
44r = 440
r = 10 cm
问题 7. 如果圆柱的总表面积为 528 平方厘米,半径为 7 厘米,求圆柱的曲面面积。
解决方案:
We have, A = 528 and r = 7 cm.
Total surface area = 2πr(r + h)
528 = 2 (22/7) (7) (7 + h)
2 (22) (7 + h) = 528
7 + h = 12
h = 5 cm
Curved surface area of cylinder = 2πrh
= 2 (22/7) (7) (5)
= 2 (22) (5)
= 220 sq. cm