📌  相关文章
📜  毕奥-萨伐法则

📅  最后修改于: 2022-05-13 01:57:37.148000             🧑  作者: Mango

毕奥-萨伐法则

Biot-Savart 方程表示由载流导线产生的磁场。该导体或导线表示为称为电流元素的矢量。让我们详细看一下biot-savart定律的规律和公式，

毕奥-萨伐法则

定律中规定了由载流导体的微小元素引起的某个地方的磁感应强度。

  • 与电流成正比
  • 与元素的长度成正比
  • 与元素和连接元素中心到该点的线之间的角度的正弦成正比，并且，
  • 与点到元素中心的距离的平方成反比

推导

考虑带有电流 (I) 和微小长度元件 (dl) 的任何形式的导体（参见下图）。电流垂直向上描绘。

令 (P) 为距载流元素 (r) 距离的任意点， (r) 为 (P) 相对于当前元素的位置向量， 为 (dl) 和 (r) 之间的角度，在电流的方向。

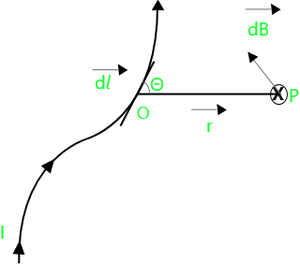
比奥——萨瓦特定律

根据 Biot – Savart 定律，点 (P) 处的磁感应由下式给出，

这里，K 是常数，它的值取决于单位系统以及导体所在的介质。

在 SI 系统中，真空或空气的常数 K 写为 [μ o / 4π] 其中 μ 0称为真空或自由空间的渗透率。

将 K 代入等式 (1) 我们得到，

磁感应的方向垂直于图的平面并指向平面内。 （根据右手拇指规则。）

以矢量形式，

其中 [r] 是从元素中心到该点的向量，[dl] 是元素在电流方向上的长度。通过添加所有此类元素 [dl] 的贡献，可以找到由整个导体引起的点 P 处的总磁感应，并表示为：

\vec B =\sum \vec {dB} = \frac{\mu_0}{4\pi}\sum I_{dl\rightarrow 0} \frac {\vec {dl}\times \vec r}{r^3}

\vec B = \frac{\mu_0}{4\pi}\sum I_{dl\rightarrow 0} \frac {d\vec l\times \vec r}{r^3}

求和替换为∫

现在，由于无限长且直的导体在距导体距离为“a”的点处载有电流 I 的磁感应强度由下式给出，

Biot – savart 定律的应用

以下是 Biot-Savart 定律的一些重要性：

  • 我们可以利用 Biot-Savart 定律来确定原子或分子水平的磁响应。
  • 它可以在空气动力学理论中用于确定涡线促进的速度。
  • 该规则可用于计算电流元件产生的磁场。

Biot-Savart 定律的重要性

以下是 Biot-Savart 定律的一些重要性：

  • 在静电学中，Biot-Savart 定律类似于库仑定律。
  • 该立法也适用于传输电流的极小导体。

概念问题

问题 1：State Biot – savart 定律并将其写成向量形式。

回答：

问题 2：陈述 Biot savart 定律的任意两个应用。

回答：

示例问题

问题 1：沿南北方向保持的导线中，有 5A 的电流从南流向北。求一根 1cm 长的电线在距电线东北 2m 处产生的磁场。

解决方案：

问题 2：一根长直导线载有 35A 的电流。距离导线 20cm 处的磁场 B 是多少？

解决方案：

问题3：水平面的一根长直导线在南北方向上承载50A的电流。在导线以东 3m 处给出 B 的大小和方向。

解决方案：

问题4：真空中两条长直平行线相距4m，同向分别承载6A和8A电流。找到中性点，即合成磁感应为零的点。

解决方案：