查找可以放置在大小为n * m的矩形内的大小为2 * 1的矩形的数量

给定两个整数$n$ ， $m$ 。找到大小为2 * 1的矩形数，可以将其放置在大小为n * m的矩形内。

笔记：

1. No two small rectangles overlap.
2. Each small rectangle lies entirely inside the large rectangle. It is allowed to touch the edges of the large rectangle.

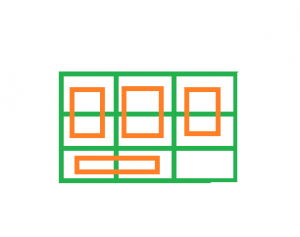
例子：

Input : n = 3, m =3
Output : 4

Input : n = 2, m = 4
Output : 4

方法：

1. 如果N是偶数，则放置M行N / 2个小矩形，并覆盖整个大矩形。
2. 如果M为偶数，则放置N行M / 2个小矩形，并覆盖整个大矩形。
3. 如果两者都为奇数，则用小矩形覆盖N – 1行的木板，并将floor(M / 2)小矩形置于最后一行。在最坏的情况下(N和M为奇数)，一个单元仍未被发现。

下面是上述方法的实现：

C++

// CPP program to Find the number of
// rectangles of size 2*1 can be placed
// inside a rectangle of size n*m
#include 
using namespace std;
  
// function to Find the number of
// rectangles of size 2*1 can be placed
// inside a rectangle of size n*m
int NumberOfRectangles(int n, int m)
{
    // if n is even
    if (n % 2 == 0)
        return (n / 2) * m;
  
    // if m is even
    else if (m % 2 == 0)
        return (m / 2) * n;
  
    // if both are odd
    return (n * m - 1) / 2;
}
  
// Driver code
int main()
{
    int n = 3, m = 3;
  
    // function call
    cout << NumberOfRectangles(n, m);
  
    return 0;
}

Java

// Java program to Find the number of 
// rectangles of size 2*1 can be placed 
// inside a rectangle of size n*m 
  
public class GFG {
      
    // function to Find the number of 
    // rectangles of size 2*1 can be placed 
    // inside a rectangle of size n*m 
    static int NumberOfRectangles(int n, int m) 
    { 
        // if n is even 
        if (n % 2 == 0) 
            return (n / 2) * m; 
        
        // if m is even 
        else if (m % 2 == 0) 
            return (m / 2) * n; 
        
        // if both are odd 
        return (n * m - 1) / 2; 
    } 
    public static void main(String args[])
    {
         int n = 3, m = 3; 
            
            // function call 
            System.out.println(NumberOfRectangles(n, m)); 
            
    }
    // This Code is contributed by ANKITRAI1
}

Python 3

# Python 3 program to Find the 
# number of rectangles of size
# 2*1 can be placed inside a
# rectangle of size n*m
  
# function to Find the number 
# of rectangles of size 2*1 
# can be placed inside a 
# rectangle of size n*m
def NumberOfRectangles(n, m):
  
    # if n is even
    if (n % 2 == 0):
        return (n / 2) * m
  
    # if m is even
    elif (m % 2 == 0):
        return (m // 2) * n
  
    # if both are odd
    return (n * m - 1) // 2
  
# Driver code
if __name__ == "__main__":
    n = 3
    m = 3
  
    # function call
    print(NumberOfRectangles(n, m))
  
# This code is contributed
# by ChitraNayal

C#

// C# program to Find the number of 
// rectangles of size 2*1 can be placed 
// inside a rectangle of size n*m 
using System;
  
class GFG 
{
      
// function to Find the number of 
// rectangles of size 2*1 can be placed 
// inside a rectangle of size n*m 
static int NumberOfRectangles(int n, int m) 
{ 
    // if n is even 
    if (n % 2 == 0) 
        return (n / 2) * m; 
  
    // if m is even 
    else if (m % 2 == 0) 
        return (m / 2) * n; 
  
    // if both are odd 
    return (n * m - 1) / 2; 
} 
  
// Driver Code
public static void Main()
{
    int n = 3, m = 3; 
  
    // function call 
    Console.WriteLine(NumberOfRectangles(n, m)); 
      
}
// This code is contributed 
// by Akanksha Rai(Abby_akku)
}

输出：

4