算术级数是一个数字序列,其中任何两个连续数字之间的差是恒定的。例如
1、3、5、7、9……。在一个序列中,两个连续项之间的公有差(3-1)等于2。如果我们以自然数作为序列1,2,3,4…的示例,则公有差(2-1)两个连续项之间的等于1。
换句话说,算术级数可以定义为“两个连续项之间的差始终为常数的数学序列”。
We come across the different words like sequence, series, and progression in AP, now let us see what does each word define –
- Sequence is a finite or infinite list of numbers that follows a certain pattern. For example 0, 1, 2, 3, 4, 5… is the sequence, which is infinite sequence of whole numbers.
- Series is the sum of the elements in which the sequence is corresponding For example 1 + 2 + 3 + 4 + 5….is the series of natural numbers. Each number in a sequence or a series is called a term. Here 1 is a term, 2 is a term, 3 is a term …….
- Progression is a sequence in which the general term can be expressed using a mathematical formula or the Sequence which uses a mathematical formula that can be defined as the progression.
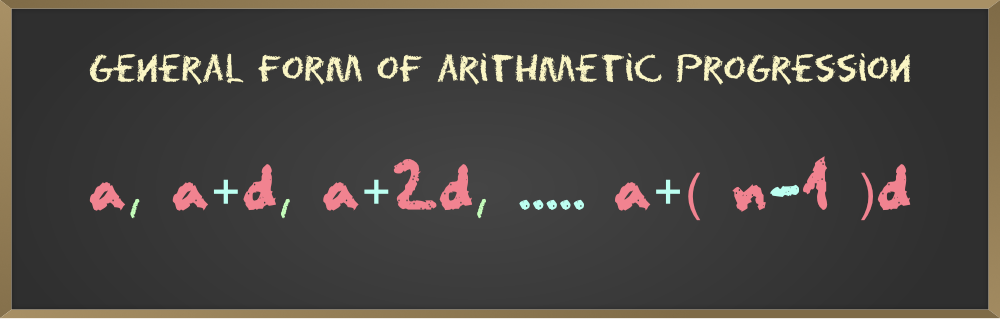
AP的共同区别是什么?
算术级数的共同差异用d表示。连续项与其前一项之间的差。对于算术级数,它始终是常数或相同。换句话说,我们可以说,在给定序列中,如果共同差异是恒定的或相同,那么我们可以说给定序列处于算术级数。
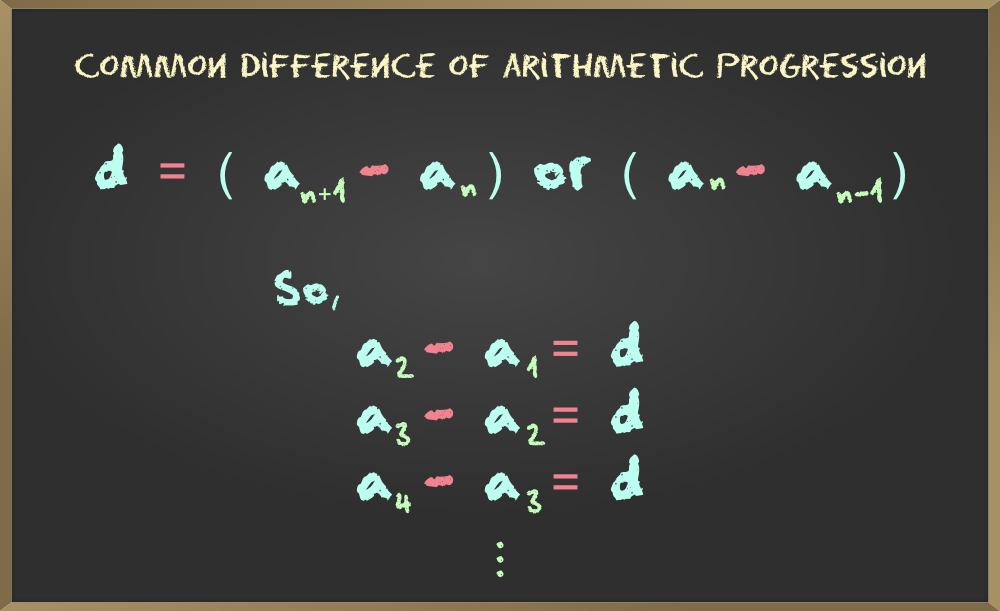
- 寻找共同差异的公式是d =(a n + 1 – a n )或d =(a n – a n-1 ) 。
- 如果共同差异为正,则AP会增加。对于这些系列中的示例4、8、12、16…..,AP增加
- 如果共同差异为负,则AP减小。对于示例-4,-6,-8 ….,此处AP减小。
- 如果公共差为零,则AP将为常数。对于示例1、2、3、4、5………,这里AP是恒定的。
差数列的序列会像A 1,A 2,A 3,A 4,…
示例1:0、5、10、15、20…..
here,
a1 = 0, a2 = 5, so a2 - a1 = d = 5 - 0 = 5.
a3 = 10, a2 = 5, so a3 - a2 = 10 - 5 = 5.
a4 = 15, a3 = 10, so a4 - a3 = 15 - 10 =5.
a5 =20, a4 =15, so a5 -a4 = 20 - 15 = 5.
从上面的示例中,我们可以说共同的区别是“ 5”。
范例2:0、7、14、21、28……。
here,
a1 = 0, a2 = 7, so a2 - a1 = 7 - 0 = 7
a3 = 14, a2 = 7, so a3 - a2 = 14 - 7 = 7
a4 = 21, a3 = 14, so a4 - a3 = 21 - 14 = 7
a5 =28, a4 = 21, so a5 -a4 =28 - 21 = 7
从上面的示例中,我们可以说共同的区别是“ 7”。
如何找到AP的中间术语?
为了找到算术级数的中间项,我们需要一个序列中的项总数。我们有两种情况:
Even: If the number of terms in the sequence is even then we will be having two middle terms i.e (n/2) and (n/2 + 1).n
Odd: If the number of terms in the sequence is odd then we will be having only one middle terms i.e (n/2).
范例1:
If n = 9 then,
Middle term = n/2 = 9/2 = 4.
范例2:
If n = 16 then,
First middle term = n/2 = 16/2 = 8.
Second middle term = (n/2) + 1 = (16/2) + 1 = 8 + 1 = 9.
AP的第N个术语是什么?
为了找到算术级数的第n个项,我们知道AP系列的形式为a,a + d,a + 2d,a + 3d,a + 4d………。
所述第术语n由T N表示。因此,找到一个AP系列的第n个项将是:

示例:找到给定AP序列的第9个项:3、6、9、12、15………..?
步骤1:编写给定的系列。
Given series = 3, 6, 9, 12, 15...........
步骤2 :现在记下给定序列中的a和n的值。
a = 3, n = 9
第3步:使用公式(a n + 1 -a n )找到共同的差异d。
d = a2 - a1 ,
here a2 = 6 and a1 = 3
so d = (6 - 3) = 3.
步骤4 :我们需要在公式(T n = a +(n – 1)d)中替换a,d,n的值。
Tn = a + (n - 1)d
given n = 9.
T9 = 3 + (9 - 1)3
= 3 + (8)3
= 3 + 24 = 27
因此,给定的AP系列3、6、9、12、15 ………的第9个术语。是“ 27”。