第10章RD Sharma解决方案-第14章坐标几何–练习14.1

问题1：以下几点位于哪条轴上?

(i)P(5，0)

解决方案：

As its ordinate is 0. So, it lies on x-axis.

(ii)Q(0，-2)

解决方案：

As its abscissa is 0. So, it lies on y-axis (negative half).

(iii)R(-4，0)

解决方案：

As its ordinate is 0. So, it lies on x-axis (negative half).

(iv)S(0，5)

解决方案：

As its abscissa is 0. So, it lies on y-axis.

问题2：让ABCD为面2a的平方。在以下情况下找到该正方形的顶点坐标：

(i)A与原点重合，AB和AB和坐标轴分别平行于边AB和AD。

(ii)正方形的中心在原点，坐标轴分别平行于AB和AD边。

解决方案：

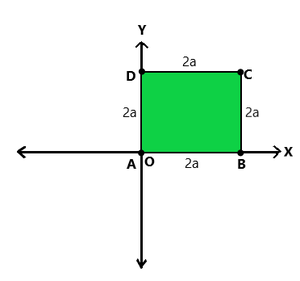
(一世)

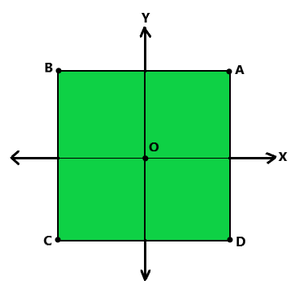
(二)

(i) Coordinate of the vertices of the square ABCD of side 2a will be – A(0, 0), B(2a, 0), C(2a, 2a) and D(0, 2a)

(ii) Coordinate of the vertices of the square ABCD of side 2a will be – A(a, a), B(-a, a), C(-a, -a) and D(a, -a)

问题3：边为2a的两个等边三角形PQR和PQR’的底边PQ沿y轴放置，以使PQ的中点位于原点。找到三角形的顶点R和R’的坐标。

解决方案：

Here, We have two equilateral triangles PQR and PQR’ with side 2a lying along y-axis.

O is the mid-point of PQ.

Now, in ∆QOR, ∠QOR = 90°

Now, By using Pythagoras theorem – 

OR² + OQ² = QR²

OR² = (2a)² – (a)²  

OR² = 3a²

OR = (√3)a

Thus, the coordinate of vertex R is (√3 a, 0) and coordinate of vertex R’ is (-√3 a, 0)