可简化为线性形式的方程

线性方程是一阶多项式。它可以包含一个或多个变量。例如，2x + 5，5x + 10y…etc是线性方程的一些示例。有时默认情况下，某些方程式本质上不是线性的，但可以将其转化为线性形式。

例如：

$\frac{7}{x} + \frac{3}{y} = 2$

上面给出的方程式不是线性方程式，但是可以通过一些重排和代数方法将其转换为线性形式。让我们详细看看它们。

可简化为线性形式的方程

让我们通过一个例子来看看这个过程。

假设我们采用上面给出的方程式$\frac{7}{x} + \frac{3}{y} = 2$ 。这个方程本质上不是线性的，但是如果我们决定替代

$p = \frac{1}{x} \text{ and } q = \frac{1}{y}$

然后这个等式变成

7p + 3q = 2

现在，可以使用“ p”和“ q”找出该方程的解，然后，我们可以根据先前的关系获得真实的解，

$p = \frac{1}{x} \text{ and } q = \frac{1}{y}$

问题1：将以下方程式简化为线性形式。

$\frac{2}{x - 3} + \frac{1}{y} = 1$

解决方案：

Like explained above, let’s say.

$p = \frac{1}{x -3}$ and $q = \frac{1}{y}$

Substituting these values in the equation, we get

2p + q = 1

现在让我们来看一个例子如何求解一对这样的方程，

问题2：求解给定的方程组

$\frac{2}{x - 4} + \frac{1}{y -1} = 1 \\ \text{ and } \\ \frac{5}{x - 4} + \frac{2}{y-1} = 3$

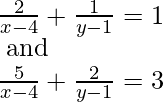
解决方案：

Let’s say, 

p = $\frac{1}{x - 4}$ and q = $\frac{1}{y - 1}$

Then both equations become, 

2p + q = 1  ……(1)

5p + 2q = 3 ……..(2)

We now have to solve these equations, 

From equation (1), 

q = 1 – 2p

Plugging this value in the equation (2) 

5p + 2(1 – 2p) = 3 

⇒5p + 2 – 4p = 3 

⇒p + 2 = 3 

⇒p = 1 

Then q = -1. 

Now let’s back the actual solutions by using the expressions we assumed in the beginning. 

p = $\frac{1}{x - 4}$  

⇒ 1 = $\frac{1}{x - 4}$

⇒ x – 4 = 1 

⇒ x = 5

Similarly, 

q = $\frac{1}{y - 1}$

⇒ -1 = $\frac{1}{y - 1}$

⇒ -y + 1 = 1 

⇒ y = 0

Thus, the solution to this system of equation is x = 5 and y = 0. 

单词问题

问题1：一艘船在20小时内向上游行驶60公里，向下游行驶88公里。在另一趟旅程中，它在26小时内向上游80公里，向下游110公里。找出溪流的速度和在静止水中乘船的速度。

解决方案：

Let’s assume that speed of boat in still water is “x Km/h” and speed of stream is “y Km/h”. 

So, speed of boat in downstream = x + y 

And speed of boat in upstream = x – y

We know that, time = $\frac{distance}{speed}$

So, for the first trip the equation will be, 

$20 = \frac{60}{x - y} + \frac{88}{x + y}$ 

Similarly, the equation for the second trip will be, 

$26 = \frac{80}{x - y} + \frac{110}{x + y}$

These equations now need to be reduced in a linear form, 

Let’s say p = $\frac{1}{x - y}$ and $q = \frac{1}{x + y}$

Substituting this in the above equations, we get 

20 = 60p + 88q 

26 = 80p + 110q 

Solving this with cross multiplication method, 

$\frac{p}{88(-26) - (110)(-20)} = \frac{q}{80(-20) - 60(-26)} = \frac{1}{(60(110) - (88)(80)} \\ \frac{p}{-22} = \frac{q}{-10} = \frac{1}{-110}$

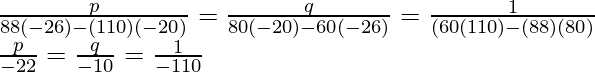
This gives us, 

p = $\frac{1}{5}$ and q =$\frac{1}{11}$

Now put these value of “p” and “q” in the equation, 

 $p = \frac{1}{x - y} \\ \frac{1}{5} = \frac{1}{x - y} \\ 5 = x - y$ 

 $q = \frac{1}{x + y} \\ \frac{1}{11} = \frac{1}{x + y} \\ x + y = 11$

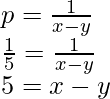
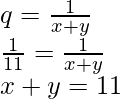
To get the values of “x” and “y” we will solve these equations, 

x – y = 5 ….. (1)

x + y = 11 ….. (2)

Putting the value of x = y + 5 

y + 5 + y = 11 

⇒2y = 6 

⇒y = 3

So, x = 8

Thus, the solution is x = 8 and y = 3. 

问题2：两个城市之间的距离是300Km。一个人必须同时乘坐公共汽车和火车来旅行。如果我们乘火车去60公里，旅行需要4个小时，其余的距离由公共汽车覆盖。如果乘火车旅行100公里，其余乘公共汽车旅行，则需要10分钟的时间。查找在两个城市之间运行的火车和公共汽车的速度。

解决方案：

Let the speed of bus be “x” and that of train be “y”. 

So total time taken, 

 $4 = \frac{60}{x} + \frac{240}{y}$

$4 + \frac{1}{6} = \frac{100}{x} + \frac{200}{y}$

Let’s say $p = \frac{1}{x} \text{ and } q = \frac{1}{y}$

Now the two equations become, 

4  = 60p + 240q 

$\frac{25}{6}$ = 100p + 200q

Solving these equations, 

The solution comes out to be 

$p = \frac{1}{60} \text{ and } q = \frac{1}{80}$

Computing the values of x and y from this, 

x = 60 and y = 80 

Thus, speed of the bus is 60Km/h and that of train is 80Km/h. 

问题3：拉梅什的年龄是儿子的两倍，四年前，儿子的年龄比父亲小20岁。找到他们当前的年龄。

解决方案：

Let the age of Ramesh be x and the age of his son is y

The equations obtained are,

y= 2x ⇢ 1

y-4 = (x-4) +20 ⇢ 2

2x- 4 = x-4 + 20

x = 20 years

问题4：求解以下等式，获得p和q的值，

1 /(2p)– 1 / q = 2

1 / p + 1 /(2q)= 10

解决方案：

As it is clearly seen that the above two equations are not in the form of linear equation as the reciprocal of p and q are given.

Simplifying,

(1/2)(1/p) – 1/q = 2

1/p + (1/2)(1/q) = 10

Let 1/p= x and 1/q = y

Now, the equations shall look like,

1/2(x) – y = 2

x + 1/2(y) = 10

Simplifying further to find the values of x and y,

x- 2y = 4     (1)

2x+ y= 20        (2)

Multiplying equation 2 with 2 in order to equate both equations

x- 2y= 4

4x+ 2y= 40

5x = 44

x= 44/5

y= 12/5

Therefore, p= 1/x= 5/44

q= 1/y= 5/12

问题5：有两个朋友，阿迪亚(Aditya)和阿曼(Aman)，他们俩都决定购买一些糖果和巧克力，他们俩共同花了卢比。在购买商品上有200个，但阿曼花了4倍于阿迪亚花了一半。计算两个朋友的确切消费金额。

解决方案：

Let the amount spent by both Aditya and Aman be a and b respectively,

Now, they both collectively spent 200 rupees

a+ b= 200  (1)

Aman spent 4 rupees more than half the aditya’s amount

a/2 +4 = b  (2)

Solving equation (2)

a+ 8= 2b

a= 2b-8

Putting the value of a in equation (1)

2b- 8 +b = 200

3b = 208

b= 208/3 = Rs. 69.7 ∼ Rs. 70

b= Rs. 70

Putting the value of b in equation (1)

a= Rs. 130