圆锥截面

我们了解基本曲线(例如直线)及其各种形式的方程。但是每天我们也会遇到不同类型的曲线，例如圆形，抛物线形，双曲线形等。这些曲线被称为圆锥截面，因为它们可以从圆锥体上切出。这些曲线具有非常广泛的应用范围，并且在自然界中也观察到很多。让我们看看如何将它们从圆锥体中切出，以及它们的方程式是什么。

圆锥截面

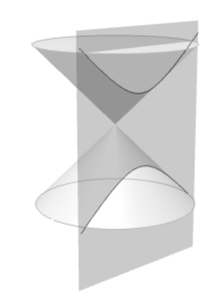
圆锥截面(也称为“圆锥形”)是与圆锥相交的平面。想象一下一个圆锥形，在不同的地方用刀将其切成不同类型的曲线，这就是所谓的“圆锥截面”。所获得的三个主要圆锥截面是抛物线，双曲线和椭圆形(“圆”可以称为椭圆的一种)。

假设我们采用固定的垂直线。我们称它为“ l”。现在，以与该线成恒定角度α的角度制作另一条线。

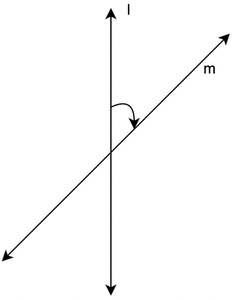
现在，如果我们开始通过保持角度相同来绕m旋转线m。我们将得到一个在两个方向上都延伸到无限大的圆锥体。

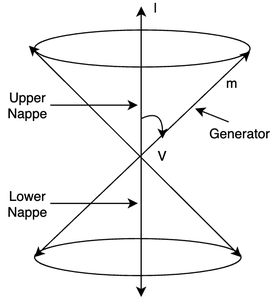
旋转线称为圆锥体的生成器。垂直线是圆锥的轴。 V是顶点，它将圆锥分成两个部分，称为纳普。

现在，当我们将生成的圆锥体与平面相交时，获得的截面称为圆锥截面。

此相交会根据与圆锥相交的平面的角度生成不同类型的曲线。

生成的圆锥截面

根据平面与圆锥相交的不同角度，可以找到不同类型的曲线。想象一下手中有一个冰淇淋蛋筒，从冰淇淋蛋筒的顶部看它会看起来像一个圆，因为倒置蛋筒的顶视图是一个圆，这得出一个结论，即用平面切割一个蛋筒精确地在90°处将提供一个圆。类似地，不同的角度将导致不同类型的曲线。

让我们看一下该平面与垂直轴成一个角度β。根据角度的值，可以有多个相交曲线。

1.如果β= 90°。我们得到一个圆圈。

2.如果β介于(α，90°)之间。我们得到一个椭圆。

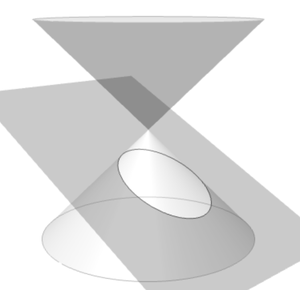
3.如果β=α。生成的形状称为抛物线。

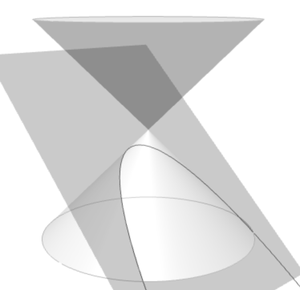
4.如果β在[0，α]区间内。生成的形状称为双曲线。

圆圈

当平面与圆锥成直角相交时，将生成一个圆。如上图所示，让我们看一下并以更数学的方式定义它，

A circle is a set of points which are equidistant from a fixed point. The fixed point is called centre of the circle and the distance is called radius. 

O是圆的中心，并且将中心连接到圆的线表示圆的半径。现在，让我们导出该圆的方程式。

圆方程

假设圆心由C(h，k)给出，其半径为“ r”。如果我们假设P(x，y)是圆上的任意点，则根据上述定义，P与C的距离必须为“ r”。

$|CP| = r \\ \sqrt{(x-h)^2 + (y - k)^2} = r \\ (x-h)^2 + (y - k)^2 = r^2$

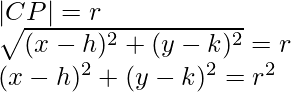
当给出中心和半径时，这是圆的方程。  

让我们来看一些关于这些概念的示例问题。

样本问题

问题1：找到以(0,0)为中心，半径为5的圆的方程。

解决方案：

We have studied the formula for the equation of the circle. 

(x-h)² + (y – k)² = r²

We just need to plug in the values in the formula. 

Here, h = 0, k = 0 and r = 5 

(x – 0)² + (y – 0)² = 5²

⇒x² + y² = 5²

⇒x² + y² = 25

问题2：找到中心为(-4，5)，半径为4的圆的方程。

解决方案：

The formula for the equation of the circle. 

(x-h)² + (y – k)² = r²

We just need to plug in the values in the formula. 

Here, h = -4, k = 5 and r = 4 

(x – (-4))² + (y – 5)² = 5²

⇒(x+ 4)² + (y – 5)² = 25

⇒x² + 16 + 8x + y² + 25 – 10y = 25

⇒x² + 8x + y² -10y + 16= 0

问题3：下面给出的方程是圆的方程，求出半径和中心。

x ² + 6x + y ² – 4y = 3

解决方案：

We are given the equation, now to find out the radius and the centre. We need to rearrange the equation such that this equation can come in the form given below. 

(x-h)² + (y – k)² = r²

x² + 6x + y² – 4y = 3

⇒ x² + (2)(3)x + y² – 2(2)y = 3 

We can see that these equations can be separated into two perfect squares. 

⇒ x² + (2)(3)x + 9 – 9 + y² – 2(2)y + 4 – 4 = 3 

⇒ (x + 3)² – 9 + (y – 2)² – 4 = 3 

⇒ (x + 3)² + (y – 2)² = 3  + 4 + 9 

⇒ (x + 3)² + (y – 2)² = 16

⇒ (x + 3)² + (y – 2)² = 4²

Now comparing this equation with the standard equation of the circle, we notice, 

h = -3, k = 2 and radius = 4. 

问题4：找到圆的方程式，其中心为(-h，-k)和半径$\sqrt{h^2 + k^2}$

解决方案：

The standard equation of the circle is given by, 

(x-h)² + (y – k)² = r²

Here, we have h = -h and k = -k and radius = $\sqrt{h^2 + k^2}$. Putting these values into the equation. 

$(x + h)^2 + (y + k)^2 = (\sqrt{h^2 + k^2})^2 \\ x^2 + h^2 + 2xh + y^2 + k^2 + 2yk = h^2 + k^2 \\ x^2 + 2xh + y^2 + 2yk = 0$

问题5：假设给了x + y = 2的直线，并通过了点(2，-2)和(3,4)的圆。还假定圆的中心位于直线上。找出圆的半径和中心。

解决方案：

Let’s say the equation of the circle is, 

(x-h)² + (y – k)² = r²

Now we know that the centre of the circle lies on the line x + y = 2. Since the centre of the circle is (h, k), it should satisfy this line. 

h + k = 2

Putting the value of h from this equation into the equation of the circle. 

(x-(2 – k))² + (y – k)² = r²

Now we also know that the circle satisfies the points (2, -2) and (3,4). Putting (2,-2) in the above equation.

(2-(2 – k))² + (-2 – k)² = r²

⇒ k² + (k + 2)² = r²

⇒ k² + k²  + 4 + 4k = r²

⇒ 2k² + 4 + 4k = r² …..(1)

Putting the equation (3,4) is, 

(x-(2 – k))² + (y – k)² = r²

⇒ (3-(2 – k))² + (4 – k)² = r²

⇒(1 – k)² + (4 – k)² = r²

⇒ k² -2k + 1 + 16 -8k + k² =r²

⇒ 2k² -10k + 17 =r² ……(2)

Solving these equations we get, 

h = 0.7, k = 1.7 and r² = 12.58