从等式确定圆锥截面

圆锥截面是当平面以不同角度与右圆锥相交时生成的曲线。圆锥截面的名称中具有定义，它是通过切割圆锥体而形成的截面。每种类型的交点都提供不同类型的曲线。根据平面相交的角度，曲线的形状会发生变化，并形成不同的曲线，例如抛物线，双曲线，椭圆形等。

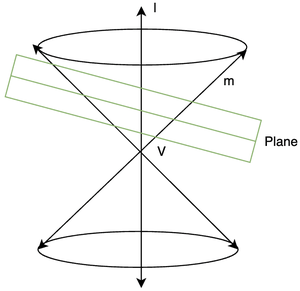
我们可以从相交处获得以下曲线：

1. 圆圈
2. 抛物线
3. 双曲线
4. 椭圆

每条曲线的方程式都有某些共同的特征。让我们研究它们中的每一个，看看如何从它们的方程式中识别它们。

圆圈

Circle is set of points which are equidistant from a fixed point. 

下图表示一个圆，其圆心由O给出，半径是将圆心连接到圆上任何点的线。

其等式由下式给出：

(x – h) ² +(y – k) ² = r ²

其中(h，k)是圆的中心，并且半径由“ r”给出。可以重新排列此等式以使其具有以下形式：

x ² + y ² -2hx -2kx + h ² + k ² = r ²

从方程中识别圆

一个圆在其方程式中将同时具有x和y平方，且系数均为非零。 x ²和y ²必须具有相同的符号。一旦我们知道它是圆的方程，就可以恢复曲线。

例如：

The equation given is, 4x²+ 4y²+ 7y= 9, The easiest way to identify when the given equation is the equation of a circle,

1. Both squares of x and y are present in the equation.

2. The coefficients of the squares of x and y are same (here, +4).

These two information tells that the provided curve’s equation is a Circle.

抛物线

A parabola is the set of all points in a plane that are equidistant from a fixed line and a fixed point (not on the line) in the plane

固定线称为Directrix，点称为焦点。

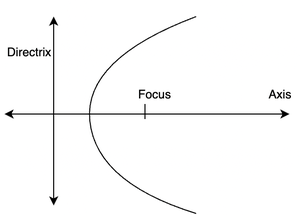
抛物线的标准方程式

以x轴为轴，经过(a，0)的原点和焦点的标准抛物线方程，由下式给出：

y ² = 4ax

下图显示了不同类型的抛物线及其方程式。

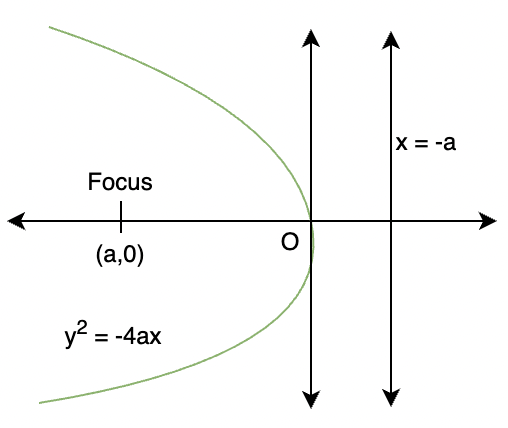
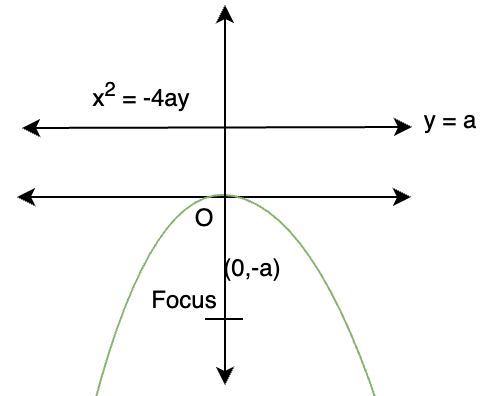
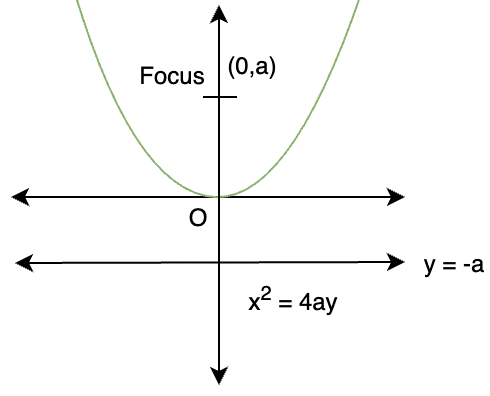
从方程式中识别抛物线

从展开方程中识别抛物线方程。我们只需要注意一件事。抛物线方程应具有y或x平方，但不能同时具有两者。其余的值可以是任何值。如果方程式有x或y平方，但不是两个都平方。然后可以以抛物线的标准方程式的形式对其进行重新排列。

例如：

Take a look at these equations,

x²= y+ 4, y²– 3x+ 9= 0

Both the equations mentioned above are a Parabola because,

1. Both equations have one of the variables squared, but not both. Therefore, the equations are parabola.

椭圆

An ellipse is a set of all the points in a plane, the sum of whose distances from two fixed points in a plane is constant. 

上面定义中提到的两点称为椭圆的焦点。

以x轴为长轴，y轴为短轴的椭圆的标准方程由下式给出：

$\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$

其中，c ² = a ² – b ²

如果椭圆的长轴在y轴上，依此类推。然后，等式由下式给出：

$\frac{x^2}{b^2} + \frac{y^2}{a^2} = 1$

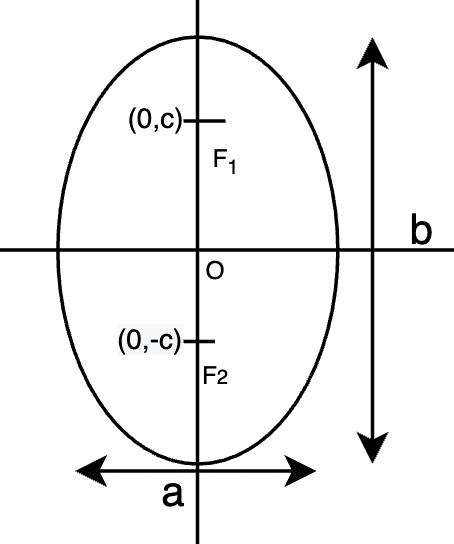
从方程式中识别椭圆：与圆形相似，椭圆也具有x和y正方形。但是不同之处在于它们将具有不同的系数。

例如：

5x²+ 7y²– 9x- 6y = 0, 9y²+ 2y²+ 8x+ y= 0

Both the equations are the curves of ellipse because,

1. Both x and y variables are squared.

2. The coefficients of both squared variables are different in values (if the coefficients were equal, the curve would be a Circle)

双曲线

Hyperbola is a set of points in a plane, the difference of whose distances from two fixed points in the plane is a constant. 

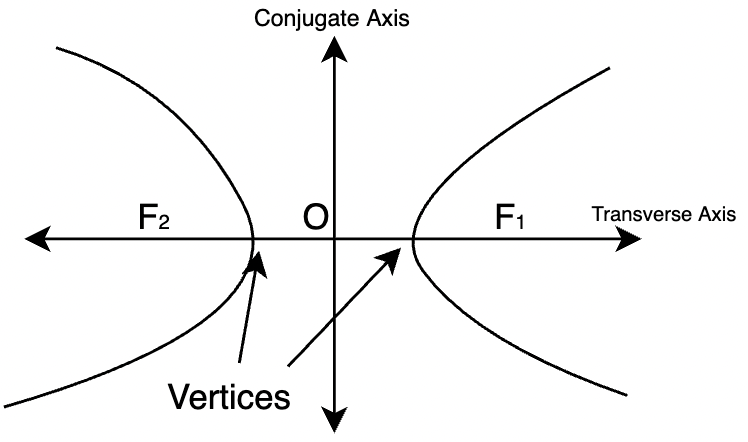
双曲线的标准方程式为

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

同样在这里，c ² = a ² – b ²

在上述情况下，横轴是x轴，共轭轴是y轴。如果轴反转，则方程将变为

$\frac{x^2}{b^2} - \frac{y^2}{a^2} = 1$

从方程式中识别双曲线：与圆相似，双曲线也具有x和y平方。但是不同之处在于它们将具有不同的系数，并且符号将相反。

例如：

5x²– 2y²+ 7x= 8

The above equation is the equation of a Hyperbola since,

1. Both x and y with a degree 2 present.

2. one of the squared variables have different sign than other, in this case y² coefficient is negative and x² coefficient is positive.

让我们看一下有关这些概念的一些示例问题

样本问题

问题1：从扩展方程中识别曲线。

y ² -4y + 2 = 12x

解决方案：

y² – 4y + 2 = 12x

This equation contains only y square. We have seen in the previous sections that the parabola equations have either x or y squared. So, this must be the equation of the parabola. 

Rearranging the given equation, 

y² – 4y + 2 = 12x

⇒ y² – 4y + 4 + 2 – 4 = 12x 

⇒(y – 2)² – 2 =12x 

⇒(y – 2)² = 12x + 2 

⇒$(y - 2)^2= 12(x - \frac{1}{6})$

问题2：从扩展方程中识别曲线：

4x ² + 9y ² = 36

解决方案：

4x² + 9y² = 36

The given equation has both x and y squares present in it. Both have positive but different coefficients. So, it might be an equation of ellipse. 

$4x^2 + 9y^2 = 36 \\ \frac{4x^2}{36} + \frac{9y^2}{36} = 1 \\ \frac{x^2}{9} + \frac{y^2}{4} = 1 \\ \frac{x^2}{3^2} + \frac{y^2}{2^2} = 1$

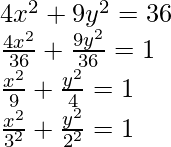
So, this is the equation with a = 3 and b = 2. 

问题3：根据扩展方程确定曲线：

7x ² – 9y ² = 36

解决方案：

7x² – 9y² = 36

This equation also has squares of both x and y, but the signs are different. Based on the above-mentioned method, we can say that this is a hyperbola. 

Now we need to bring the expanded equation in standard form. 

$7x^2 - 9y^2 = 36 \\ = \frac{7x^2}{36} -\frac{9y^2}{36} = 1 \\ = \frac{x^2}{\frac{36}{7}} - \frac{y^2}{\frac{36}{9}} = 1 \\ = \frac{x^2}{(\frac{6}{\sqrt{7}})^2} - \frac{y^2}{2^2} = 1 \\$

Here, a = $\frac{6}{\sqrt{7}}$and b = 2

问题4：给定曲线的扩展方程式，将其识别出来，然后将其恢复为标准形式。

x ² + y ² + 6y = 27

解决方案：

x² + y² + 6y = 27

Both x and y squares are present and have same sign and 1 as their coefficient. This is an equation of a circle. 

x² + y² + 6y = 27

⇒ x² + y² + 6y + 9 = 27 + 9 

⇒ x² + (y + 3)² = 36 

⇒ x² + (y + 3)² = 6²

This is the equation of circle with centre at (0,-3) and radius 6.  

问题5：根据给定的表达式确定曲线并公式化方程。

x ² + y ² + 4x + 6x = 12

解决方案：

Let’s take the given equation,

x² + y² + 4x + 6x = 12

From the equations we have studied above, notice that in the given equation x and y both are squared and both have the same sign and same coefficients. As mentioned previously, equations of circle should have both x and y squares with same sign and coefficients. Thus, this is the equation of the circle. 

To find the centre and the radius of the circle, we need to rearrange it. 

x² + y² + 4x + 6x = 12

We need to make whole squares out of the x and y terms 

x² + 4x + y² + 6x = 12

⇒ x² + 4x + 4 – 4 + y² + 6x + 9 – 9 = 12

⇒ (x + 2)² -4 + (y + 3)² – 9 = 12 

⇒ (x + 2)² + (y + 3)² = 12 + 9 + 4 

⇒(x + 2)² + (y + 3)² = 25 

⇒(x + 2)² + (y + 3)² = 5²

So, the centre is (-2,-3) and the radius is 5.