证明:
问题1:罪2  + cos 2
+ cos 2 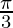 –棕褐色2
–棕褐色2 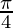 =
= 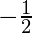
解决方案:
Taking LHS in consideration, we get
= sin2![]() +cos2
+cos2![]() – tan2
– tan2![]()
Substituting the values,
=![]()
=![]() – 1
– 1
=![]()
Hence, LHS = RHS
问题2:2sin 2 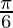 +安全2
+安全2 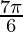 cos 2
cos 2 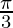 =
= 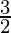
解决方案:
Taking LHS in consideration, we get
= 2sin2![]() +cosec2
+cosec2![]() cos2
cos2![]()
= 2sin2![]() + (- cosec
+ (- cosec![]() cos2
cos2![]()
Substituting the values,
=![]()
= 2![]() + 4
+ 4![]()
=![]() + 1
+ 1
=![]()
Hence, LHS = RHS
问题3:婴儿床2 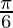 + cosec
+ cosec 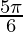 + 3tan 2
+ 3tan 2 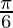 = 6
= 6
解决方案:
Taking LHS in consideration, we get
= cot2![]() + cosec
+ cosec![]() + 3tan2
+ 3tan2![]()
= cot2![]() + cosec
+ cosec![]() + 3tan2
+ 3tan2![]()
Substituting the values,
= (√3)2 + 2 + 3![]()
= 3 + 2 + 3![]()
= 6
Hence, LHS = RHS
问题4:2sin 2 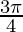 + 2cos 2
+ 2cos 2  + 2秒2
+ 2秒2 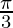 = 10
= 10
解决方案:
Taking LHS in consideration, we get
= 2sin2![]() + 2cos2
+ 2cos2![]() + 2sec2
+ 2sec2![]()
= 2sin2![]() + 2cos2
+ 2cos2![]() + 2sec2
+ 2sec2![]()
Substituting the values,
=![]()
= 2![]() + 2(4)
+ 2(4)
= 1 + 1 + 8
= 10
Hence, LHS = RHS
问题5:查找以下项的值:
(i)罪恶75度
解决方案:
As, we don’t know the angle value for 75°, so we will break into the angles which we know.
75° = 30° + 45°, so lets use this and solve for sin(30° + 45°)
Using the trigonometric formula,
sin (A+B) = sin A cos B + cos A sin B
sin(30° + 45°) = sin 30° cos 45° + cos 30° sin 45°
Substituting values, we get
sin(75°) =![]()
sin(75°) =![]()
sin(75°) =![]()
(ii)棕褐色15°
解决方案:
As, we don’t know the angle value for 15°, so we will break into the angles which we know.
15° = 60° – 45° or 45° – 30° so lets use this and solve for tan(45° – 30°)
Using the trigonometric formula,
tan (A-B) =![]()
tan(45° – 30°) =![]()
Substituting values, we get
tan(15°) =
tan(15°) =
tan(15°) =![]()
Now rationalizing the denominator, multiply and divide by![]()
tan(15°) =![]()
tan(15°) =![]()
tan(15°) =![]()
tan(15°) =![]()
tan(15°) =![]()
tan(15°) = 2 –![]()
证明以下内容:
问题6: 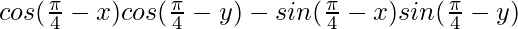 =罪(x + y)
=罪(x + y)
解决方案:
Taking LHS in consideration, we get
=![]()
As, here there is multiplication of cos cos and sin sin, we will use Defactorisation Formulae,
2 cos A cos B = cos (A+B) + cos (A-B) and, ……………….(1)
2 sin A sin B = cos (A-B) – cos (A+B) ……………….(2)
Multiply and divide LHS by 2, we get
=![]()
=![]()
Subtracting (2) from (1) and using identity formulae, we get
2 cos A cos B – 2 sin A sin B = cos (A+B) + cos (A-B) – (cos (A-B) – cos (A+B))
2 cos A cos B – 2 sin A sin B = 2 cos (A+B)
Hence, using this
=![]()
= cos![]()
= cos![]()
= sin (x+y) (As cos![]() = sin θ)
= sin θ)
Hence, LHS = RHS
问题7: 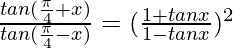
解决方案:
Taking LHS in consideration, we get
![]()
As, using Factorisation Formulae of tan, we have
tan (A+B) =![]() and,
and,
tan (A-B) =![]()
Now, substituting the values
=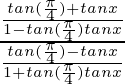
=
=![]()
=![]()
Hence, LHS = RHS
问题8: 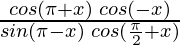 =婴儿床2 x
=婴儿床2 x
解决方案:
Taking LHS in consideration, we get
=![]()
As, we know these standard values
cos(-x) = cos x
cos![]() = – cos x
= – cos x
sin![]() = sin x
= sin x
cos![]() = – sin x
= – sin x
Substituting these values, we have
=![]()
=![]()
= cot2 x
Hence, LHS = RHS
问题9: ![由QuickLaTeX.com渲染 cos (\frac{3\pi}{2}+x)\hspace{0.1cm}cos (2\pi+x)[\hspace{0.1cm}cot (\frac{3\pi}{2}-x)+cot(2\pi+x)]](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions-%20Chapter%203%20Trigonometric%20Function%20%E2%80%93%20Exercise%203.3%20%7C%20Set%201_85.jpg) = 1
= 1
解决方案:
Taking LHS in consideration, we get
=![]()
As, we know these standard values
cos![]() = sin x
= sin x
cos![]() = cos x
= cos x
cot![]() = cot x
= cot x
cot![]() = tan x
= tan x
Substituting the values, we have
=![]()
=![]()
=![]()
As sin2 x + cos2 x = 1
= 1
Hence, LHS = RHS
问题10:sin(n + 1)x sin(n + 2)x + cos(n +1)x cos(n + 2)x = cos x
解决方案:
Taking LHS in consideration, we get
= sin(n + 1)x sin(n + 2)x + cos(n + 1)x cos(n + 2)x
As, here there is multiplication of cos cos and sin sin, we will use Defactorisation Formulae,
2 cos A cos B = cos (A+B) + cos (A-B) and, ……………….(1)
2 sin A sin B = cos (A-B) – cos (A+B) ……………….(2)
Multiply and divide LHS by 2, we get
=![]() (sin(n + 1)x sin(n + 2)x + cos(n + 1)x cos(n + 2)x)
(sin(n + 1)x sin(n + 2)x + cos(n + 1)x cos(n + 2)x)
=![]() (2 sin(n + 1)x sin(n + 2)x + 2 cos(n + 1)x cos(n + 2)x)
(2 sin(n + 1)x sin(n + 2)x + 2 cos(n + 1)x cos(n + 2)x)
Adding (1) and (2) and using identity formulae, we get
2 cos A cos B + 2 sin A sin B = cos (A+B) + cos (A-B) + cos (A-B) – cos (A+B)
2 cos A cos B + 2 sin A sin B = 2 cos (A-B)
Hence, using this
=![]() (2 cos((n + 1)x – (n + 2)x))
(2 cos((n + 1)x – (n + 2)x))
= cos((n + 1)x – (n + 2)x)
= cos (x-2x)
= cos (- x)
= cos x (As, cos(-x) = cos x)
Hence, LHS = RHS
问题11: 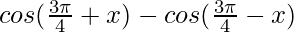 = –
= – 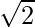 罪恶x
罪恶x
解决方案:
Taking LHS in consideration, we get
=![]()
Using the identity,
cos A – cos B = 2 sin![]() sin
sin![]()
Substituting the values,
=![]()
= 2 sin![]() sin
sin![]()
= 2 sin![]() sin (-x)
sin (-x)
= 2 sin![]() sin (-x)
sin (-x)
= 2 ( sin![]() ) sin (-x)
) sin (-x)
= 2![]() (- sin x)
(- sin x)
=![]()
Rationalizing the denominator, by multiplying and dividing by![]()
=![]()
=![]()
=![]()
=![]() sin x
sin x
Hence, LHS = RHS
问题12:sin 2 6x – sin 2 4x = sin 2x sin 10x
解决方案:
Taking LHS in consideration, we get
= sin2 6x – sin2 4x
= sin 6x sin 6x – sin 4x sin 4x
As, here there is multiplication of sin sin, we will use Defactorisation Formulae,
2 sin A sin B = cos (A-B) – cos (A+B)
Multiply and divide LHS by 2, we get
=![]() (sin 6x sin 6x – sin 4x sin 4x)
(sin 6x sin 6x – sin 4x sin 4x)
=![]() (2 sin 6x sin 6x – 2 sin 4x sin 4x)
(2 sin 6x sin 6x – 2 sin 4x sin 4x)
Using the identity, we can simplify
=![]() [(cos(6x-6x) – cos(6x+6x)) – (cos(4x-4x) – cos(4x+4x))]
[(cos(6x-6x) – cos(6x+6x)) – (cos(4x-4x) – cos(4x+4x))]
=![]() [(cos(0) – cos(12x)) – (cos(0) – cos(8x))]
[(cos(0) – cos(12x)) – (cos(0) – cos(8x))]
=![]() [1 – cos(12x) – 1 + cos(8x)] (As, cos 0 = 1)
[1 – cos(12x) – 1 + cos(8x)] (As, cos 0 = 1)
=![]() [cos(8x) – cos (12x)]
[cos(8x) – cos (12x)]
Now, using the identity
cos A – cos B = 2 sin![]() sin
sin![]()
Substituting the values, we have
=![]()
= sin![]() sin
sin![]()
= sin (10 x) sin (2x)
Hence, LHS = RHS
问题13:cos 2 2x – cos 2 6x = sin 4x sin 8x
解决方案:
Taking LHS in consideration, we get
= cos2 2x – cos2 6x
= cos 2x cos 2x – cos 6x cos 6x
As, here there is multiplication of cos cos, we will use Defactorisation Formulae,
2 cos A cos B = cos (A+B) + cos (A-B)
Multiply and divide LHS by 2, we get
=![]() (cos 2x cos 2x – cos 6x cos 6x)
(cos 2x cos 2x – cos 6x cos 6x)
=![]() (2 cos 2x cos 2x – 2 cos 6x cos 6x)
(2 cos 2x cos 2x – 2 cos 6x cos 6x)
Using the identity, we can simplify
=![]() [(cos(2x+2x) + cos(2x-2x)) – (cos(6x+6x) + cos(6x-6x))]
[(cos(2x+2x) + cos(2x-2x)) – (cos(6x+6x) + cos(6x-6x))]
=![]() [(cos(2x+2x) + cos(0)) – (cos(6x+6x) + cos(0))]
[(cos(2x+2x) + cos(0)) – (cos(6x+6x) + cos(0))]
=![]() [(cos(4x) + 1 – cos(12x) – 1)] (As, cos 0 = 1)
[(cos(4x) + 1 – cos(12x) – 1)] (As, cos 0 = 1)
=![]() [cos(4x) – cos(12x)]
[cos(4x) – cos(12x)]
Now, using the identity
cos A – cos B = 2 sin![]() sin
sin![]()
Substituting the values, we have
=![]()
= sin![]() sin
sin![]()
= sin (8x) sin (4x)
Hence, LHS = RHS
问题14:sin 2x + 2 sin 4x + sin 6x = 4 cos 2 x sin 4x
解决方案:
Taking LHS in consideration, we get
sin 2x + 2 sin 4x + sin 6x
After rearranging, we have
= (sin 2x + sin 6x) + 2 sin 4x
Using the identity, we can simplify
sin A+ sin B = 2 sin![]() cos
cos![]()
= 2 sin![]() cos
cos![]() + 2 sin 4x
+ 2 sin 4x
= 2 sin![]() cos
cos![]() + 2 sin 4x
+ 2 sin 4x
= 2 sin (4x) cos (2x) + 2 sin 4x
Taking (2 sin 4x), we have
= 2 sin (4x) (cos (2x) + 1)
= 2 sin (4x) (2 cos2 x – 1 + 1) (As, cos 2θ = 2cos2 θ – 1)
= 2 sin (4x) (2 cos2 x)
= 4 sin (4x) cos2 x
Hence, LHS = RHS