9类NCERT解决方案-第11章构造–练习11.1

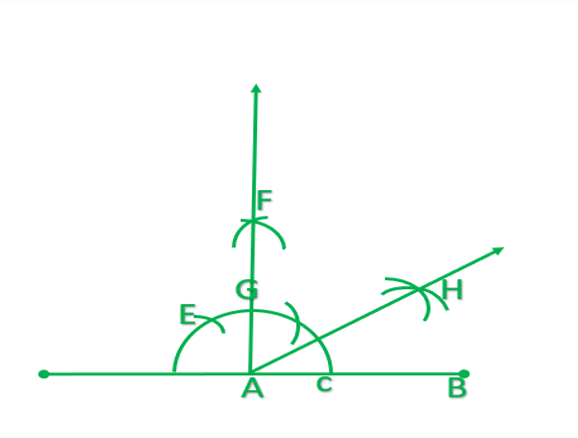
问题1.在给定射线的起始点构造一个90°的角，并证明该构造的合理性。

解决方案：

Steps of construction

1. Take a ray with initial point A.
2. Taking care center and same radius draw an Arc of a circle which intersect AB at C.
3. With C as Centre and the same radius, draw an arc intersecting the previous arc at E.
4. With E as Centre and the same radius draw an arc which intersects the arc drawn in step 2 at F.
5. With E as Centre and the same radius, draw another arc, intersecting the previous arc at G.
6. Draw the ray AG.
7. Then ∠BAG is the required angle 90°

Justification:

Join AE, CE, EF and AE, AF

AC = CE = AE      [ by construction]

∴ ACE is an equilateral Triangle

⇒ ∠CAE = 60°    —————–1

Similarly, AE = EF = AF

∴Triangle AEF is an equilateral Triangle

⇒ ∠EAF = 60°

Because AG bisects ⇒ ∠EAF

∴∠GAE = 1\2 = 30° = 30°————2

1+2

∴∠CAE + ∠GAE = 60°+30°

∠GAB=30°

问题2.在给定射线的起始点构造一个45°的角度以证明构造的合理性。

解决方案：

Step of Construction:

1. Take a ray AB with initial point A
2. Draw ∠BAF=90°
3. Taking C as Centre and radius more than   draw an arc.
4. Taking G as Centre and the same radius as before, draw another arc.
5. Taking G as Centre and the same radius as before, draw another arc. Intersecting previous arc at H.
6. Draw the ray AH.
7. Then ∠BAH is the required angle of 45°

Justification:

Join GH and HC    (construct)

In ∆ AHG and ∆ AHC

HG=HC…………….[arc of equal radii]

AG=AC……………..[radii of same arc]

AH=AH………………[common]

AHG≅AHC             [S.S.S]

∠HAG=∠HAC                [C.P.C.T]

But ∠HAG+∠HAC=90

∠HAG=∠HAC=90\2=45

∴∠BAH=45

问题3.画出以下测量的角度

i)30°

解决方案：

Step of construction

1. Draw a ray AB with initial point A.
2. With A as centre, draw an arc intersecting AB at c.
3. With c as centre and the same radius, draw another arc, intersecting the previously drawn arc at D.
4. Taking C and D as centre  and with the radius more than 1\2 DC draws arcs to intersect each other at E.
5. Draw ray AE. ∠EAB is the required angle of 30.

ii)22½°

解决方案：

Steps of construction

1. Take a ray AB
2. Draw an angle ∠AB=90° on point A.
3. Bisect ∠CAB and draw ∠DAB=45°
4. Bisect ∠DAB and draw ∠EAB
5. ∠EAB is required angle of 22 ½°

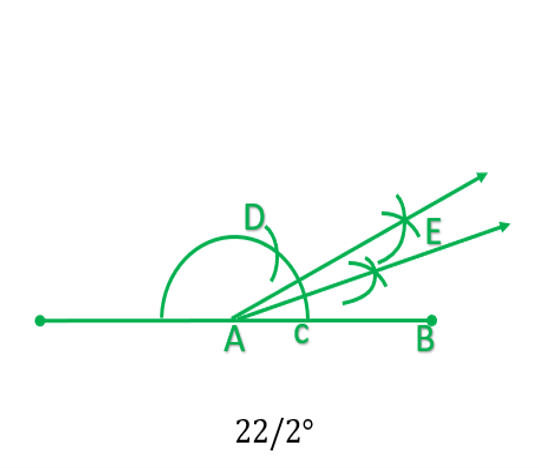
iii)15°

解决方案：

Steps of construction

1. Take a ray AB.
2. Draw an arc on AB, by taking A a center, which intersect AB at c.
3. From C with the same radius draw another re which intersect the previous  arc at D.
4. Join DA.
5. ∠DAB =60°
6. Bisect  ∠DAB and draw angle  EAB=30°
7. Bisect ∠EAB and draw ∠FAB
8. ∠FAB is the required angle.

  

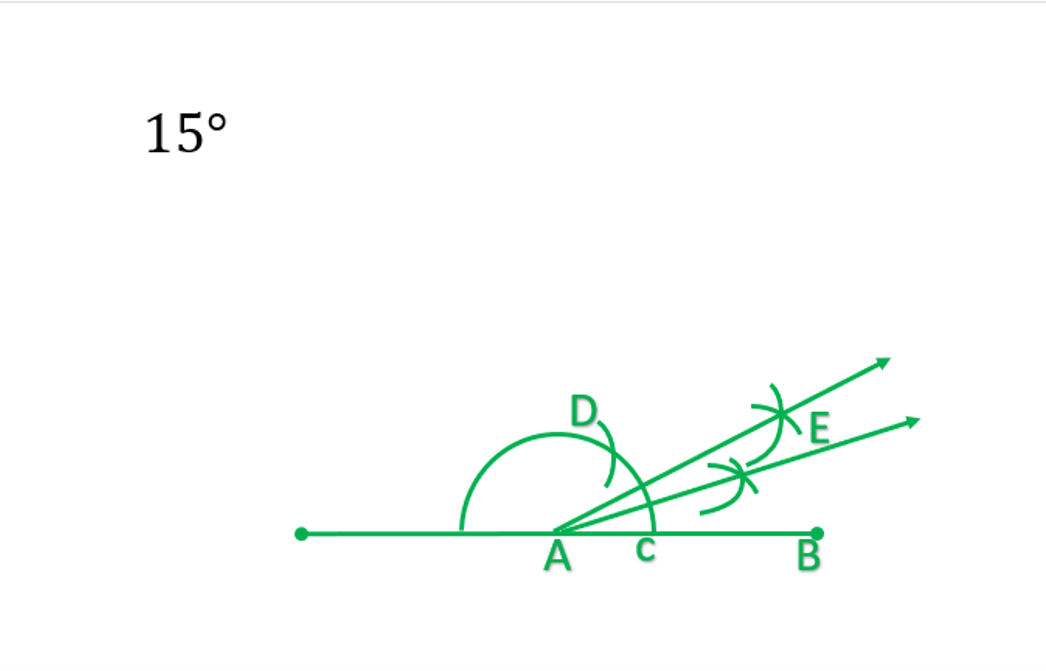
问题4.构造以下角度并通过量角器测量来验证

(i)75度

解决方案：

Steps of construction

1. Draw a ray AB with initial point  A.
2. At point A draw an angle ∠CAB=90°
3. At point A draw ∠DAB=60°
4. Bisect ∠CAD, now ∠EAD=15°
5. ∠EAB=75°        {∠EAB=∠EAD+∠DAB=15°+60°=75°}

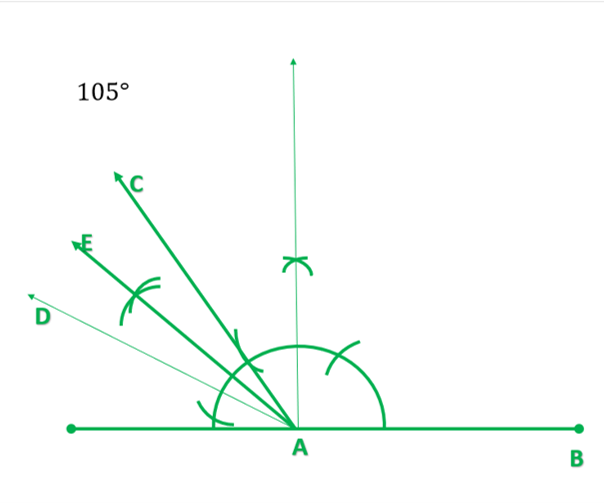
(ii)105度

解决方案：

Steps of construction

1. Draw a ray AB with initial point  A.
2. At point A draw an angle ∠CAB=90°
3. At point A draw ∠DAB=120°
4. Bisect ∠CAD, now ∠EAD=15°
5. ∠EAB=75°        {∠EAB=∠EAC+∠CAB=15°+90°=105°}

(iii)135度

解决方案：

Steps of construction

1. Draw a ray AB with initial point A.
2. At point A draw an angle ∠CAB=120°
3. At point A draw ∠DAB=150°
4. Bisect ∠CAD, now ∠EAC=15°
5. ∠EAB=135°        {∠EAB=∠EAC+∠CAB=15°+120°=135°}

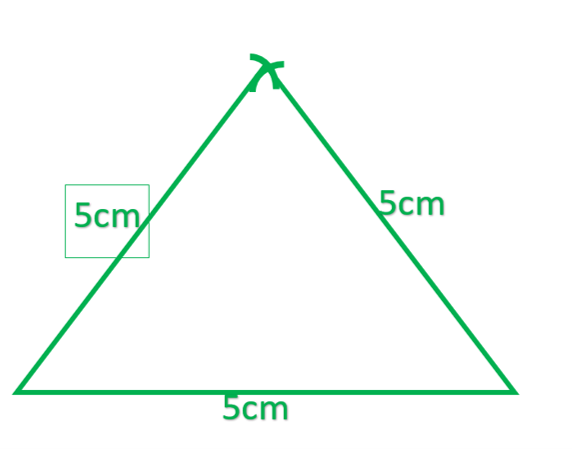
问题5.给定边的边构造一个等边三角形并证明其合理性。

解决方案：

Steps of construction

1. Draw a line segment of AB of a given length.
2. With A and B as centre and radius equal to AB draw arcs to intersect each other at c.
3. Join AC and BC.

Then ABC is the required equilateral triangle.

Justification:

AB=AC    ……………. [by construction]

AB=BC    ……………..[by construction]

AB=AC=BC

Hence, ∆ABC is required equilateral triangle.