双曲线

圆锥形部分用于日常生活中，从吉他，立交桥到足球。一切都有一条曲线，该曲线属于圆锥截面的曲线。圆锥截面有四种类型-圆形，抛物线形，椭圆形和双曲线形。双曲线和椭圆形是类似的圆锥截面。双曲线定义为由所有点集构成的曲线，这些点与平面中两个固定点的距离之差是恒定的。双曲线的一些示例是吉他的边界。让我们更详细地看一下曲线。

什么是双曲线?

双曲线定义为平面中的一组点，其与平面中两个固定点的距离之差是恒定的。下图显示了双曲线的基本形状及其不同的部分。我们有四个点P ₁ ，P ₂ ，P ₃和P ₄ 。我们测量每个点到F ₁和F ₂的距离之间的差。

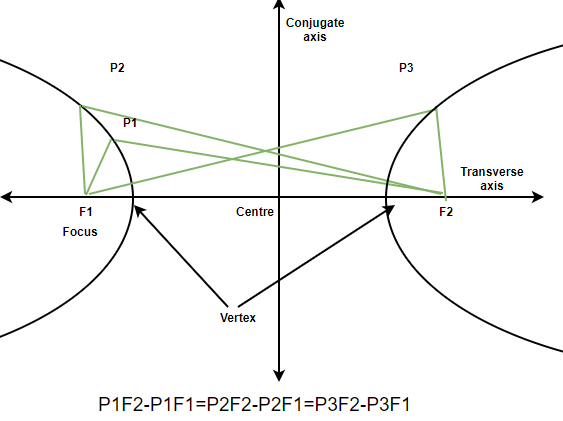
我们在定义双曲线时所讨论的距离之差是到较远点与较近点的距离之差。这两个点称为双曲线焦点。连接它们的线段的中点称为双曲线的中心。在给定的图中，穿过两个焦点的线称为双曲线的横轴，而垂直于横轴绘制的线称为共轭轴。抛物线的顶点定义为曲线与移动轴相交的点。

假设两个焦点之间的距离由“ 2c”给出，两个顶点之间的距离可以称为“ 2a”。让我们定义b，

b = $\sqrt{c^2 - a^2}$

The length of the conjugate axis is 2b. 

现在让我们计算P ₁ F ₁和P ₁ F ₂之间的差。考虑上图，我们在顶点处取了点A和B。我们知道，

BF₁ – BF₂ = AF₂ – AF₁

BA + AF₁ – BF₂ = AB + BF₂ – AF₁

AF₁ = BF₂

So that, BF₁ – BF₂ = BA + AF₁ – BF₂ = BA = 2a

偏心率

偏心率定义为“ c”与“ a”之比。我们知道c≥a，这就是为什么它的值在和0到1之间的原因。

$e = \frac{c}{a}$

Distance between foci in terms of eccentricity is given by 2ae. 

双曲线的标准方程式

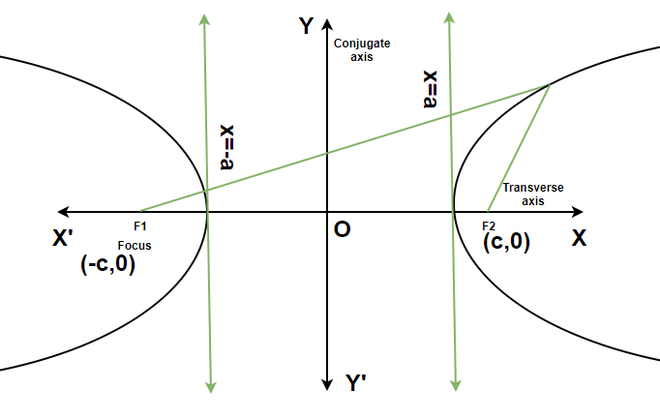
双曲线的标准方程有两种类型。通常，在标准方程式中，我们假设双曲线的中心在原点，而焦点分别在x和y轴上。下图显示了双曲线的标准方程式中的两种可能性。

让我们导出双曲线方程，

双曲线方程

下图显示了一个双曲线，其中心为原点，长轴为x轴。 F1和F2代表双曲线的焦点，比方说，我们在双曲线上的任意位置取一个点A(x，y)。

我们知道，A点到两个焦点的距离之差为“ 2a”。

AF ₁ – AF ₂ = 2a

让我们使用Euclid的距离公式替代距离的值。

$\sqrt{(x - c)^2 + y^2} - \sqrt{(x+c)^2 + y^2} = 2a$

= $\sqrt{(x - c)^2 + y^2} = 2a + \sqrt{(x+c)^2 + y^2}$

Squaring both sides, 

= $(\sqrt{(x - c)^2 + y^2})^2 = (2a + \sqrt{(x + c)^2 + y^2})^2$

= $(x - c)^2 + y^2 = 4a^2 + (x + c)^2 + y^2 + 2(2a)(\sqrt{(x + c)^2 + y^2})$

On Simplifying, 

= $\frac{cx}{a} - a = \sqrt{(x - c)^2 + y^2}$

Squaring again, 

= $\frac{x^2}{a^2} - \frac{y^2}{c^2 - a^2} = 1$

= $\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

Thus, this is the standard equation for hyperbola. 

直肠

这是一条垂直于移动轴并穿过焦点的线段。子宫直肠的端点位于双曲线和这条线之间的交点。

双曲线中的子宫直肠的长度由下式给出：  $\frac{2b}{a^2}$

样本问题

问题1：找到焦点在(2,0)和(-2,0)且顶点在(-1,0)和(1,0)处的双曲线方程。

解决方案：

Since, the foci lie on the x-axis. We know that the major axis of the hyperbola is x-axis only. So, it is of the form, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

Since the vertices lie at (-1,0) and (1,0), a = 1. 

c = $\frac{\text{distance between foci}}{2} = 2$

We know that, 

c² = a² + b²

⇒ 2² = 1 + b²

⇒ 3 = b²

⇒ b = √3 

So, the equation of the hyperbola becomes, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 \\ \frac{x^2}{1^2} - \frac{y^2}{\sqrt{3}^2} = 1 \\ x^2 - \frac{y^2}{3} = 1$

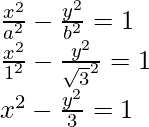
问题2：找到焦点在(4,0)和(-4,0)且顶点在(-1,0)和(1,0)处的双曲线方程。

解决方案：

Since, the foci lie on the x-axis. We know that the major axis of the hyperbola is x-axis only. So, it is of the form, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

Since the vertices lie at (-1,0) and (1,0), a = 1. 

c = $\frac{\text{distance between foci}}{2} = 4$

We know that, 

c² = a² + b²

⇒ 4² = 1 + b²

⇒ 15 = b²

⇒ b = √15 

So, the equation of the hyperbola becomes, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 \\ \frac{x^2}{1^2} - \frac{y^2}{\sqrt{15}^2} = 1 \\ x^2 - \frac{y^2}{15} = 1$

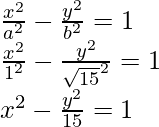
问题3：找到焦点在(12,0)和(-12,0)的双曲线方程，且直肠的长度为36。

解决方案：

Since, the foci lie on the x-axis. We know that the major axis of the hyperbola is x-axis only. So, it is of the form, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

 c = $\frac{\text{distance between foci}}{2} = 12$

We know that the length of latus rectum is 36. 

$\frac{2b^2}{a} = 36 \\ b^2 = 18a$

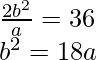
We know, 

c² = a² + b²

⇒ 12² = a² + 18a 

⇒0 = a² + 18a – 144

a = -24 and 6. 

So, value of a = 6 

From the above equations, 

b² = 18 × 6 

b = 6√3

So, the equation of the hyperbola becomes, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 \\ \frac{x^2}{6^2} - \frac{y^2}{({6\sqrt{3}})^2} = 1 \\ \frac{x^2}{36} - \frac{y^2}{108} = 1$

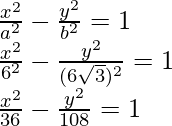
问题4：找到焦点在(6,0)和(-6,0)的双曲线方程，且直肠的长度为18。

解决方案：

Since, the foci lie on the x-axis. We know that the major axis of the hyperbola is x-axis only. So, it is of the form, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

 c = 6

We know that the length of latus rectum is 18. 

$\frac{2b^2}{a} = 18 \\ b^2 = 9a$

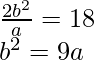
We know, 

c² = a² + b²

⇒ 6² = a² + 9a 

⇒0 = a² + 9a – 36

a = – 12 and 3. 

So, value of a = 3 

From the above equations, 

b² = 3 × 6 

b = 3√2

So, the equation of the hyperbola becomes, 

$\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 \\ \frac{x^2}{3^2} - \frac{y^2}{({3\sqrt{2}})^2} = 1 \\ \frac{x^2}{9} - \frac{y^2}{18} = 1$

问题5：找到给定双曲线的胎儿的中心，焦点和直肠的长度。

$\frac{x^2}{36} - \frac{y^2}{9} = 1$

解决方案：

We know that this is the standard form the equation for hyperbola, so the center lies at the origin. In this hyperbola, 

a = 6 and b = 3. 

Length of the latus rectum is given by, 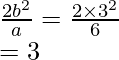$\frac{2b^2}{a} = \frac{2 \times 3^2}{6} \\ = 3$

c² = a² + b²

c² = 6² + 3²

c² = 36+ 9 

c = √45

The coordinates of foci are (c,0) and (-c,0) 

Thus, foci are (√45,0) and (-√45,0).