第 11 类 RD Sharma 解决方案 – 第 27 章双曲线 – 练习 27.1

问题 1. 双曲线准线方程为 x – y + 3 = 0。其焦点是 (-1, 1) 和偏心率 3. 求双曲线方程。

解决方案：

Given: Focus = (-1, 1) and Eccentricity = 3

The equation of the directrix of a hyperbola ⇒ x – y + 3 = 0.

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

We know, e = PF/PM ⇒ PF² = e²PM²

$⇒ (x+1)^2 + (y-1)^2 = 3^2[\frac{x-y+3}{\sqrt{1^2+(-1)^2}}]$

⇒ 2{x² + 1 + 2x + y² + 1 – 2y} = 9{x² + y²+ 9 + 6x – 6y – 2xy}

⇒ 2x² + 2 + 4x + 2y² + 2 – 4y = 9x² + 9y²+ 81 + 54x – 54y – 18xy

⇒ 2x² + 4 + 4x + 2y²– 4y – 9x² – 9y² – 81 – 54x + 54y + 18xy = 0

⇒ – 7x² – 7y² – 50x + 50y + 18xy – 77 = 0

⇒ 7(x² + y²) – 18xy + 50x – 50y + 77 = 0

∴The equation of hyperbola is 7(x² + y²) – 18xy + 50x – 50y + 77 = 0.

问题 2. 求双曲线方程

(i) 焦点是 (0, 3)，准线是 x + y – 1 = 0 和偏心率 = 2

解决方案：

Given: Focus = (0, 3), Directrix => x + y – 1 = 0 and Eccentricity = 2

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

We know, e = PF/PM ⇒ PF² = e²PM² 

$⇒ (x-0)^2 + (y-3)^2 = 2^2[\frac{x+y-1}{\sqrt{1^2+(1)^2}}]^2$

⇒ 2{x² + y² + 9 – 6y} = 4{x² + y² + 1 – 2x – 2y + 2xy}

⇒ 2x² + 2y² + 18 – 12y – 4x² – 4y² – 4 – 8x + 8y – 8xy = 0

⇒ – 2x² – 2y² – 8x – 4y – 8xy + 14 = 0

⇒ –2(x² + y² – 4x + 2y + 4xy – 7) = 0

⇒ x² + y² – 4x + 2y + 4xy – 7 = 0

∴The equation of hyperbola is x² + y² – 4x + 2y + 4xy – 7 = 0.

(ii) 焦点是 (1, 1)，准线是 3x + 4y + 8 = 0 和偏心率 = 2

解决方案：

Focus = (1, 1), Directrix => 3x + 4y + 8 = 0 and Eccentricity = 2

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

We know, e = PF/PM ⇒ PF² = e²PM²

$⇒ (x-1)^2 + (y-1)^2 = 2^2[\frac{3x+4y+8}{\sqrt{3^2+(4)^2}}]^2$

⇒ 25{x² + 1 – 2x + y² + 1 – 2y} = 4{9x² + 16y²+ 64 + 24xy + 64y + 48x}

⇒ 25x² + 25 – 50x + 25y² + 25 – 50y = 36x² + 64y² + 256 + 96xy + 256y + 192x

⇒ 25x² + 25 – 50x + 25y² + 25 – 50y – 36x² – 64y² – 256 – 96xy – 256y – 192x = 0

⇒ – 11x² – 39y² – 242x – 306y – 96xy – 206 = 0

⇒ 11x² + 96xy + 39y² + 242x + 306y + 206 = 0

∴The equation of hyperbola is 11x² + 96xy + 39y² + 242x + 306y + 206 = 0.

(iii) 焦点是 (1, 1) 准线是 2x + y = 1 和偏心率 = 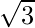$\sqrt{3}$

解决方案：

Given: Focus = (1, 1), Directrix => 2x + y = 1 and Eccentricity = $\sqrt{3}$

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

We know, e = PF/PM ⇒ PF² = e²PM² 

$⇒ (x-1)^2 + (y-1)^2 = (\sqrt{3})^2[\frac{2x+y-1}{\sqrt{2^2+(1)^2}}]^2$

⇒ 5{x² + 1 – 2x + y² + 1 – 2y} = 3{4x² + y²+ 1 + 4xy – 2y – 4x}

⇒ 5x² + 5 – 10x + 5y² + 5 – 10y = 12x² + 3y² + 3 + 12xy – 6y – 12x

⇒ 5x² + 5 – 10x + 5y² + 5 – 10y – 12x² – 3y² – 3 – 12xy + 6y + 12x = 0

⇒ – 7x² + 2y² + 2x – 4y – 12xy + 7 = 0

⇒ 7x² + 12xy – 2y² – 2x + 4y– 7 = 0

∴The equation of hyperbola is 7x² + 12xy – 2y² – 2x + 4y– 7 = 0.

(iv) 焦点是 (2, -1)，准线是 2x + 3y = 1，偏心率 = 2

解决方案：

Given: Focus = (2, -1), Directrix => 2x + 3y = 1 and Eccentricity = 2

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

By using the formula, e = PF/PM ⇒ PF² = e²PM²

$⇒ (x-2)^2 + (y+1)^2 = 2^2[\frac{2x+3y-1}{\sqrt{2^2+(3)^2}}]^2$

⇒ 13{x² + 4 – 4x + y² + 1 + 2y} = 4{4x² + 9y² + 1 + 12xy – 6y – 4x}

⇒ 13x² + 52 – 52x + 13y² + 13 + 26y = 16x² + 36y² + 4 + 48xy – 24y – 16x

⇒ 13x² + 52 – 52x + 13y² + 13 + 26y – 16x² – 36y² – 4 – 48xy + 24y + 16x = 0

⇒ – 3x² – 23y² – 36x + 50y – 48xy + 61 = 0

⇒ 3x² + 23y² + 48xy + 36x – 50y– 61 = 0

∴The equation of hyperbola is 3x² + 23y² + 48xy + 36x – 50y– 61 = 0.

(v) 焦点是 (a, 0)，准线是 2x + 3y = 1，偏心率 = 2

解决方案：

Given: Focus = (a, 0), Directrix => 2x + 3y = 1 and Eccentricity = 2

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

By using the formula, e = PF/PM ⇒ PF² = e²PM² 

$⇒ (x-a)^2 + (y-0)^2 = (4/3)^2[\frac{2x-y+a}{\sqrt{2^2+(-1)^2}}]^2$  

⇒ 45{x² + a² – 2ax + y2} = 16{4x² + y² + a² – 4xy – 2ay + 4ax}

⇒ 45x² + 45a² – 90ax + 45y² = 64x² + 16y² + 16a² – 64xy – 32ay + 64ax

⇒ 45x² + 45a² – 90ax + 45y² – 64x² – 16y² – 16a² + 64xy + 32ay – 64ax = 0

⇒ 19x² – 29y² + 154ax – 32ay – 64xy – 29a2 = 0

∴The equation of hyperbola is 19x² – 29y² + 154ax – 32ay – 64xy – 29a² = 0.

(vi) 焦点是 (2, 2)，准线是 x + y = 9，偏心率 = 2

解决方案：

Given: Focus = (2, 2), Directrix => x + y = 9 and Eccentricity = 2

Let ‘M’ be the point on directrix and P(x, y) be any point of the hyperbola.

By using the formula, e = PF/PM ⇒ PF² = e²PM²

$⇒ (x-2)^2 + (y-2)^2 = (2)^2[\frac{x+y-9}{\sqrt{1^2+(1)^2}}]^2$

⇒ x² + 4 – 4x + y² + 4 – 4y = 2{x² + y² + 81 + 2xy – 18y – 18x}

⇒ x² – 4x + y² + 8 – 4y = 2x² + 2y² + 162 + 4xy – 36y – 36x

⇒ x² – 4x + y² + 8 – 4y – 2x² – 2y² – 162 – 4xy + 36y + 36x = 0

⇒ – x² – y² + 32x + 32y + 4xy – 154 = 0

⇒ x² + 4xy + y² – 32x – 32y + 154 = 0

∴The equation of hyperbola is x² + 4xy + y² – 32x – 32y + 154 = 0.

问题 3. 求双曲线的偏心率、焦点坐标、准线方程和直角直角长度。

(i) 9x ² – 16y ² = 144

解决方案：

Given: 9x² – 16y² = 144

$⇒ \frac{9x^2}{144}-\frac{16y^2}{144} = 1$

$⇒ \frac{x^2}{16}-\frac{y^2}{9} = 1$

This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2} = 1$ where, a² = 16, b² = 9 i.e., a = 4 and b = 3

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{9}{16}}$

$= \sqrt{\frac{25}{16}}$

Eccentricity $= \frac{5}{4}$

Foci: The coordinates of the foci are (±ae, 0)

Foci = (±5, 0)

The equation of directrices is given as: $x = ±\frac{a}{e}$ ⇒ 5x ∓ 16 = 0

The length of latus-rectum is given as: 2b²/a = 2(9)/4

Length of latus rectum= 9/2

(ii) 16x ² – 9y ² = –144

解决方案：

Given: 16x² – 9y² = –144

$⇒ \frac{16x^2}{144}-\frac{9y^2}{144} = -1$

$⇒ \frac{x^2}{9}-\frac{y^2}{16} = -1$

This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2} = -1$ where, a² = 9, b² = 16 i.e., a = 3 and b = 4.

Eccentricity is given by:

$e = \sqrt{1+\frac{a^2}{b^2}}$

$= \sqrt{1+\frac{9}{16}}$

$= \sqrt{\frac{25}{16}}$

Eccentricity = $\frac{5}{4}$

Foci: The coordinates of the foci are (0, ±be)

(0, ±be) = (0, ±4(5/4))

= (0, ±5).

The equation of directrices is given as: x = $±\frac{b}{e}$ ⇒ 5x ∓ 16 = 0.

The length of latus-rectum is given as: 2a²/b = 2(9)/4 = 9/2.

(iii) 4x ² – 3y ² = 36

解决方案：

Given: 4x² – 3y² = 36

$⇒ \frac{4x^2}{36}-\frac{3y^2}{36} = 1$

$⇒ \frac{x^2}{9}-\frac{y^2}{12} = 1$

This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2} = 1$ where, a² = 9, b² = 12 i.e., a = 3 and b = √12

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{12}{9}}$

Eccentricity = $\frac{7}{3}.$

Foci: The coordinates of the foci are (±ae, 0)= (±ae, 0) = (±√21, 0)

The length of latus-rectum is given as= 2b²/a = 2(12)/3 = 24/3 = 8

(iv) 3x ² – y ² = 4

解决方案：

Given: 3x² – y² = 4

$⇒ \frac{3x^2}{4}-\frac{y^2}{4} = 1$

$⇒ \frac{x^2}{\frac{4}{3}}-\frac{y^2}{4} = 1$

$⇒ \frac{x^2}{(\frac{2}{\sqrt3})^2}-\frac{y^2}{(2)^2} = 1$

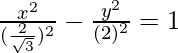
This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2} = 1$ where, $a = \frac{2}{\sqrt3}$ and b = 2

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{4}{3}}$

Eccentricity = 2

Foci: The coordinates of the foci are (±ae, 0)= (±ae, 0) = ±(2/√3)(2) = ±4/√3

(±ae, 0) = (±4/√3, 0)

The length of latus-rectum is given as:= 2b2/a = 2(4)/[2/√3] = 4√3.

(v) 2x ² – 3y ² = 5

解决方案：

Given: 2x² – 3y² = 5

$⇒ \frac{2x^2}{5}-\frac{3y^2}{5} = 1$

$⇒ \frac{x^2}{\frac{5}{2}}-\frac{y^2}{\frac{5}{3}} = 1$

$⇒ \frac{x^2}{(\sqrt{\frac{5}{2})}^2}-\frac{y^2}{(\sqrt{\frac{5}{3})}^2} = 1$

This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ where, $a = \sqrt{\frac{5}{2}}$ and $b = \sqrt{\frac{5}{3}}$

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{2}{3}}$

Eccentricity = $\sqrt{\frac{5}{3}}$.

Foci: The coordinates of the foci are (±ae, 0)

or, (±ae, 0) = $(±\frac{5}{\sqrt6}, 0)$

The length of latus-rectum is given as: 2b²/a = $\frac{10\sqrt2}{3\sqrt5}$

问题 4. 求双曲线 25x ² – 36y ² = 225的轴、偏心率、直角和焦点坐标。

解决方案：

Given: 25x² – 36y² = 225

$⇒ \frac{25x^2}{225}-\frac{36y^2}{225} = 1$

$⇒ \frac{x^2}{9}-\frac{4y^2}{25} = 1$

$⇒ \frac{x^2}{3^2}-\frac{y^2}{(\frac{5}{2})^2} = 1$

This is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ where, a = 3 and b = 5/2

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{25}{36}}$

$= \frac{\sqrt61}{6}$

Foci: The coordinates of the foci are (±ae, 0)

(±ae, 0) = (± √61/2, 0)

The length of latus-rectum is given as: 2b²/a

∴ Transverse axis = 6, conjugate axis = 5, e = √61/6, LR = 25/6, foci = (± √61/2, 0)

问题 5. 求双曲线的中心、偏心率、焦点和方向

(i) 16x ² – 9y ² + 32x + 36y – 164 = 0

解决方案：

Given: 16x² – 9y² + 32x + 36y – 164 = 0.

⇒ 16x² + 32x + 16 – 9y² + 36y – 36 – 16 + 36 – 164 = 0

⇒ 16(x² + 2x + 1) – 9(y² – 4y + 4) – 16 + 36 – 164 = 0

⇒ 16(x² + 2x + 1) – 9(y² – 4y + 4) – 144 = 0

⇒ 16(x + 1)² – 9(y – 2)² = 144

Here, center of the hyperbola is (-1, 2).

So, let x + 1 = X and y – 2 = Y

The obtained equation is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ where, a = 3 and b = 4.

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{16}{9}}$

$= \frac{5}{3}$

Foci: The coordinates of the foci are (±ae, 0)

X = ±5 and Y = 0

x + 1 = ±5 and y – 2 = 0

x = ±5 – 1 and y = 2

x = 4, -6 and y = 2

So, Foci: (4, 2) (-6, 2)

∴ The center is (-1, 2), eccentricity (e) = 5/3, Foci = (4, 2) (-6, 2), Equation of directrix = 5x – 4 = 0 and 5x + 14 = 0.

(ii) x ² – y ² + 4x = 0

解决方案：

Given:  x² – y² + 4x = 0.

⇒ x² – y² + 4x = 0

⇒ x² + 4x + 4 – y² – 4 = 0

⇒ (x + 2)² – y² = 4

Here, center of the hyperbola is (2, 0).

The obtained equation is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ where, a = 2 and b = 2

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{4}{4}}$

$= \sqrt{2}$

Foci: The coordinates of the foci are (±ae, 0)

X = ± 2√2 and Y = 0

X + 2 = ± 2√2 and Y = 0

X= ± 2√2 – 2 and Y = 0

So, Foci = (± 2√2 – 2, 0)

∴ The center is (-2, 0), eccentricity (e) = √2, Foci = (-2± 2√2, 0), Equation of directrix = x + 2 = ±√2.

(iii) x ² – 3y ² – 2x = 8

解决方案：

Given: x² – 3y² – 2x = 8.

⇒ x² – 3y² – 2x = 8

⇒ x² – 2x + 1 – 3y² – 1 = 8

⇒ (x – 1)² – 3y² = 9

Here, center of the hyperbola is (1, 0)

The obtained equation is of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ where, a = 3 and b = √3

Eccentricity is given by:

$e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{1+\frac{3}{9}}$

$= \frac{2\sqrt{3}}{3}$

Foci: The coordinates of the foci are (±ae, 0)

X = ± 2√3 and Y = 0

X – 1 = ± 2√3 and Y = 0

X= ± 2√3 + 1 and Y = 0

So, Foci = (1 ± 2√3, 0)

∴ The center is (1, 0), eccentricity (e) = 2√3/3, Foci = (1 ± 2√3, 0), Equation of directrix = X = 1±9/2√3.

问题 6. 在下列情况下，求双曲线方程，将其主轴称为坐标轴：

(i) 焦点之间的距离 = 16 和偏心率 = √2

解决方案：

Given: Distance between the foci = 16 and Eccentricity = √2

Let us compare with the equation of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$          …..(1)

Distance between the foci is 2ae and b² = a²(e² – 1)

So, 2ae = 16

⇒ ae = 16/2

⇒ a√2 = 8

⇒ a = 8/√2

⇒ a² = 64/2 = 32

We know that, b² = a²(e² – 1)

So, b² = 32[(√2)2 – 1]

= 32(2 – 1)

= 32

The Equation of hyperbola is given as $\frac{x^2}{32}-\frac{y^2}{32}=1$ 

⇒ x² – y² = 32

∴ The Equation of hyperbola is x² – y² = 32.

(ii) 共轭轴为 5，焦点之间的距离 = 13

解决方案：

Given: Conjugate axis = 5 and Distance between foci = 13

Let us compare with the equation of the form $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$             …..(1)

Distance between the foci is 2ae and b² = a²(e² – 1)

Length of conjugate axis is 2b

So, 2b = 5

⇒ b = 5/2

⇒ b² = 25/4

We know that, 2ae = 13

ae = 13/2

⇒ a²e² = 169/4

b² = a²(e² – 1)

⇒ b² = a²e² – a²

⇒ 25/4 = 169/4 – a²

⇒ a² = 169/4 – 25/4

⇒ a² = 144/4 = 36

The Equation of hyperbola is given as $\frac{x^2}{36}-\frac{y^2}{\frac{25}{4}}=1$

$⇒ \frac{x^2}{36}-\frac{4y^2}{25}=1$

∴ The Equation of hyperbola is 25x² – 144y² = 900.

(iii) 共轭轴为 7 并通过点 (3, -2)

解决方案：

Given: Conjugate axis = 7 and Passes through the point (3, -2)

Conjugate axis is 2b

So, 2b = 7

⇒ b = 7/2

⇒ b² = 49/4

The Equation of hyperbola is given as $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$

Since it passes through points (3, -2), we have

$\frac{3^2}{a^2}-\frac{(-2)^2}{b^2}=1$

$⇒ \frac{9}{a^2}-\frac{4}{\frac{49}{4}}=1$

⇒ a² = 441/65

The equation of hyperbola is given as: $\frac{x^2}{\frac{441}{65}}-\frac{y^2}{\frac{49}{4}}=1$

∴ The Equation of hyperbola is 65x² – 36y² = 441.

问题 7. 求双曲线方程：

(i) 焦点是 (6,4) 和 (-4,4)，偏心率是 2。

解决方案：

Clearly, coordinates of the center are (1,4).

Equation of the hyperbola is: 

$\frac{(x-1)^2}{a^2}-\frac{(y-4)^2}{b^2} = 1$

Distance between the foci = 2ae

⇒ 2ae = 10

⇒ a = 5/2

⇒ a² = 25/4

Since, b² = a²(e² – 1)

⇒ b² = 75/4

Putting the values in the equation, we get

$\frac{(x-1)^2}{\frac{25}{4}}-\frac{(y-4)^2}{\frac{75}{4}} = 1$

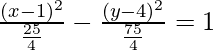
⇒ 12×2 – 4y2 – 24x + 32y -127 = 0.

(ii) 顶点是 (-8,-1) 和 (16,-1)，焦点是 (17,-1)

解决方案：

Clearly, coordinates of the center are (4,-1).

Equation of the hyperbola is : 

$\frac{(x-4)^2}{a^2}-\frac{(y+1)^2}{b^2} = 1$

Distance between vertices = 2ae

⇒ 24 = 2a

⇒ a = 12

⇒ a² = 144 and e² = 169/144

Since, b² = a²(e² – 1)

⇒ b² = 25

Putting the values in the equation, we get

$\frac{(x-1)^2}{144}-\frac{(y-4)^2}{25} = 1$

⇒ 25x² – 144y² – 200x – 288y – 3344 = 0.

(iii) 焦点是 (4,2) 和 (8,2)，偏心率是 2。

解决方案：

Clearly, coordinates of the center are (6,2).

Equation of the hyperbola is :

$\frac{(x-6)^2}{a^2}-\frac{(y-2)^2}{b^2} = 1$

Distance between the foci = 2ae

⇒ 2ae = 4

⇒ a = 1

Since, b² = a²(e² – 1)

⇒ b² =3

Putting the values in the equation, we get

$\frac{(x-6)^2}{1}-\frac{(y-2)^2}{3} = 1$

⇒ 3×2 – y2 – 36x + 4y + 101 = 0.

(iv) 顶点为 (0, ±7)，焦点位于 (0, ±28/3)。

解决方案：

Vertices of coordinates are (0, ±b) and (0, ±be).

⇒ b = 7

⇒ b² = 49

and, be = 28/3

⇒ e = 4/3 ⇒ e² = 16/9

Now, a² = b²(e²-1)

⇒ a² = 343/9

The equation becomes:

$\frac{x^2}{\frac{343}{9}}-\frac{y^2}{49} = 1$

问题 8. 如果共轭轴的长度是横移轴长度的 3/4，求偏心率。

解决方案：

Given: 2b = 6a/4

⇒ b/a = 3/4

⇒ b²/a² = 9/16

Now, $e = \sqrt{1+\frac{b^2}{a^2}}$

$= \sqrt{\frac{25}{16}}$

e = 5/4.

问题 9. 找出焦点在 (5,2) 和 (4,2) 并以 (3,2) 为中心的双曲线方程。

解决方案：

Clearly the coordinates of the first vertex are (2,2).

Equation of the hyperbola is :

$\frac{(x-3)^2}{a^2}-\frac{(y-2)^2}{b^2} = 1$

Distance between 2 vertices = 2a

 ⇒ a = 1

and, e = 2

 b² = a²(e² – 1)

⇒ b² = 3

The equation becomes:

$\frac{(x-3)^2}{1}-\frac{(y-2)^2}{3} = 1$

⇒ 3(x-3)² – (y-2)² = 3.

问题 10. 如果 P 是双曲线上任意一个轴相等的点，证明 SP.S'P = CP ² 。

解决方案：

Given: a = b

Equation becomes: x² – y² = a²

$e = \sqrt{2}$, C = (0,0), $S = (\sqrt{2}a,0)$ and $S' = (-\sqrt{2}a,0)$

SP. S’P = 4a⁴ + 4a²(a² + b²) + (a² + b²)² – 8a²b²

= (a² + b²)² = CP

Hence, SP.S’P = CP².

问题 11.求双曲线方程：

(i) 焦点是 (±2,0)，焦点是 (±3,0)。

解决方案：

Equation of the hyperbola is :

$\frac{x^2}{a^2}-\frac{y^2}{b^2} = 1$

Distance between the foci = 2ae

⇒ a = 2

⇒ a² = 4

e = 3/2

Since, b² = a²(e² – 1)

⇒ b² = 5

Putting the values in the equation, we get

$\frac{x^2}{4}-\frac{y^2}{5} = 1.$

(ii) 顶点为 (0, ±4)，焦点位于 (0, ±2/3)。

解决方案：

Vertices of coordinates are (0, ±b) and (0, ±be).

⇒ b = 4

⇒ b² = 16

and, be = 2/3

⇒ e = 2/3 ⇒ e² = 4/9

Now, a² = b²(e²-1)

⇒ a² = 343/9

The equation becomes:

$\frac{x^2}{\frac{343}{9}}-\frac{y^2}{49} = 1$

问题 12. 求焦点距离为 16，偏心率为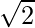$\sqrt{2}$ .

解决方案：

Distance between foci = 2ae = 16

$⇒ 2a(\sqrt{2}) = 16$

$⇒ a = \frac{16}{2\sqrt{2}}$

$⇒ a = 4\sqrt{2}$

$e = \frac{\sqrt{a^2+b^2}}{a}$

or, b² = 32

Equation becomes: x² – y² = 32.

问题 13.证明所有点的集合使得它们与 (4,0) 和 (-4,0) 的距离之差总是等于 2 表示双曲线。

解决方案：

Let P(x, y) be the point of the set.

Distance of P from (4,0) = $\sqrt{(x-4)^2+y^2}$

Distance of P from (-4,0) = $\sqrt{(x+4)^2+y^2}$

Given: 

$\sqrt{(x-4)^2+y^2} - \sqrt{(x+4)^2+y^2} = 2$

$⇒ \sqrt{(x-4)^2+y^2} = \sqrt{(x+4)^2+y^2} + 2$

Squaring both sides, we have

$-4x-1 = \sqrt{(x+4)^2+y^2}$

⇒ 15x² – y² = 15.

Thus, P represents a hyperbola.