反函数

我们知道这些功能就像机器或盒子一样，它们接受输入值并在处理后为我们提供一些输出值。只能有一个输出对应一个输入。有时可能会发生我们拥有输出，而我们想知道输入的情况。在这种情况下，我们需要还原对输入应用的所有处理。因此，了解该过程的逆向变得至关重要。如果该函数是数学函数，那么我们需要知道函数的逆函数是什么，以便我们可以取回输入。让我们详细了解逆过程。

逆函数简介

下图描述了一个函数及其反函数。该函数表示为f(x)，并接受一些输入值并给出输出。此函数的逆为f ^-1(x)的。例如，假设f(x)= 2x。它将输入的数字加倍，其反数应使它们减半以取回输入。 f ^-1 (x)= $\frac{x}{2}$ 。

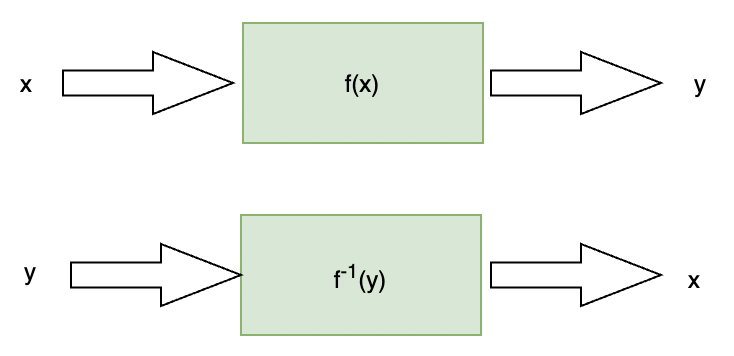
If functions f(x) and g(x) are inverses of each other, then f(x) = y only if g(y) = x. 

g(f(x)) = x

假设我们有一个函数f(x)= x ² 。现在我们被要求找出这个函数的逆函数。该函数对输入进行平方，我们知道我们需要取平方根来计算逆。

F ^-1(X)=√x2

=±x

我们看到可能有两个答案，选择哪个?在这种情况下，逆运算是不可能的。因此，对于可能产生逆函数的函数，我们需要注意一些事项。

• Function must be Bijective(One-One and Onto) for its inverse to exist.

This function has same values at two different values of input. This function is not ideal for calculating inverse. 

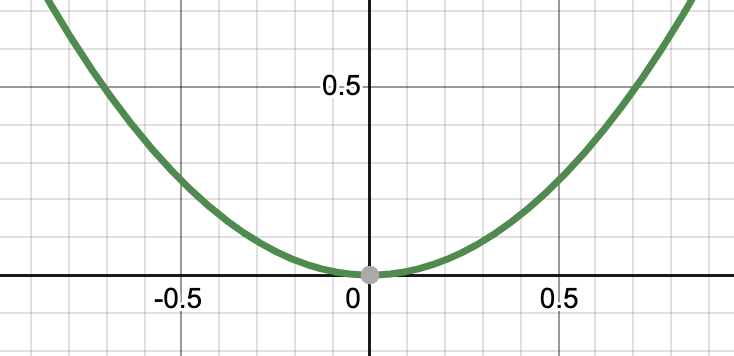
• We need to look for the points which are not in domain for the inverse. For example, in the previous case, negative values cannot be allowed.

用代数方法求逆

函数的逆还可以使用代数方法来计算。这个想法是，用y的值代替f(x)，然后求解y。让我们看一个例子来理解它。

示例：求f(x)= 6x + 10的反函数。

解决方案：

We know, f(x) = 6x + 10. Let’s substitute y in place of f(x). 

y = 6x + 10 

⇒ y – 10 = 6x 

⇒ x = $\frac{y - 10}{6}$

 f^-1(y) = $\frac{y - 10}{6}$

常用函数的逆函数

下表给出了一些常用函数的反函数，这些函数在计算复杂函数的反函数时可能会派上用场。该表表示函数，其逆函数和其极端情况。极端情况描述了不允许作为函数反函数输入的值。

Function	Inverse	Corner Cases
Multiplication	Divide	Divide by zero is not allowed
Divide	Multiply
Addition	Subtraction
Subtractions	Addition
xn	$x^{\frac{1}{n}}$	Negative values not allowed when n is even
ax	logax	x > 0 and a > 0
sin(x)	sin-1(x)	Only values between -1 to 1 are allowed
cos(x)	cos-1(x)	Only values between -1 to 1 are allowed
tan(x)	tan-1(x)

逆函数图

通过一个例子，我们将看到一个函数逆的图形的形状。假设我们有f(x)= e ^x 。假设此函数的逆是g(x)，我们知道指数函数的逆是对数函数。因此，g(x)= log _e x。下图显示了两个功能的图表。

注意在图中，您可以看到两条曲线相对于线y = x都是彼此的镜像。因此，可以说，当通过线y = x观察时，函数的逆函数是该函数的镜像。

让我们看看这些概念上的一些问题。

样本问题

问题1：求函数f(x)的反函数= $\frac{x + 4}{2x + 1}$

解决方案：

 $f(x) = \frac{x + 4}{2x + 1}$

Substituting f(x) with y. 

$y = \frac{x + 4}{2x + 1}$

⇒ $y(2x + 1) = x + 4$

⇒ 2xy + y = x + 4 

⇒ x(2y – 1) = 4 – y

⇒ x = $\frac{4 - y}{2y - 1}$

Thus, f^-1(y) = $\frac{4 - y}{2y - 1}$

问题2：求函数f(x)= lnx + 5的反函数。

解决方案：

f(x) = lnx + 5 

Substituting the f(x) with y 

y = lnx + 5 

⇒ lnx= y – 5

⇒ x = e^{(y – 5)}

 f^-1(y) = e^{(y – 5)}

问题3：找到以下函数的反函数并绘制其图形。

f(x)= e ^x + 20

解决方案：

f(x) = e^x + 20

Substituting the f(x) with y 

⇒y = e^x + 20

⇒y – 20 = e^x

⇒ln(y – 20) = x

f^-1(y) = ln(y – 20)

The figure below, shows the graphs for f(x) and it’s inverse. 

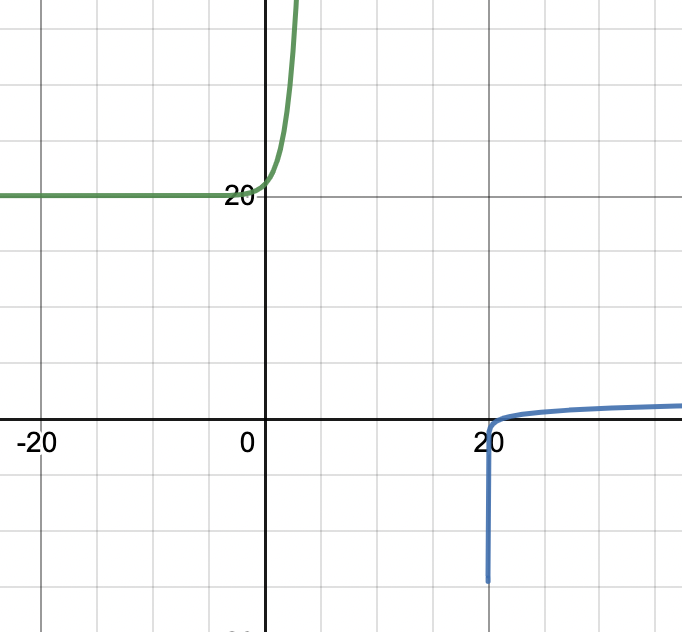
Notice that y > 20 for this function. 

问题4：陈述陈述是对还是错。对于给定的函数f(x)= x ² + 4，并非对x的所有值都存在逆。

解决方案：

We know that f(x) = x² + 4 is not bijective. For example, 

f(-2) = 8 and f(2) = 8. So, the inverse for this function cannot exist for all values of x. Thus, this statement is called False. 

问题5：找到以下函数的逆：

f(x)= $\frac{x}{5x + 1}$

解决方案：

f(x) = $\frac{x}{5x + 1}$ 

Substituting f(x) with y. 

$y = \frac{x}{5x + 1}$

⇒ $y = \frac{x}{5x + 1}$

⇒ y(5x + 1) = x 

⇒ 5xy + y = x

⇒ x(5y – 1) = -y 

⇒ x =$\frac{-y}{5y - 1}$

Thus, f^-1(y) = $\frac{-y}{5y - 1}$