反函数的导数

在数学中，如果给定g的输出返回给定f的输入值，则将一个函数(例如f)称为另一个函数(例如g)的逆函数。此外，这对于g的域co-domain(范围)中的每个元素都必须成立。例如，假设x和y是常数，如果g(x)= y和f(y)= x，则函数f被称为函数g的逆。换句话说，如果函数f：A⇢B是一个一，并且在函数或双射函数，则由g：B⇢A定义的函数称为函数f的逆。逆函数也称为反函数。函数的倒数由f ^-1表示。

f(g(x)) = g(f(x)) = x

在此，f和g是反函数。

查找f的逆的过程：

• 检查一对一函数。
• 如果可逆，则在f(x)的定义中互换x和y。
• 根据x查找y。
• 获得的y是从B⇢A定义的f的倒数。

Example: f(x) = e^x

y = e^x

Inverse of f(x) will be obtained by y ↔ x

x = e^y

y = ln x

Now, e^x and ln x are inverse function of each other.

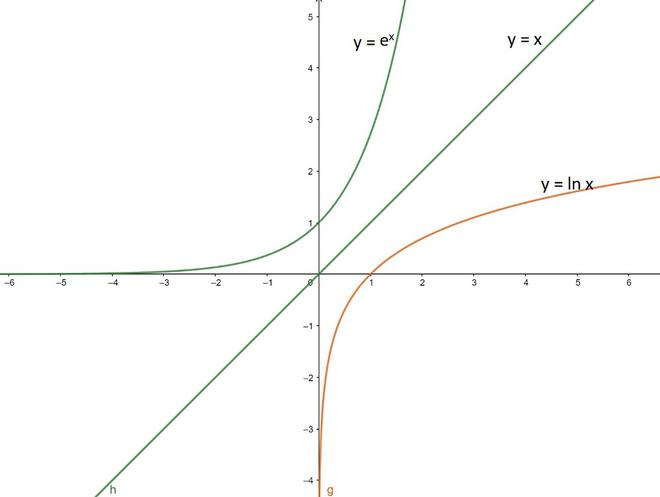
Inverse functions e^x and ln x are symmetry about y = x.

反函数的导数

我们已经知道逆函数是什么，所以现在我们要找到逆函数的导数。因此，如果函数f(x)是在间隔上定义的连续一对一函数或双射函数，以免说I，那么它的逆函数也是连续的，并且如果函数f(x)是可微函数，则其逆也是一个微分函数。

g'(x) = $\mathbf{\frac{1}{f'(g(x))}}$

在这里，f和g是反函数。它被称为逆函数定理。

Proof:

Let us considered f and g be the inverse functions and x is present in the g domain, then 

f(g(x)) = x

Differentiate both side with respect to x 

$\frac{d f(g(x))}{x} = \frac{d(x)}{dx}$

Now solve the LHS using chain rule we get

f'(g(x))g'(x) = 1

Now solve for g'(x):

g'(x) = ${\frac{1}{f'(g(x))}}$

因此，求解了反函数的导数。

例1：f(x)= e ^x ，检查条件是否成立。
解决方案：

As, f(x) = ex

y = e^x  

x = ln y

g(x) = f^-1(x) = ln x

Now, 

f'(x) = $\frac{d}{dx}$ (e^x) = e^x

g'(x) = $\frac{d}{dx}$ (ln x) = $\frac{1}{x}$

g'(f(x)) = $\frac{1}{e^x}$

$\frac{1}{g'(f(x))} = \frac{1}{\frac{1}{e^x}}$ = e^x

Hence, 

f'(x) = $\frac{1}{g'(f(x))}$, holds true.

示例2：令f(x)= $\frac{1}{2}$ x ³ + 3x – 4，令g为f的反函数，其中f(-2)= -14。求g’(-14)

解决方案：

f'(x) = $\frac{1}{2}$ (3x²) + 3

According to eq(1).

$g'(x) = \mathbf{\frac{1}{f'(g(x))}}$

As, f(x) = g^-1(x) and f(-2) = -14

then g(-14) = -2

x = -14

g'(-14) = $\frac{1}{f'(g(-14))}$

g'(-14) = $\frac{1}{f'(-2)}$

f'(-2) = $\frac{1}{2}$ (3(-2)²) + 3

f'(-2) = $\frac{12}{2}$+ 3

f'(-2) = 9

then, g'(-14) = $\frac{1}{9}$

如何从表中找到反函数的导数?

让我们借助示例来讨论这个概念。因此，让我们假设g和f是反函数，下表列出了f，g和f’的一些值。

x	f(x)	g(x)	f'(x)
2	4	8	$\frac{-1}{6}$
8	3	2	$\frac{1}{2}$

我们必须找到g’(2)。从问题中可以看出，f和g是反函数。这意味着如果我们有两个集合，现在让我们假设第一个集合是f的域。因此，在这个集合中，如果我们从某个x值开始，则f会将那个x映射到另一个值f(x)(这是函数f的用法)。现在我们知道g是f的倒数，因此g使我们回到第一个集合(这是对函数g的使用)。

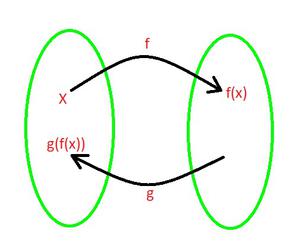
因此我们得到

g(f(x))= x…(i)

f(g(x))= x…(ii)

两者都有效。

从等式(ii)，我们有

f(g(x))= x

现在区分双方wrtx我们得到

$\frac{d(f(g(x)))}{dx} = \frac{d(x)}{dx}$

现在在LHS上我们应用链式规则，现在我们得到

f’(g(x))g’(x)= 1

g'(x) = $\mathbf{\frac{1}{f'(g(x))}}$

现在我们将找到g’(2)的值

g’(2)= $\frac{1}{f'(g(2))}$

从表中我们得到g(2)的值

g’(2)= $\frac{1}{f'(8)}$

从表中我们得到f’(8)的值

g’(2)= $\frac{1}{\frac{1}{2}}$

g’(2)= 2

因此，g’(2)的值= 2。

如何找到反三角函数的导数?

我们注意到反三角函数是连续函数。现在，我们使用第一原理和链式规则来找到这些函数的派生：

1.由f(x)= sin ^–1 x给出的f的导数。

From first principle 

f(x) = sin^–1 x and f(x+h) = sin^–1 (x+h)

$\frac{d}{dx}(f(x)) = \lim_{h\to0} \frac{f(x+h)-f(x)}{h} \\ = \lim_{h\to0} \frac{sin^{-1}(x+h)-sin^{-1}x}{h}\\ = \lim_{h\to0} \frac{sin^{-1}[(x+h)\sqrt{1-x^2} - x\sqrt{1-(x+h)^2}]}{h}$

Using the formula,

sin^–1 x – sin^–1 y = sin-1(x $\sqrt{1-y^2}$ – y $\sqrt{1-x^2}$)

$= \lim_{h\to0} \frac{sin^{-1}[(x+h)\sqrt{1-x^2}-x\sqrt{1-(x+h)^2}]}{(x+h)\sqrt{1-x^2}-x\sqrt{1-(x+h)^2}} \times \frac{(x+h)\sqrt{1-x^2}-x\sqrt{1-(x+h)^2}}{h}$

As

$\lim_{x\to0} (\frac{sin^{-1}x}{x}) = 1 \\ So, \\ = \lim_{h\to0} 1. \frac{(x+h)\sqrt{1-x^2}-x\sqrt{1-(x+h)^2}}{h} \\ = \lim_{h\to0} \frac{(x+h)^2(1-x^2)-x^2(1-(x+h)^2)}{h} \times \frac{1}{(x+h)\sqrt{1-x^2}+x\sqrt{1-(x+h)^2}}\\ = \lim_{h\to0} \frac{(x+h)^2-x^2}{h} \times \frac{1}{(x+h)\sqrt{1-x^2}+x\sqrt{1-(x+h)^2}}\\ = \lim_{h\to0} (2x+h) \times \frac{1}{(x+h)\sqrt{1-x^2}+x\sqrt{1-(x+h)^2}}\\ = \frac{(2x)}{2x \sqrt{1-x^2}}\\ = \frac{1}{\sqrt{1-x^2}}$

Hence

$\frac{d}{dx} (sin^{-1}x) = \frac{1}{\sqrt{1-x^2}}$

From chain rule 

y = sin^-1x

sin y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(sin \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(sin \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ \cos\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = \frac{1}{cos\hspace{0.1cm}y}\\ \frac{dy}{dx} = \frac{1}{\sqrt{1-sin^2\hspace{0.1cm}y}}$

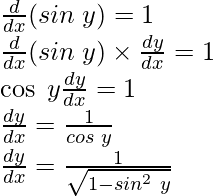
As

$\sin\hspace{0.1cm}y = x \\ \frac{dy}{dx} = \frac{1}{\sqrt{1-x^2}}\\$

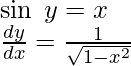
2.由f(x)= cos ^–1 x给出的f的导数。

From first principle

f(x) = cos^–1 x and f(x+h) = cos^–1 (x+h)

$\frac{d}{dx}(f(x)) = \lim_{h\to0} \frac{f(x+h)-f(x)}{h} \\ = \lim_{h\to0} \frac{cos^{-1}(x+h)-cos^{-1}x}{h}\\ = \lim_{h\to0} \frac{(\frac{\pi}{2}-sin^{-1}(x+h))-(\frac{\pi}{2}-sin^{-1}x)}{h}$

As

$cos^{-1} x = \frac{\pi}{2} - sin^{-1} x \\ = - \lim_{h\to0} \frac{sin^{-1}(x+h)-sin^{-1}x)}{h}$

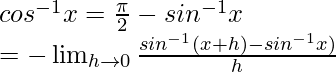
By using the previous result

$= - \frac{1}{\sqrt{1-x^2}}$

Hence, 

$\frac{d}{dx} (cos^{-1}x) = -\frac{1}{\sqrt{1-x^2}}$

From chain rule

y = cos^-1x

cos y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(cos \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(cos \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ -sin\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = - \frac{1}{sin\hspace{0.1cm}y}\\ \frac{dy}{dx} = -\frac{1}{\sqrt{1-cos^2\hspace{0.1cm}y}}$

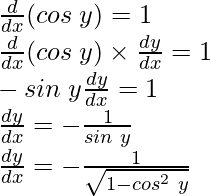
As

$cos\hspace{0.1cm}y = x \\ \frac{dy}{dx} = -\frac{1}{\sqrt{1-x^2}}\\$

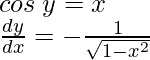
3. f的导数由f(x)= tan ^–1 x给出。

From first principle

f(x) = tan^–1 x and f(x+h) = tan^–1 (x+h)

$\frac{d}{dx}(f(x)) = \lim_{h\to0} \frac{f(x+h)-f(x)}{h} \\ = \lim_{h\to0} \frac{tan^{-1}(x+h)-tan^{-1}x}{h}$

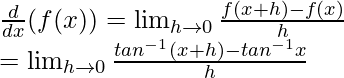
As

$tan^{-1} x - tan^{-1}y = tan^{-1}(\frac{x-y}{1+xy}) \\ = \lim_{h\to0} \frac{tan^{-1}(\frac{x+h-x}{1+x(x+h)})}{h}\\ = \lim_{h\to0} \frac{tan^{-1}(\frac{h}{1+x^2+xh})}{\frac{h}{1+x^2+xh}} \times \frac{1}{1+x^2+xh}\\ = 1. \frac{1}{1+x^2}$

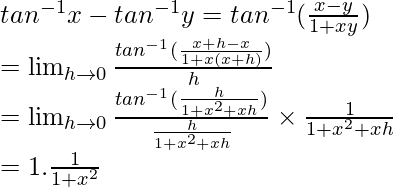
Hence

$\frac{d}{dx} (tan^{-1}x)= \frac{1}{1+x^2}$

From chain rule

y = tan^-1x

tan y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(tan \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(tan \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ sec^2\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = \frac{1}{sec^2\hspace{0.1cm}y}\\ \frac{dy}{dx} = \frac{1}{1+tan^2\hspace{0.1cm}y}$

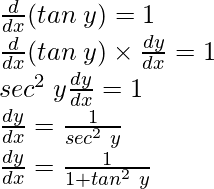
As

tan y = x dy/dx = 1/1+x²

4.由f(x)= cot ^–1 x给出的f的导数。

From first principle

f(x) = cot^–1 x and f(x+h) = cot^–1 (x+h)

$\frac{d}{dx}(f(x)) = \lim_{h\to0} \frac{f(x+h)-f(x)}{h} \\ = \lim_{h\to0} \frac{cot^{-1}(x+h)-cot^{-1}x}{h}$

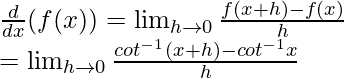
As

$cot^{-1} x = \frac{\pi}{2} - tan^{-1}x\\ = \lim_{h\to0} \frac{(\frac{\pi}{2} - tan^{-1}(x+h))-(\frac{\pi}{2} - tan^{-1}x)}{h}\\ = - \lim_{h\to0} \frac{tan^{-1}(x+h)-tan^{-1}x}{h}$

$= - \frac{1}{1+x^2}$

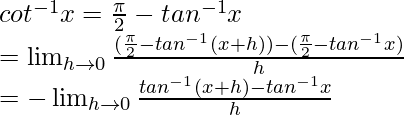
Hence

$\frac{d}{dx} (cot^{-1}x)= \frac{-1}{1+x^2}$

From chain rule

y = cot^-1x

cot y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(cot \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(cot \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ -cosec^2\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = \frac{-1}{cosec^2\hspace{0.1cm}y}\\ \frac{dy}{dx} = \frac{-1}{1+cot^2\hspace{0.1cm}y}$

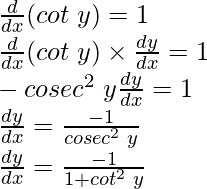
As

cot y = x dy/dx = -1/1 + x²

5.由f(x)= sec ^–1 x给出的f的导数。

From chain rule

y = sec^-1x

sec y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(sec \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(sec \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ sec\hspace{0.1cm}y \hspace{0.1cm}tan\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = \frac{1}{sec\hspace{0.1cm}y \hspace{0.1cm}tan\hspace{0.1cm}y}\\ \frac{dy}{dx} = \frac{1}{|sec\hspace{0.1cm}y| \hspace{0.1cm}|tan\hspace{0.1cm}y|}\\$

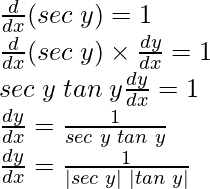
If x > 1, then y ∈ (0, π/2)

∴ sec y > 0, tan y > 0 ⇒ |sec y||tan y| = sec y tan y

If x < -1, then y ∈ (π/2,π)

∴ sec y < 0, tan y < 0 ⇒ |sec y||tan y| = (-sec y) (-tan y) = sec y tan y

$\frac{dy}{dx} = \frac{1}{|sec \hspace{0.1cm}y| \sqrt{tan^2y}}\\ \frac{dy}{dx} = \frac{1}{|sec \hspace{0.1cm}y| \sqrt{1-sec^2y}}$

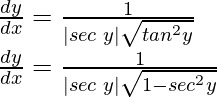
As

sec y = x 

$\frac{dy}{dx} = \frac{1}{|x|\sqrt{x^2-1}}\\$

6.由f(x)= cosec ^–1 x给出的f的导数。

From chain rule

y = cosec^-1x

cosec y = x

Differentiating both sides w.r.t x, we get

$\frac{d}{dx}(cosec \hspace{0.1cm}y) = 1 \\ \frac{d}{dx}(cosec \hspace{0.1cm}y) \times \frac{dy}{dx} = 1\\ -cosec\hspace{0.1cm}y \hspace{0.1cm}cot\hspace{0.1cm}y\frac{dy}{dx} = 1\\ \frac{dy}{dx} = \frac{1}{-cosec\hspace{0.1cm}y \hspace{0.1cm}cot\hspace{0.1cm}y}\\ \frac{dy}{dx} = \frac{-1}{|cosec\hspace{0.1cm}y| \hspace{0.1cm}|cot\hspace{0.1cm}y|}\\$

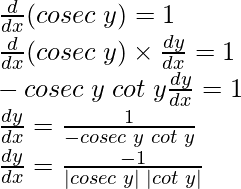
If x > 1, then y ∈ (0, π/2)

∴ cosec y > 0, cot y > 0 ⇒ |cosec y||cot y| = cosec y cot y

If x < -1, then y∈ (-π/2, 0)

∴ cosec y < 0, cot y < 0 ⇒ |cosec y||cot y| = (-cosec y) (-cot y)

$\frac{dy}{dx} = \frac{-1}{|cosec \hspace{0.1cm}y| \sqrt{cot^2y}}\\ \frac{dy}{dx} = \frac{-1}{|cosec \hspace{0.1cm}y| \sqrt{cosec^2y-1}}$

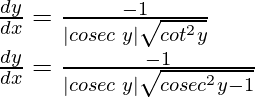
As

cosec y = x

$\frac{dy}{dx} = \frac{-1}{|x|\sqrt{x^2-1}}\\$

例子：

问题1.找到y = tan ^-1 (x ² )的导数。

解决方案：

Differentiating both side, we get

$\frac{dy}{dx} = \frac{d(tan^{-1} (x^2))}{dx}$ 

Using the inverse derivative of tan^-1 θ

= $\frac{1}{1+(x^2)^2} \frac{d (x^2)}{dx}$ 

 = $\frac{2x}{1+x^4}$

问题2。求y = sin ^-1 (3x-2)的导数。

解决方案：

Differentiating both side, we get

$\frac{dy}{dx} = \frac{d (sin^{ -1} (3x-2))}{dx}$

Using the inverse derivative of sin^-1 θ

= $\frac{1}{\sqrt{1-(3x-2)^2}} \frac{d(3x-2)}{dx}$ 

= $\frac{1}{\sqrt{1-(9x^2-18x+4)}}$(3)

= $\frac{3}{\sqrt{(18x-9x^2-4)}}$

问题3。求y = cos ^-1 (1 – x ² )的导数。

解决方案：

Differentiating both side, we get

$\frac{dy}{dx} = \frac{d(cos^{ -1} (1 - x^2))}{dx}$ 

Using the inverse derivative of cos^-1 θ

= $\frac{-1}{\sqrt{1-(1-x^2)^2}} \frac{d(1-x^2)}{dx}$ 

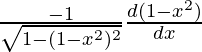
= $\frac{-1}{\sqrt{1-(1-2x^2+x^4)}}$(-2x)

= $\frac{2x}{\sqrt{(2x^2-x^4)}}$

= $\frac{2}{\sqrt{(2-x^2)}}$