四边形的类型–一些特殊的平行四边形

四边形可以被定义为，类型的具有四个侧面，四个顶点，并有一对对角线沿着四个角度多边形。内部的萨姆角四边形的是360°。有各种各样的四边形。顾名思义，该词是两个拉丁词的组合，“ Quadri ”是四个变体，“ latus ”是“ side”。在本主题中，我们将研究一些特殊类型的平行四边形，例如矩形，正方形，菱形。

平行四边形是一种特殊的四边形，其相对边相等且平行。

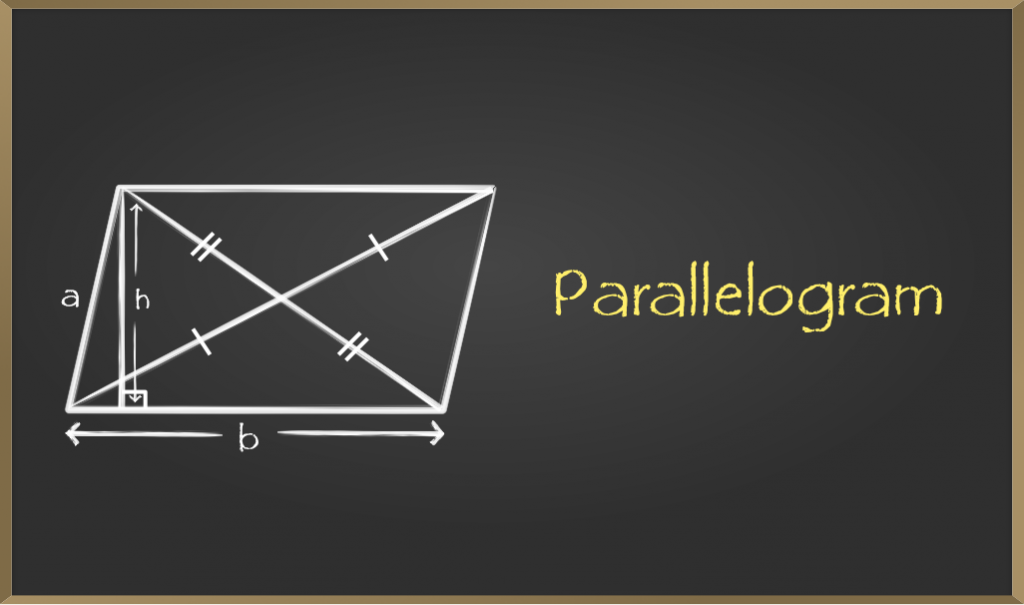
平行四边形的性质

平行四边形具有不同的属性。

1. 相对的边是相等的。
2. 对角相等
3. 对角线一分为二。
4. 平行四边形的对角线将其分成两个相等的三角形。

一些特殊的平行四边形

根据属性，有三种特殊类型的平行四边形：

• 长方形
• 菱形
• 正方形

长方形

矩形是平行四边形的一种特殊类型，它具有平行四边形的所有属性以及一些不同的属性。矩形的每个角度必须为直角，即90°。

矩形的属性

矩形的属性类似于平行四边形：

• 对面彼此平行。
• 矩形的相对边相等。
• 对角线一分为二
• 矩形的对角线相等。
• 矩形的每个内角相等，即90°

Note: Rectangle has all the properties of parallelogram. So all rectangles are parallelogram but all parallelograms are not rectangle.

矩形上的样本问题

问题1：在矩形ABCD中，AO = 5厘米。找出对角线BD的长度。如果AB = 8cm，AD = 6cm，则还要找到矩形的周长。

解决方案：

AO = OC = 5cm (diagonals bisect each other)

Therefore, AC = 10cm

BD = AC =10cm (diagonals of rectangle are equal) 

Perimeter = AB + BC + CD + DA

                 =8 + 6 +8 +6 (opposite sides are equal)

                 = 28cm

问题2：在矩形ABCD中，∠ABD= 3x – 7和∠CBA= 6x –2。找到x的值。

解决方案：

Each angle of rectangle is 90⁰

Therefore,

 ∠ABD + ∠CBA = 90⁰

3x – 7 + 6x – 2 = 90

9x – 9 = 90

9x = 99

x = 11

问题3：在ABCD矩形中AO = 2x – 10 cm，OB = x + 4 cm。找出对角线BD的长度

解决方案：

In rectangle diagonals bisect each other and are equal.

Therefore, AO = OB

2x – 10 = x + 4

x = 14

OB = 14 + 4 = 18 cm

OD = 18 cm (as diagonals bisect each other)

Therefore, BD = 36 cm

菱形

四边相等且相对边平行的四边形称为菱形。菱形的对角相等，菱形的对角线彼此垂直平分。请注意，所有菱形都是平行四边形，但事实并非如此。

菱形的属性

菱形的属性类似于平行四边形：

• 对面彼此平行。
• 菱形的所有边彼此相等。
• 对角线一分为二
• 菱形的对角相等。

Note: Rhombus is a parallelogram with all side equal

菱形上的样本问题

问题1：菱形的对角线分别为24cm和10cm。找到菱形的一面。

解决方案：

AC = 24cm

BD = 10cm

Therefore, AO = 12cm and OB = 5cm (diagonals bisect each other)

In right-angled triangle AOB, (diagonals of rhombus are perpendicular)

AB² = OA² + OB²

AB² = 12² + 5²

AB² = 144 + 25

AB² = 169

AB = 13cm

Therefore, side of rhombus is 13cm.

问题2：在菱形中，对角线之一等于菱形的一侧。找到菱形的角度。

解决方案：

In rhombus PQRS PR = PQ (given)

Therefore, PQ = QR = RS = SP = PR (as all side of rhombus are equal)

In triangle PQR

PQ = QR = PR 

Therefore, it is an equilateral triangle.

∠QPR = ∠Q = ∠QRP = 60⁰

||ly ∠SPR = ∠S = ∠PRS = 60⁰

Therefore, angles of rhombus are ∠P = 120⁰ , ∠Q = 60⁰, ∠R = 120⁰, ∠S = 60⁰

问题3：推导菱形的公式。

解决方案：

As diagonals of rhombus bisect each other at right angle.

In rhombus ABCD 

area of triangle ABD = 1/2 * BD *AO (1/2 * base *height) ……….  (1)

area of triangle BCD = 1/2 * BD * CO ………………………………………….. (2)

Area of rhombus = Area of triangle ABD + area of triangle BCD

                             = 1/2 * BD * AO + 1/2 * BD * CO

                              = 1/2 * BD (AO + CO)

                              = 1/2 * BD * AC           (AE + CE = AC)

Therefore, area of rhombus = 1/2 * product of diagonals

正方形

四边形，其所有侧面相等，并且相对侧面平行，并且所有内角均等于90°，称为四边形。正方形的对角线彼此垂直一分为二。请注意，所有正方形都是菱形，但反之亦然。

正方形的性质

正方形的属性类似于菱形的属性：

• 对面彼此平行。
• 正方形的所有边彼此相等。
• 对角线是彼此垂直的等分线并且相等。
• 正方形的所有角度均相等，且每个角度均为90°。

广场上的样本问题

问题1：所有菱形都是正方形或所有正方形都是菱形。以下哪项陈述是正确的，为什么?

解决方案：

Square and rhombus both have all sides equal but a rhombus is called square if each of its angle is 90⁰. So all squares can be called rhombus but converse is not true.

问题2：在图中ROPE是一个正方形。证明对角线相等。

解决方案

In Δ REP and Δ OEP

RE = OP (sides of square)

∠E = ∠P (each 90⁰)

EP = EP (common)

Therefore, triangles are congruent by SAS criteria.

Therefore, RP = OE (c.p.c.t)

Therefore, diagonals of square are equal.