三角形中的不等式

我们知道，由三个边组成的闭合图形称为三角形。三角形具有三个边，三个顶点和三个角度。任何类型的三角形的角度总和始终为180°。三角形的类型有三种：等边三角形(所有边和所有角度始终相等(60°))，等腰三角形(此处将两个边和两个角度视为相等)，斜角三角形(这些是最广义的形式)三角形，因为没有任何一个边或角度被认为是相等的)。如果三角形的高度表示为H，而底数表示为B，则

三角形的面积显示为$\frac{1}{2}\times B \times H$

在上图中，ABC是一个三角形。 AB，BC和AC是三角形的三个边，∠A，∠B和∠C是该三角形的角度。让我们研究三角形的一些性质和不等式。

等腰三角形

两个边相等的三角形称为等腰三角形。因此， ∆ABC的AB = AC，“等腰线”一词的意思是“两条腿”，因此，在三角形中，当两条腿/两侧相等时，该三角形也称为等腰三角形。在众所周知的等腰三角形中，一个是“右等腰三角形” ，其中一个角度为90°，并且两个边(底边和垂直边)相等。

等腰三角形的示例：

定理：等腰三角形中与等边相反的角度相等。

证明：

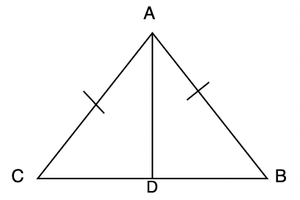
We need to prove that ∠B and ∠C are equal. Let’s draw bisector of ∠A and join it to BC. 

In triangle BAD and CAD, 

AB = AC, 

∠BAD =∠CAD

AD = AD

So, the two triangles are congruent. 

So, ∠B and ∠C are corresponding angles of congruent triangles. Thus, they are equal. 

这个定理的反面也成立吗?如果三角形的两个角度相等，是否意味着与它们相对的边也相等?

Yes, the converse is also true, this theorem can be proved in a similar manner, by using the ASA congruence rule. 

让我们解决有关这些属性的一些与证明相关的问题：

问题1： E和F分别是ΔABC的等边AB和AC的中点(见图)。证明BF = CE。

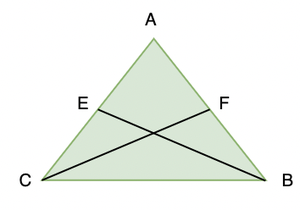
解决方案：

In Δ ABF and Δ ACE,

AB = AC (Given)

∠ A = ∠ A (Common)

AF = AE (Halves of equal sides)

So, Δ ABF ≅ Δ ACE (SAS rule)

Therefore, BF = CE (CPCT)

问题2：在等腰三角形中，AB = AC，D和E的ABC是BC上的点，因此BE = CD。证明AD = AE。

解决方案：

In Δ ABD and Δ ACE,

AB = AC (Given)

∠ B = ∠ C (Angles opposite to equal sides) 

Also, BE = CD

So, BE – DE = CD – DE

That is, BD = CE

So, Δ ABD ≅ Δ ACE(Using SAS Rule)

This gives AD = AE

Hence, Proved. 

等边三角形

三个边都相等的三角形称为等边三角形。等边三角形的所有三个角度都相等，并且由于所有角度的总和为180°，因此每个角度分别为60°。重要的是要注意，所有等边三角形始终彼此相似。

定理：如果ABC是等边三角形，而P是三角形ABC外接圆的弧BC上的点，则； PA = PB + PC

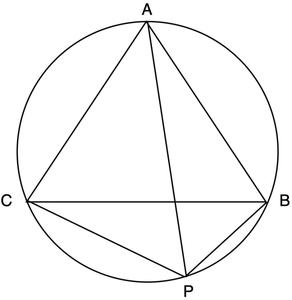
证明：

For a cyclic quadrilateral ABPC, we have;

PA.BC=PB.AC+PC.AB

For an equilateral triangle ABC,

AB = BC = AC

So, PA.AB = PB.AB+PC.AB

Taking AB as a common;

PA.AB = AB(PB+PC)

PA = PB + PC

Hence, proved.

订购三角形的边和角

如果给定角度，我们可以按长度增加的顺序订购边。类似地，三角形的角度可以排序。最大的一面与最大的角度正好相反的属性等我们来解决。

问题：在下面给出的ABC三角形中，使用给定的角度从最短到最长对边进行排序。

回答：

In any triangle, the smallest angle is always opposite to the shortest side and the largest angle is always opposite to the longest side and vice versa. In the triangle ABC given above, because angle A is the smallest angle, the side BC must be the shortest side. And also, because angle B is the largest angle, the side AC must be the longest side. Let’s put it all together. Hence, the order of the three sides from shortest to longest is side BC, side AB, and side AC

三角不等式定理

到目前为止，我们一直专注于一个或多个三角形的边和角的相等性。有时，我们确实遇到不平等的物体，我们需要对它们进行比较。

定理1：如果三角形的两个边不相等，则与较大边相反的角度较大。

定理2：在任何三角形中，与较大(较大)角度相反的一侧较长。这与上述定理1相反。

定理3：三角形的任意两个边的总和始终大于第三个边。

证明：

Notice that the side BA of Δ ABC has been produced to a point D such that AD = AC. Now, since ∠BCD > ∠BDC. 

By the properties mentioned above, we can conclude that BD > BC. 

We know that, BD = BA + AD

So, BA + AD > BC 

= BA + AC > BC 

So, this proved sum of two sides triangle is always greater than the other side.  

问题： D是三角形ABC的BC边上的点，因此AD = DC。显示AB> BD。

解决方案：

In triangle DAC, 

AD = AC, 

∠ADC = ∠ACD (Angles opposite to equal sides)

∠ ADC is an exterior angle for ΔABD.

∠ ADC > ∠ ABD

∠ ACD > ∠ ABD

∠ ACB > ∠ ABC

AB > AC (Side opposite to larger angle in Δ ABC)

AB > AD (AD = AC)