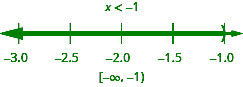线性不等式是包含线性函数的不等式。当两个不等式由单词“ and”连接时,当两个不等式在相等的时间为真时,就会出现答案。但是,当两个不等式由单词“或”连接时,当其中一个不等式为真时,就会出现解决方案。解决方案是两个单独解决方案的组合或结合。
复合不等式
复合不等式是通过“ AND ”或“ OR ”组合两个简单不等式的不等式。
带“ AND”的复合不等式表明,这两个陈述之一在本质上是正确的。如果对于复合不等式的各个陈述,解决方案存在重叠。而“或”表示,只要一个陈述中的任何一个为真,则整个复合句子为真。这称为单个语句的解决方案集的并集。
复合不等式:OR
不等式带有“ OR”的图表示并集,因为一个陈述中的任何一个为真,整个复合句子为真。一个或一个不等式的解决方案是,一个值必须使不等式的一部分成为真实。如果数字是至少一个不等式的解,则数字是对化合物不等式的解。当在复合不等式中使用时,连词“或”形成所谓的析取。
示例:x <3或x> 5
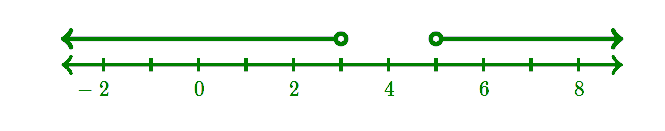
数字0和6都是不等式的解,但是数字4不是给定不等式的解
复合不等式:AND
具有“ and”的复合不等式的图表示不等式的图的交集。要回答AND不等式,必须使两个部分都成立。它是各个语句的答案集的重叠或交集。如果数量可能是两个不等式的解,则数字可能是化合物不等式的解。使用单词“和”的复合不等式被理解为合取。
示例:x> 0 AND x <4
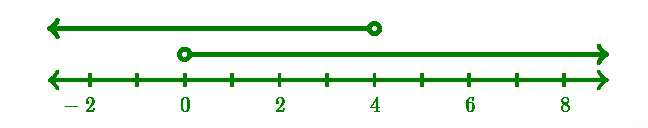
因此,在这种情况下,2是复合不等式的解,但是5并不是因为它仅满足一个不等式,而不能同时满足这两个不等式。
Note: It can also be written as,
0 < x < 4
复合不等式问题
问题1:解决x in 2x +3≥7或2x + 9> 11
解决方案:
Solving the first inequality for x, we get:
2x+3≥7
2x≥4
x≥2
Solving the second inequality for x, we get:
2x+9>11
2x>2
x>1
Graphically, we get:
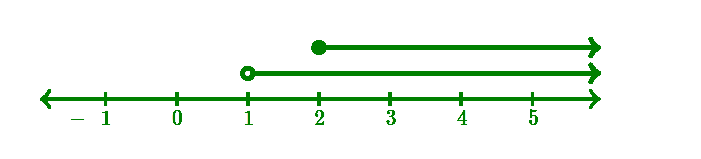
The inequality can be expressed as the simple inequality:
x > 1
问题2:在2y + 7 <13或−3y – 2 <10中求解y
解决方案:
2y + 7< 13 or -3y – 2 ≤ 10
+ 7 -7 + 2 + 2
2y/2 < 6/2 -3y/-3 ≥ 12/-3
y < 3 or y ≥ -4
The solution is all real numbers.
This number line shows the solution set of y < 3 or y ≥ 4.

问题3:解决5−3x≤−1或8 +2x≤5。绘制解决方案的图形,并以时间间隔表示法编写解决方案。
解决方案:
Given, 5 – 3x ≤ – 1 or 8 + 2x ≤ 5
Solve each inequality. 5 – 3x ≤ – 1 8 + 2x ≤ 5
−3x ≤ −6 2x ≤ −3
x ≥ 2 or x ≤ −32

Graph numbers that make either inequality true.
The answer for the given inequalities is (−∞,−32]∪[2,∞)
问题4:求解x:3 x + 2 <14和2 x – 5> -11。
解决方案:
为了解决这个问题,我们将从分别求解每个方程式开始。当它们由“和”连接时,则意味着所需的解决方案是重叠或交集。
3x + 2 < 14
Now, Subtract 2 form it and divide it by 3 on both sides
3x + 2 – 2 < 14 – 2
3x/3 < 12/3
x < 4 2x – 5 > -11
Now,
Add 5 to both sides of the equation and divide the equation by 2
2x – 6 + 5 > -11 + 5
2x > -6
x > -3
x < 4 indicates that all the numbers to the left are 4, and x >( -3 )indicates that all numbers to the right of the inequality are -3. Hence, the solution for this compound inequalities will be, x > –3 and x < 4
问题5:解决3(2x + 5)≤18和2(x – 7)<-6。绘制解决方案的图形,并以时间间隔表示法编写解决方案。
解决方案:
3(2x + 5) ≤18 and 2(x – 7)<−6
Solve each inequality. 6x+15≤18 2x – 14<-6
6x ≤ 3 2x < 8
x ≤ 12 and x < 4
The answer for the given inequalities is (−∞, 12]
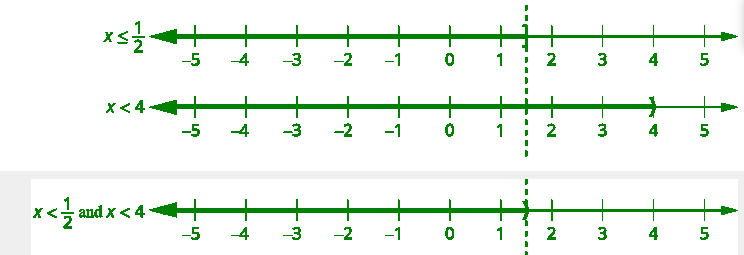
问题6:解决复合不等式。绘制解决方案的图形,并以时间间隔表示法写解决方案:5(3x – 1)≤10和4(x + 3)<8。
解决方案:
这是一个非常简单的问题,似乎可以直接解决