TCS 安置文件 |第八题

这是一份用于能力准备的 TCS 模型安置论文。这份安置文件将涵盖 TCS 招聘活动中提出的能力问题，并严格遵循 TCS 面试中提出的问题模式。建议解决以下每个问题，以增加通过 TCS 面试的机会。

确定系列中缺失的数字：2、5、__、19、37、75？
一）16
b) 12
c) 10
d) 9
```
Answer: d) 9
```
Solution:
2 * 2 + 1 = 5
5 * 2 – 1 = 9
9 * 2 + 1 = 19
19 * 2 – 1 = 37 and so on

编程需要懂一点英语
一个矩形被分成四个矩形，面积分别为 70、36、20 和 x。 “x”的值是多少？

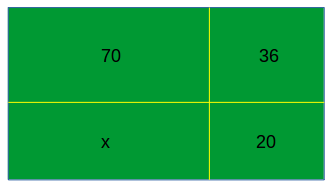
a) 350/7
b) 350/11
c) 350/9
d) 350/13
```
Answer: c) 350/9
```
Solution:
Since the areas of the rectangles are in proportion we can say,
=> 70/x = 36/20
=> x = 350/9

编程需要懂一点英语
如果 VXUPLVH 写成 SURMISE，那么 SHDVD 写成什么？
一）PEASA
b) PBASA
c) PEBSB
d) 以上都不是
```
Answer: a) PEASA
```
Solution:
It is a question of coding-decoding where,
V is written as S (V – 3 = S)
X is written as U (X – 3 = U)
and so on.
Similarly, SHDVD will be written as PEASA

编程需要懂一点英语
Aman 欠 Bipul 50 卢比。他同意从星期一开始连续几天向 Bipul 付款，每天支付一张 10 卢比或 20 卢比的票据。 Aman 可以通过多少种不同的方式来回报 Bipul。（两种方式据说不同，如果至少一天，给出不同面额的纸币）
一）5
b) 6
c) 7
d) 8
```
Answer: d) 8
```
Solution:
Aman can pay Bipul in all 10 rupees note in 5 days = 5 * 10 = 50 rupees = 1 way
Aman can pay Bipul in 3 ten rupee note and 1 twenty rupee note = 4!/(3! * 1!) = 4 ways
Aman can pay Bipul in 1 ten rupee note and 2 twenty rupee note = 3!/(1! * 2!) = 3 ways
So in all Aman can pay Bipul in 8 ways.

编程需要懂一点英语
Salim 以 27 个橙子的价格购买了一定数量的橙子，价格为 2 乘以 M 的卢比，其中 M 是一个整数。他将这些橙子分成相等的两半，其中一部分以 13 个橙子的价格出售，另一部分以 14 个橙子的价格出售。最少的橙子。他买了多少？
一）980
b) 9828
c) 1880
d) 102660
```
Answer: b) 9828
```
Solution:
Let Salim buy 2x number of oranges.
So he buys 27 oranges at a price of 2M.
He buys 1 orange at a price of 2M/27
or, x oranges cost him Rs. 2Mx/27
Now he sells x oranges at the rate of 13 oranges for Rs. M
So he sells 1 orange at Rs. M/13
and x oranges at Rs Mx/13
Same goes for 14 oranges which are Mx/14,
According to the question, 2Mx/27, Mx/13, Mx/14 are integers
So, x oranges must be divisible by 27, 13 and 14
The lcm of 27, 13 and 14 = 4914 or 2x = 9828

编程需要懂一点英语
在一场足球比赛中，有 16 支球队参加，分为 4 个小组。每个小组的每个团队将互相比赛一次。前 2 名获胜的球队将进入下一轮，因此前两名的球队将进行最后一场比赛。那么在该锦标赛中最少要进行多少场比赛呢？
一）40
b) 14
c) 43
d) 50
```
Answer: c) 43
```
Solution:
Total matches to be played = 4C2 = 6 matches.
So total number of matches played in the first round = 6 * 4 = 24 matches
Now top two teams from each group progress to the next round. These 8 teams are to be divided into 2 groups.
Total matches played in the second round = 6 × 2 = 12 matches
Now 4 teams progress to the next round. Total matches played in the third round = 6 * 1 = 6matches
From this round, 2 teams progress to the next round. And final will be played between them.
Total matches = 24 + 12 + 6 + 1 = 43

编程需要懂一点英语
有 12 个字母和 12 个信封。每个信封中随机插入一封信。在不正确的信封中恰好插入 1 个字母的概率是多少？
一）1
b) 0
c) 10 个！
d) 这些都不是
```
Answer: b) 0
```
Solution:
This is a question of very common sense in which,
12 letters are to be inserted in 12 envelopes, 1 in each, so if one letter is inserted into a wrong envelope there has to be another letter which is to be inserted into another wrong envelope. So the probability of this is 0.

编程需要懂一点英语
地球表面的空洞将被填充。填充的总成本为卢比。 20000。每立方米的填充成本为225卢比。3立方米的土壤需要多少次才能填充空心空间？
a) 29.62
b) 30.32
c) 88.88
d) 43.64
```
Answer: a) 29.62
```
Solution:
The total cost of filling = 20, 000
Cost of filling 1 cubic meter = Rs. 225
So cubic meters to be filled = 20, 000/225 = 88.89 meter-cube
Now we need to find the three times of 88.89 to be filled = 88.89/3 = 29.63
So the closest match is 29.62

编程需要懂一点英语
一个 7 位数字由所有不同的数字组成。如果最右边和最左边的数字分别固定为 5 和 6，请找出这样的数字可以组成多少个？
一）120
b) 30240
c) 6720
d) 这些都不是
```
Answer: c) 6720
```
Solution:
If the digits at extreme left and right are fixed as 5 and 6, then the number of digits left = 8
So the in-between 5 places can be filled in 8 * 7 * 6 * 5 * 4 ways
= 6720 ways

编程需要懂一点英语
一辆轿车有五个轮胎（四个公路轮胎和一个备用轮胎），它们在行驶 40, 000 公里的旅程中平均使用。每个轮胎的使用公里数为
a) 32000
b) 8000
c) 4000
d) 10000
```
Answer: a) 32000
```
Solution:
The total km travelled by the sedan = 40, 000 km
Since every tire capacity’s = 40, 000/5 = 8000 km each
So total distance covered by each tire = 8000*4 = 32000 km each will be travelled by each tire after being worn out after every 8000 km.

编程需要懂一点英语