连续电荷分布

电荷是物质的基本特征，它调节基本粒子如何受到电场或磁场的影响。正负电荷存在于离散的自然单元中，不能制造或破坏。有两种电荷：正电荷和负电荷。当一种电荷过多的两个项目彼此相对接近时，它们会相互排斥。当两个具有过多相反电荷的东西，一个带正电，另一个带负电，靠近时，它们会相互吸引。

电荷是许多基本或亚原子物质粒子共有的属性。例如，电子带负电荷，而质子带正电荷。另一方面，中子不带电荷。实验发现，每个电子的负电荷与每个质子的正电荷大小相同。电荷以自然单位测量，相当于电子或质子的电荷，这是一个基本的物理常数。

连续电荷分布

处理离散电荷组合涉及 q1、q2、…、qn。数学处理更容易，不需要微积分，这也是我们将自己局限于离散电荷的原因之一。然而，由于许多原因，使用离散电荷是不切实际的，我们必须改为使用连续电荷分布。在连续电荷分布中，所有电荷紧密结合在一起，它们之间的空间很小。

费用可以通过三种方式分配，包括

• 线性电荷分布。
• 表面电荷分布。
• 体积电荷分布。

线性电荷分布

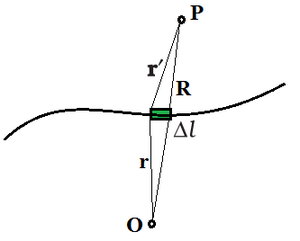
线性电荷分布

当电荷沿长度均匀分布时，例如围绕圆的圆周或沿直线，这称为线性电荷分布。线性电荷分布由符号 λ 表示。

The linear charge density λ of a wire is defined by

$\lambda=\frac{\Delta{Q}}{\Delta{l}}$

where, ∆l is on the macroscopic scale, a small line element of wire, yet it contains a significant number of microscopic charged elements and ∆Q is the charge contained in that line element. 

λ 的单位是 C/m。

表面电荷分布

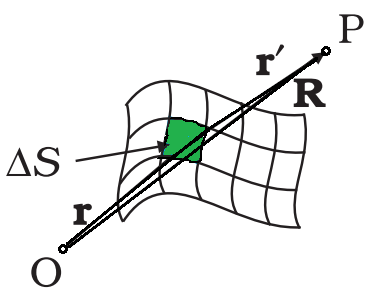
表面电荷分布

根据微小带电元素的位置来表征带电导体表面的电荷分布是不切实际的。更实际的是考虑导体表面上的面积元素 S（在宏观尺度上很小，但大到足以包含许多电子）并指定该元素上的电荷 Q。

A surface charge density σ at the area element by  

$\sigma=\frac{\Delta{Q}}{\Delta{S}}$

The surface charge density σ is a continuous function. 

所述的表面电荷密度忽略了微观水平上的电荷量化和电荷分布不连续性，这是一个区域元素 ΔS 上的微观电荷密度的平滑平均值，它在微观上很大但在宏观上很小，反映了宏观表面电荷密度。

表面电荷密度σ的单位是C/m ² 。

体积电荷分布

体积电荷分布

类似地，当电荷均匀地分布在一个体积上时，它被称为体积电荷分布 ρ，例如在球体或圆柱体内。

The volume charge density ρ (also known as charge density) is defined.

$\rho=\frac{\Delta{Q}}{\Delta{V}}$

where ∆Q denotes the charge in the macroscopically small volume element ∆V, which contains a high number of microscopic charged constituents. 

体电荷密度ρ的单位是C/m ³ 。

由于连续电荷分布而产生的场

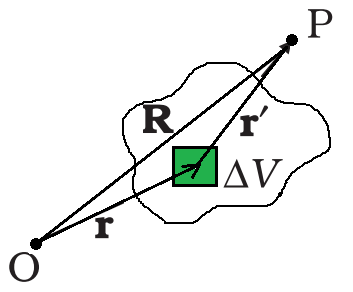
可以以与可以计算由于离散电荷系统引起的场相同的方式计算由于连续电荷分布引起的场。假设在空间中连续电荷分布存在电荷密度。取任意合适的原点O，令r为电荷分布中任意点的位置向量。电荷密度 ρ 可能因点而异，即它是 r 的函数。将电荷分布分成大小为 ΔV 的小体积元素。体积元素 ΔV 中的电荷是 ρΔV。

现在，考虑具有位置矢量 R 的分布内部或外部的任何一般点 P。由于电荷 ρΔV 产生的电场由库仑定律给出，

$\Delta{E}=\frac{1}{4\pi\epsilon_0}\frac{\rho\Delta{V}}{r'^2}\hat{r'}$

其中 r' 是电荷元素和 P 之间的距离，并且$\hat{r'}$是从电荷元素到 P 的方向上的单位向量。

由于电荷分布的总电场是使用叠加方法计算的，该方法是通过对不同体积元素引起的电场求和来计算的。

$\Delta{E}\cong\frac{1}{4\pi\epsilon_0}\sum_{all\text{ }\Delta{V} }\frac{\rho\Delta{V}}{r'^2}\hat{r'}$

示例问题

问题1：什么是线性电荷分布？

解决方案：

When charges are dispersed equally along a length, such as around the circumference of a circle or along a straight wire, this is known as linear charge distribution. The linear charge distribution is symbolized by the symbol λ. The linear charge density λ of a wire is defined by

$\lambda=\frac{\Delta{Q}}{\Delta{l}}$

where, ∆l is on the macroscopic scale, a small line element of wire, yet it contains a significant number of microscopic charged elements and ∆Q is the charge contained in that line element. The units for λ are C/m.

问题 2：电荷在空间中沿无限曲线分布，电荷分布为 λ。与直线保持一定距离的点电荷 q 所受的力是多少？

解决方案：

Let the point charge is situated at a distance r from a small part dl on the line.

The charge stored in stat small part is λ.dl.  

The force due to that small part will be directed towards the unit vector \hat{r}.  

Therefore, force on that charge due to the entire linear charge distribution can be written as

$F=q\int\lambda{r^2}\hat{r}dl$

问题 3：内半径 r 和外半径 R 的圆环具有均匀的电荷密度 a。环上的总费用是多少？

解决方案：

The total surface area of the annulus is π×(R2-r2)

It has an outer radius R and an inner radius r.

The surface charge density is the amount of charge stored on the unit surface area.

The surface charge density is a.

Therefore total charge on the annulus = π×a×(R2-r2).

问题 4：半径为 1m 的固体非导电球体带有 10 C 的总电荷，该电荷均匀分布在整个球体中。确定球体的电荷密度。

解决方案：

The volume of the sphere = (4/3)πr3.

where r is the radius of the sphere.  

Therefore, the charge density, ρ= total charge/[(4/3)πr3].

Now substituting the values,  

ρ = 10/[(4/3)πr3]

ρ= 2.38 C/m3.  

But if the sphere is conducting, we have to consider the surface charge density.

问题5：什么是表面电荷分布？

解决方案：

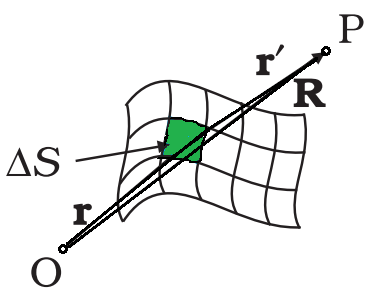
It is impractical to characterize the charge distribution on the surface of a charged conductor in terms of the positions of the tiny charged elements. It is more practical to consider an area element S on the conductor’s surface (which is small on a macroscopic scale but large enough to contain many electrons) and specify the charge Q on that element. A surface charge density σ at the area element by  

$\sigma=\frac{\Delta{Q}}{\Delta{S}}$

The surface charge density σ is a continuous function. The surface charge density as stated overlooks charge quantification and charge distribution discontinuities at the microscopic level. , which is a smoothed out average of the microscopic charge density across an area element ∆S, which is huge microscopically but small macroscopically, reflects macroscopic surface charge density. The unit of surface charge density σ is C/m².