排列与组合–定义，差异，示例

排列被称为选择，然后是来自给定序列或项目集合的特定项目集的排列。排列由以下公式表示，

ⁿP_r = (n!)/(n-r)!, n > 0 and r > 0

where,

n is considered to be the number of different elements.

r is the arrangement pattern of the element.

组合

组合是指从给定的项目序列或项目集合中选择特定项目集。在组合的情况下，单个项目的选择顺序没有任何相关性。组合由以下公式表示，

ⁿC_r = (n!)/[r! (n-r)!], n>0 and r>0

where 

n is the number of different elements

r is the arrangement pattern of the element

排列和组合之间的主要区别如下：

Permutation	Combination
Ordered arrangement of items	Unordered arrangement of items
Also known as ordered elements	Also known as an unordered set of elements
A single combination may lead to the derivation of multiple permutations.	A single permutation can lead to only a single combination.
Order is important.	Order does not hold any relevance.

为了说明排列和组合之间的区别，我们可以考虑以下示例；

假设我们有 3 个字母，A、B 和 C。

现在，

从三项中任选两项的可能组合如下：

AB  (=BC)

BC  (=CB)

CA  (=AC)

ⁿC_r = (n!)/[r! (n-r)!]

= ³C_r

$= \frac{3!}{2! * 1!} \\ \frac{3 * 2 * 1}{2 * 1} \\ = 3$

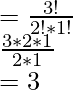
Therefore, there are three ways of choosing two items. 

现在，在排列的情况下，

AB  

BA

BC

CB

CA  

AC 

ⁿP_r = (n!)/(n-r)!

$= \frac{3!}{1!} \\ \frac{3 * 2 * 1}{1} \\ = 3 * 2 \\ = 6$

因此，有六种方法可以从总共六个项目中选择和排列两个项目。

示例问题

问题1：举一个Yash和Yashvi两个项目的排列组合的例子

解决方案：

Possible Permutations are

Yash Yashvi 

Yashvi Yash

Possible Combinations are

Yash Yashvi, since both the items have to be definitely selected

问题 2：计算从 6 个项目中选择 4 个项目的排列。

解决方案：

We know, 

ⁿP_r = (n!)/(n-r)!, n>0 and r>0

Here, 

n = 6

r = 4

Substituting the values, we get, 

ⁿP_r = (6!)/(6-4)!

= 6!/2!

= 6 * 5 * 4 * 3

= 360

问题 3：计算从 6 个项目中选择 4 个项目的组合。

解决方案：

We know, 

ⁿC_r = (n!)/[r! (n-r)!], n>0 and r>0

Here,

n = 6

r = 4

Substituting the values, we get, 

ⁿP_r = (6!)/[4! * (6-4)!]

= 6! / (4! * 2!)

= 6!/ 4! * 2

= (6 * 5 * 4 * 3 * 2 )/ (4 * 3 * 2) * 2

= 15

问题4：计算5！，如果假设，4！ = 100

解决方案：

We know, 

n! = n * (n-1)!

Substituting the given hypothetical values, we get, 

5! = 5 * 4!

= 5 * 4!

= 5 * 100

= 500