如果平行边的长度为 40 厘米和 20 厘米,并且不平行的边相等且长度为 26 厘米,则计算梯形的面积。
测量处理与各种几何形状及其测量有关的数量。它可以分为两种类型:测量 2D 和测量 3D。顾名思义,前者处理那些只有两个维度的形状:长度和宽度;而后者处理具有三个维度的形状:长度、宽度和高度。
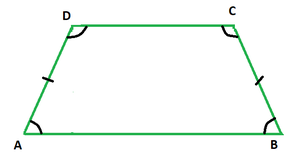
等腰梯形
等腰梯形是一个四边形,只有一对平行边,另一对不平行且长度相等。像任何其他四边形一样,它的角度之和始终为 360°。下图描绘了一个等腰梯形ABCD,其中AB∥CD和不平行边的长度相等,即AD=BC。
梯形面积
梯形的面积是其平行边之和与所述平行边之间的距离,即梯形的高度或高度的乘积的一半。
A = ½ × (a + b) × h
where,
a and b depict the lengths of parallel sides and h is the height of the trapezium.
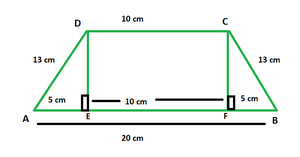
如果平行边的长度为 40 厘米和 20 厘米,并且不平行的边相等且长度为 26 厘米,则计算梯形的面积。
解决方案:
Area of a trapezium = ½ × (a + b) × h …(i)

In the figure above, DCEF is a rectangle with l = 20 cm. △ADE and △CFB are both right triangles with hypotenuse 26 cm and base 10 cm each.
In right △ADE, using Pythagoras Theorem, we have:
AD2 = AE2 + DE2
⇒ 262 − 102 = DE2
⇒ DE = 24 cm
Thus, height of trapezium = h = 24 cm, a = 20 cm and b = 40 cm.
Substituting the above values in eq. (i), we have:
A = ½ × (20 + 40) × 24
Area of the trapezium = 720 sq. cm.
类似问题
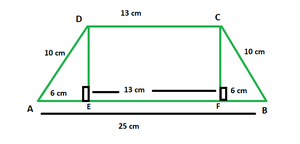
问题 1. 在梯形中,平行边的长度为 20 厘米和 10 厘米。如果梯形的非平行边相等且长度为 13 厘米,则计算梯形的面积。
解决方案:
Area of a trapezium = ½ × (a + b) × h …(i)
In the figure above, DCEF is a rectangle with l = 10 cm. △ADE and △CFB are both right triangles with hypotenuse 13 cm and base 5 cm each.
In right △ADE, using Pythagoras Theorem, we have:
AD2 = AE2 + DE2
⇒ 132 − 52 = DE2
⇒ DE = 12 cm
Thus, height of trapezium = h = 12 cm, a = 10 cm and b = 20 cm.
Substituting the above values in eq. (i), we have:
A = ½ × (10 + 20) × 12
Area of the trapezium = 180 sq. cm.
问题 2. 在梯形中,平行边的长度为 13 厘米和 25 厘米。如果梯形的非平行边相等且长度为 10 厘米,则计算梯形的面积。
解决方案:
Area of a trapezium = ½ × (a + b) × h …(i)
In the figure above, DCEF is a rectangle with l = 13 cm. △ADE and △CFB are both right triangles with hypotenuse 10 cm and base 6 cm each.
In right △ADE, using Pythagoras Theorem, we have:
AD2 = AE2 + DE2
⇒ 102 − 62 = DE2
⇒ DE = 8 cm
Thus, height of trapezium = h = 8 cm, a = 13 cm and b = 25 cm.
Substituting the above values in eq. (i), we have:
A = ½ × (13 + 25) × 8
Area of the trapezium = 152 sq. cm.
问题 3. 在梯形中,平行边的长度为 40 厘米和 70 厘米。如果梯形的非平行边相等且长度为 25 厘米,则计算梯形的面积。
解决方案:
Area of a trapezium = ½ × (a + b) × h …(i)

In the figure above, DCEF is a rectangle with l = 40 cm. △ADE and △CFB are both right triangles with hypotenuse 25 cm and base 15 cm each.
In right △ADE, using Pythagoras Theorem, we have:
AD2 = AE2 + DE2
⇒ 252 − 152 = DE2
⇒ DE = 20 cm
Thus, height of trapezium = h = 20 cm, a = 40 cm and b = 70 cm.
Substituting the above values in eq. (i), we have:
A = ½ × (40 + 70) × 20
Area of the trapezium = 1100 sq. cm.
问题 4. 在梯形中,平行边长 40 厘米和 10 厘米。如果梯形的非平行边相等且长度为 25 厘米,则计算梯形的面积。
解决方案:
Area of a trapezium = ½ × (a + b) × h …(i)

In the figure above, DCEF is a rectangle with l = 13 cm. △ADE and △CFB are both right triangles with hypotenuse 10 cm and base 6 cm each.
In right △ADE, using Pythagoras Theorem, we have:
AD2 = AE2 + DE2
⇒ 252 − 152 = DE2
⇒ DE = 20 cm
Thus, height of trapezium = h = 20 cm, a = 10 cm and b = 40 cm.
Substituting the above values in eq. (i), we have:
A = ½ × (10 + 40) × 20
Area of the trapezium = 500 sq. cm.