帕斯卡三角公式

概率是数学的支柱。它说明事件可能如何发生。它处理做出更可能的决定的数字理由。概率越高，事件发生的机会就越大，反之亦然。帕斯卡三角形是著名数学家布莱斯帕斯卡提出的一个美丽的概率概念，用于在任何二项式表达式的展开中求系数。

帕斯卡三角

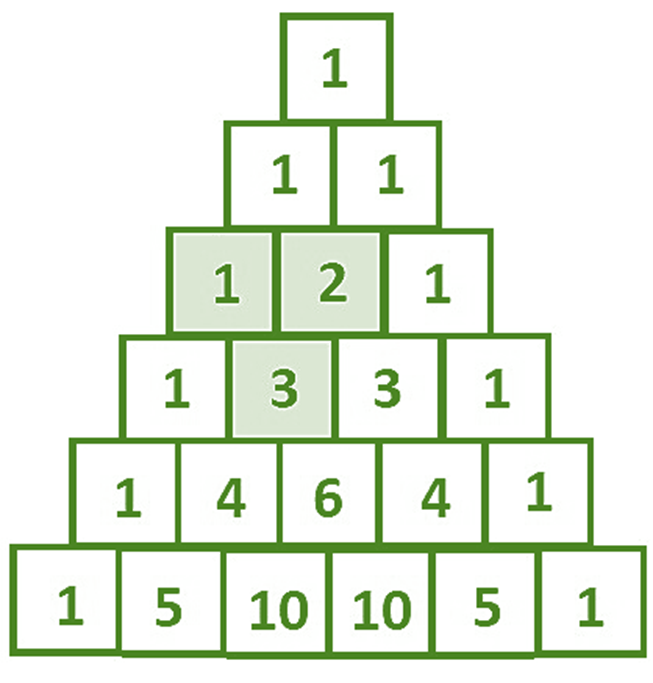
帕斯卡三角法是一种了解二项式表达式 (x + y) ⁿ项的二项式系数的方法，其中 n 可以是任何正整数，x,y 是实数。帕斯卡三角形以三角形形式表示，它是一种三角形排列形式的数字模式。它从顶部的 1 开始，在三角形的两侧向下运行 1。在帕斯卡三角形中，两个数字及以下的每个新数字，其值是上面两个数字的总和。该三角形用于不同类型的概率条件。这里每一行代表 (x + y) ⁿ的膨胀系数。

零行 n = 0, (x + y) ⁰

第一行 n = 1 , (x + y) ¹

第二行 n = 2, (x + y) ²

第三行 n = 3, (x + y) ³

第四行 n = 4, (x + y) ⁴

这里 (x + y) ⁿ的任何展开式中 y 的幂代表帕斯卡三角形的列。 n 代表帕斯卡三角形的行。行和列在帕斯卡三角中的索引为 0。

帕斯卡三角构造

制作帕斯卡三角形非常简单。从第一行（第 0 行）开始，只写数字 1。在相应的行中，帕斯卡三角形中的新正方形将是该正方形正上方并与之接触的平方和。例如，求第 4 行和第 2 列的平方和是第 3 行第 1 列和第 3 行第 2 列的平方和。所以第 4 行第 2 列的平方的值为 1 + 2 = 3。

帕斯卡三角形的性质

1. 帕斯卡三角形中的每个数字都是上面两个数字的总和。
2. 一行中的数字本质上是对称的。
3. 每个数字代表一个二项式系数。
4. 三角形左右两边的数字始终为 1。
5. 第 n 行包含 (n+1) 个数字。

帕斯卡三角公式

The pascal’s triangle formula to find the elements in the nth row and kth column of the triangle is 

$p \choose q$=  {p-1} \choose {q-1} {p-1} \choose {q-1}${p-1} \choose {q-1}$ + ${p-1} \choose {q}$

Here, 0 ≤ q ≤ p, p is a non-negative number

Or the formula to find number in the nth row and rth column is given by ^pC_q = p!/(p – q)!q!

^pC_q =^pC_q-1 + ^p-1C_q-1 

帕斯卡三角二项式展开

众所周知，帕斯卡三角定义了二项式 (x + y) ⁿ项的二项式系数，因此 (x + y) ⁿ的展开式为：

(x + y)ⁿ = a₀xⁿ + a₁x^n-1 + ……a_n-1xy^n-1 + a_nyⁿ

示例问题

问题 1：求 (x + y) ³展开式中 x ^2y项的系数。

解决方案：

Method 1:

We look at the row 3rd row of Pascal’s Triangle because n is 3 and 1st column of the Pascal’s Triangle because power of y is 1 in the term x^2y. So the coefficient is 3.

Method 2:

We simply apply ⁿC_r where n = 3, r = 1.

So coefficient of x^2y in the expansion of (x + y)³ is ³C₁ = 3

问题 2：求 (4x + 3y) ⁴展开式中 x ² y ²项的系数。

解决方案：

Method 1:

We look at the row 4th row of Pascal’s Triangle because n is 4 and 2nd column of the Pascal’s Triangle because power of y is 2 in the term x²y². So number in Pascal’s Triangle is 6. 

But we see that coefficient of x is 4 and y is 3 now since power of x is 2 and y is 2 in the term x²y² so pascal Triangle number will be multiplied by 4² and 3² to find the coefficient.

Coefficient = 6 x 4² x 3² = 864

Method 2:

We simply apply ⁿC_r where n = 4, r = 2.

So Pascal Triangle number of term x²y² in the expansion of (4x +3y)⁴ is ⁴C₂ = 6.

But we see that coefficient of x is 4 and y is 3 now since power of x is 2 and y is 2 in the term x²y² so pascal Triangle number will be multiplied by 4² and 3² to find the coefficient.

Coefficient = 6 x 4² x 3² = 864

问题 3：写出帕斯卡三角形的第 6 行

解决方案：

6th row can be written as :  6C0 6C1 6C2 6C3 6C4 6C5 6C6

                                               1, 6, 15, 20, 15, 6, 1

问题 4：求 (2x + y) ⁴的展开式中 x ⁴项的系数。

解决方案：

Method 1:

We look at the row 4th row of Pascal’s Triangle because n is 4 and 0th column of the Pascal’s Triangle because power of y is 0 in the term x⁴. So number in Pascal’s Triangle is 1.

But we see that coefficient of x is 2 and y is 0 now since power of x is 4 and y is 0 in the term x⁴ so Pascal Triangle’s number will be multiplied by 2⁴ and 1⁰ to find the coefficient.

Coefficient = 1 x 2⁴ x 1⁰= 16 

Method 2:

We simply apply ⁿC_r where n = 4, r = 0.

So Pascal Triangle number of term x⁴ in the expansion of (2x + y)⁴ is ⁴C₀ = 1.

But we see that coefficient of x is 2 and y is 0 now since power of x is 4 and y is 0 in the term x⁴ so Pascal Triangle’s number will be multiplied by 2⁴ and 1⁰ to find the coefficient.

Coefficient = 1

问题 5：求 (2x + y) ³展开式中 xy ²项的系数。

解决方案：

Method 1:

We look at the row 3rd row of Pascal’s Triangle because n is 3 and 2nd column of the Pascal’s Triangle because power of y is 2 in the term xy². So number in Pascal’s Triangle is 3.

But we see that coefficient of x is 2 and y is 1 now since power of x is 2 and y is 1 in the term xy² so Pascal Triangle’s number will be multiplied by 2¹ and 1² to find the coefficient.

Coefficient = 3 x 2¹ x 1² = 6  

Method 2:

We simply apply ⁿC_r where n = 3, r = 2.

So Pascal Triangle number of term xy² in the expansion of (2x + y)³ is ³C₂ = 3.

But we see that coefficient of x is 2 and y is 1 now since power of x is 2 and y is 1 in the term xy^2 so Pascal Triangle’s number will be multiplied by 2¹ and 1² to find the coefficient.

Coefficient = 3 x 2¹ x 1² = 6