📌  相关文章
📜  高斯定律的应用

📅  最后修改于: 2022-05-13 01:57:37.868000             🧑  作者: Mango

高斯定律的应用

根据高斯定律，封闭表面的总电通量等于所包含的电荷除以介电常数。一个区域中的电通量定义为电场乘以在垂直于该场的平面上投影的表面积。

现在我们已经确定了高斯定律是什么，让我们看看它是如何使用的。在您看来，这已经是一项具有挑战性的任务了吗？现在会容易很多。值得注意的是，高斯定律可用于解决具有独特对称性的复杂静电问题，例如平面、球面或柱面对称。因此，与普遍的看法相反，将高斯定律应用于您的工作实际上可能会使它变得更简单！

高斯定律的应用

我们现在来看看高斯定律的一些应用。首先，我们知道在某些情况下，计算电场相当困难，需要大量积分。高斯定律用于使计算电场更容易。

在了解更多有关应用程序之前，让我们先看看如何应用立法。我们需要选择一个使评估电场变得简单的高斯表面。为了使事情更容易，人们应该使用对称性。同样重要的是要认识到高斯表面不必与真实表面相匹配。它可能在高斯表面的内部或外部。

无限线产生的电场

考虑一条无限长且具有线性电荷密度 λ 的导线。为了计算电场，我们使用圆柱高斯表面。由于电场 E 是径向的，因此通过表面末端的通量将为 0。

因为电场和面积矢量相互垂直，所以情况就是这样。我们可能会争辩说，电场的大小将是恒定的，因为它垂直于曲面上的每个点。

无限线产生的电场

曲面圆柱面的表面积为 2 π r l。流过曲线的电通量等于 E × (2 π rl)。

根据高斯定律：

φ = q ⁄ ε 0

E × (2 π rl) = λ l ⁄ ε 0

E = λ ⁄ 2 π ε 0 r

无限平面片产生的电场

考虑具有横截面积 A 和表面电荷密度 σ 的无限平面薄片。无限平面片在以下位置：

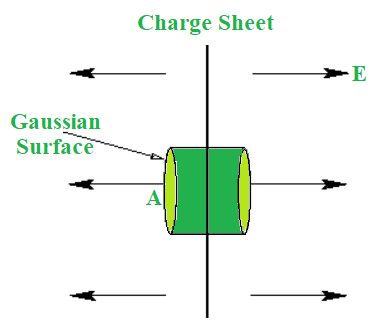
无限平面片产生的电场

无限电荷片产生的电场垂直于片的平面。考虑一个圆柱高斯曲面，其轴垂直于片材平面。高斯定律允许我们计算电场 E 如下：

φ = q ⁄ ε 0

电荷 q 将是电荷密度 (σ) 乘以连续电荷分布中的面积 (A)。在讨论净电通量时，我们将只考虑来自想象的高斯表面两端的电流。我们可以解释为曲面面积和电场相互垂直，导致电通量为零。因此，净电通量：

φ = EA - (- EA)

φ = 2 EA

所以，

2 EA = σ A ⁄ ε 0

E = σ ⁄ 2 ε0

薄球壳产生的电场

考虑一个半径为“R”且表面电荷密度为 σ 的薄球壳。壳具有球对称性。球壳产生的电场可以通过两种方式计算：

  • 球壳外
  • 球壳内部

让我们仔细看看这两种情况。

球壳外的电场

取球壳外距球壳中心 r 处的点 P 得到电场。我们使用具有半径 r 和中心 O 的高斯球面来实现对称性。因为所有点与球体中心的间距“r”相等，所以高斯表面将通过 P 并在周围经历一个恒定的电场 E。因此，因此，总电通量：

φ = q ⁄ ε 0 = E × 4 π r 2

包含在表面内的电荷，q = σ × 4 π R 2 。因此，

E × 4 π r 2 = σ × 4 π R 2 ⁄ ε 0

E = σ R 2 ⁄ ε 0 r 2

电场也可以用电荷的形式写成：

E = kq ⁄ r 2

球壳内的电场

让我们看一下球壳内的一点 P，看看那里的电场如何。我们可以使用对称性来创建一个通过 P、以 O 为中心、半径为 r 的高斯球面。现在，根据高斯定律，

φ = q ⁄ ε 0 = E × 4 π r 2

由于表面电荷密度分布在表面之外，因此壳内不包含电荷。因此，上式得出的电场也为零，即

E = 0，因为 q = 0。

示例问题

问题 1：半径为 r 的半球形碗被放置在具有均匀电场 E 的空间区域中。找出通过碗的电通量。

解决方案：

问题 2：如果包含电荷的高斯表面的半径减半，通过高斯表面的电流如何变化？

解决方案：

问题 3：一个半径为 r、长度为 l 的圆柱表面，包围着一根细的、无限长的、具有电荷密度的导线，其轴与表面重合。找出通过圆柱体表面的电通量的公式。

解决方案：

问题 4：为什么高斯定律不能应用于无界曲面？

解决方案：

问题5：2×10 -8 C的电荷均匀分布在半径为2cm的球体表面。它被一个半径为 5 厘米的同心空心导电球体覆盖。求离中心 3 cm 处的电场。

解决方案：