点梯度公式

笛卡尔平面中的一条直线经过无数个点。这些点中的每一个都有自己的 x 和 y 坐标。一条线通过的点用于找到它的斜率。不仅如此，这些点还可以用来写直线方程。下面讨论一种这样的方法。

点梯度公式

在许多以笛卡尔形式编写/查找/表达直线方程的方法中，点斜率或点梯度公式在坐标几何中占有非常重要的地位。顾名思义，这种形式的方程由直线经过的一个点及其斜率组成。

公式

点梯度公式如下：

y – y₁ = m(x – x₁)

Where,

• x and y depict general point coordinates.
• x₁ and y₁ are the numerical coordinates of a point through which the line passes.
• m represents the slope of the given line.

点梯度公式的推导

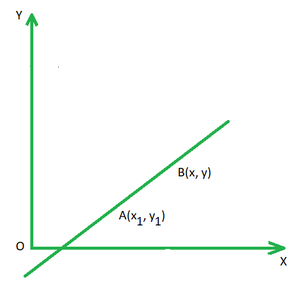
Slope of a line passing through two points (x, y) and (x₁, y₁) = m = $\frac{y-y_1}{x-x_1}$

Multiplying both sides by (x – x₁), we have:

$m(x - x_1) = (x - x_1)[\frac{y-y_1}{x-x_1}]$

⇒ y – y₁ = m(x – x₁)

Hence proved.

示例问题

问题 1：通过 (2, -4) 且斜率为 5 的直线的方程是什么？

解决方案：

The given point is (2, −4). Thus, x₁ = 2, y₁ = −4.

Also, m = slope = 5. 

We know the point slope equation of a line is given by y – y₁ = m(x – x₁).

Substituting the above values in the equation, we have:

y – (-4) = 5(x – 2)

⇒ y + 4 = 5x − 10

⇒ y = 5x − 10 − 4

⇒ y = 5x − 14

问题 2：通过 (5, 2) 且斜率为 3/4 的直线的方程是什么？

解决方案：

The given point is (5, 2). Thus, x₁ = 5, y₁ = 2.

Also, m = slope = 3/4.

We know the point slope equation of a line is given by y – y₁ = m(x – x₁).

Substituting the above values in the equation, we have:

y − (2) = 3/4(x − 5)

⇒ y − 2 = 3x/4 − 15/4

⇒ y = 3x/4 − 15/4 + 2

⇒ y = 3x/4 − 7/4

问题 3：通过 (3, 3) 的水平线的方程是什么？

解决方案：

The given point is (3, 3). Thus, x₁ = 3, y₁ = −3.

Since the slope of a horizontal line is zero, m = 0.

We know the point slope equation of a line is given by y – y₁ = m(x – x₁).

Substituting the above values in the equation, we have:

y − (3) = 0(x − 3)

⇒ y – 3 = 0

⇒ y = 3

问题4：假设一条直线经过点(1, 1) 和(-2, 4)。使用点斜率公式找到它的方程。

解决方案：

We know the point slope equation of a line is given by y – y₁ = m(x – x₁).

In order to use the point- slope form, we need to calculate the slope of the line first.

Slope = m = $\frac{y_2-y_1}{x_2-x_1}\\=\frac{-2-1}{4-1}\\=\frac{-3}{3}$

⇒ m = -1

Substituting the above values in the equation, we have:

y – (1) = -1(x – 1)

⇒ y − 1 = -x + 1

⇒ y = -x + 2

问题 5：通过 (0, 3) 且斜率为 8 的直线的方程是什么？

解决方案：

The given point is (0, 3). Thus, x₁ = 0, y₁ = 3.

Also, m = slope = 8.

We know the point slope equation of a line is given by y – y₁ = m(x – x₁).

Substituting the above values in the equation, we have:

y − 3 = 8(x – 0)

⇒ y − 3 = 8x

⇒ y = 8x + 3