婴儿床半角公式

三角学是数学的一个分支，它使用三角比来确定三角形的角度和不完整边。正弦、余弦、正切、余切、正割和余割等三角比用于研究这一数学分支。这是对直角三角形的边和角如何相关的研究。三角学由“Trigonon”和“Metron”这两个词组成，它们分别代表一个三角形和一个测量值。通过应用基于此连接的方程和恒等式，它有助于估计直角三角形的未知尺寸。

余切三角比

直角三角形任意两条边的长度之比称为三角比。在三角学中，这些比率将直角三角形的边的比率与角度联系起来。余切比表示为角的相邻边的长度除以对边的长度的比值。它由符号 cot 表示。

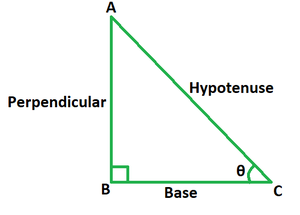
如果 θ 是直角三角形底边和斜边之间的夹角，那么，

cot θ = Base/Perpendicular = cos θ/ sin θ

Here, base is the side adjacent to the angle and perpendicular is the side opposite to it.

Cot 半角 (Cot θ /2) 公式

在三角学中，半角公式通常表示为 θ/2，其中 θ 是角度。半角方程用于确定标准角的三角比的精确值，例如 30°、45°和 60°。通过使用这些普通角度的比率值，我们可以得到复杂角度的比率值，例如 22.5°（45° 的一半）或 15°（30° 的一半）。余切半角由缩写 cot θ/2 表示。它是一个函数，返回半角的 cot函数值。函数cot θ 的周期是 π，但 cot θ/2 的周期是 2π。

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

推导

The formula for cotangent half angle is derived by using the half angle formula for sine and cosine.

We know, sin θ/2 = ±√((1 – cos θ) / 2).

Find cos θ/2 using the identity sin² θ + cos² θ = 1.

cos θ/2 = √(1 – (√((1 – cos θ) / 2))²)

cos θ/2 = √(1 – ((1 – cos θ)/ 2))

cos θ/2 = √((2 – 1 + cos θ)/ 2)

cos θ/2 = √((1 + cos θ)/ 2)

Also, we know cot θ/2 = cos (θ/2)/ sin (θ/2).

So, we get

cot θ/2 = √((1 + cos θ)/ 2)/ √((1 – cos θ)/ 2)

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

This derives the formula for cotangent half angle ratio.

示例问题

问题 1. 如果 cos θ = 3/5，使用半角公式求 cot θ/2 的值。

解决方案：

We have, cos θ = 3/5.

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (3/5))/ (1 – (3/5)))

= √((8/5)/ (2/5))

= √4

= 2

问题 2. 如果 cos θ = 12/13，使用半角公式求 cot θ/2 的值。

解决方案：

We have, cos θ = 12/13.

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (12/13))/ (1 – (12/13)))

= √((25/13)/ (1/13))

= √25

= 5

问题 3. 如果 sin θ = 8/17，使用半角公式求 cot θ/2 的值。

解决方案：

We have, sin θ = 8/17.

Find the value of cos θ using the formula sin² θ + cos² θ = 1.

cos θ = √(1 – (64/289))

= √(225/289)

= 15/17

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (15/17))/ (1 – (15/17)))

= √((32/17)/ (2/17))

= √16

= 4

问题 4. 如果 sec θ = 5/4，使用半角公式求 cot θ/2 的值。

解决方案：

We have, sec θ = 5/4.

Using cos θ = 1/sec θ, we get cos θ = 4/5.

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (4/5))/ (1 – (4/5)))

= √((9/5)/ (1/5))

= √9

= 3

问题 5. 如果 tan θ = 12/5，使用半角公式求 cot θ/2 的值。

解决方案：

We have, tan θ = 12/5.

Clearly, cos θ = 5/√(12² + 5²) = 5/13

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (5/13))/ (1 – (5/13)))

= √((18/13)/ (8/5))

= √(18/8)

= √(9/4)

= 3/2

问题 6. 如果 cot θ = 8/15，使用半角公式求 cot θ/2 的值。

解决方案：

We have, cot θ = 8/15.

Clearly, cos θ = 8/√(8² + 15²) = 8/17

Using the formula we get,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + (8/17))/ (1 – (8/17)))

= √((25/17)/ (9/17))

= √(25/9)

= 5/3

问题 7. 使用半角公式求 cot 15° 的值。

解决方案：

We have to find the value of cot 15°.

Let us take θ/2 = 15°

=> θ = 30°

Using the half angle formula we have,

cot θ/2 = √((1 + cos θ)/(1 – cos θ))

= √((1 + cos 30°)/ (1 – cos 30°))

= √((1 + (√3/2))/ (1 – (√3/2)))

= √((2 + √3)/ (2 – √3))

= √(((2 + √3) (2 + √3))/ ((2 – √3) (2 + √3)))

= √((4 + 3 + 4√3)/ (4 – 3))

= √(7 + 4√3)